Two idential charged spheres suspended from a common point by two massless strings of lengths l, are intially at a distance d(d<<1) a part because of their mutual repulsion. The charges begin to leak from both the spheresapproach each other with a velocity v.Then v varies as a function of the distance x between the spheres as:Solution in Tamil
ναx−1
ναx1/2
να x
ναx−(1/2)
Step by step video & image solution for Two idential charged spheres suspended from a common point by two massless strings of lengths l, are intially at a distance d(d<<1) a part because of their mutual repulsion. The charges begin to leak from both the spheresapproach each other with a velocity v.Then v varies as a function of the distance x between the spheres as: by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
What is the value of inductance L for which the current is a maximum in a series LCR circuit with C=10μF and w=1000s−1
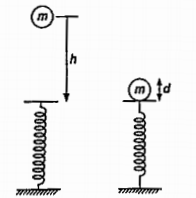
View SolutionA vertical spring with force constant K is fixed on a table. A ball of mass m at a height H above the free upper end of the spring falls vertically on the spring so that the spring is compressed by a dsitance d. The net work done in the process is:
View SolutionTwo identical charged spheres suspended from a common point by two mass-less strings of length l are initially at a distance d ( d<<l) apart because of their mutual repulsion . The charge begins to leak from both the spheres at a constant rate. As a result the charge approach each other with a velocity v. Then as a function of distance x between them .
View SolutionTwo identical charged spheres suspended from a common point by two massless strings of length l are initially a distance d ( d ltlt l) apart because of their mutual repulsion . The charge begins to leak from both the spheres at a constant rate As a result the charge approach each other with a velocity v. Then as a function of distance x between them .
View Solutionकिसी उभयनिष्ठ बिंदु से, लम्बाई l की दो द्रव्यमानहीन डोरियों से निलंबित, दो सर्वसम आवेशित गोले, अन्योन्या प्रतिकर्षण के कारण, आरम्भ में एक-दूसरे से d(d<<l) दूरी पर है। दोनों ही गोलों से एक नियत दर से आवेशों का क्षरण आरम्भ होता हैऔर इसके परिणामस्वरूप गोले एक-दूसरे की ओर वेग v से आते है तब गोलों की बीच की दूरी x के फलन के रूप में वेग से v विचरण किस रूप में होता है?
View SolutionTwo identical charged spheres suspended from a common point by two masses strings of length l are initially at a distance d (d lt lt 1) apart because of their mutual repulsion. The charge begins to leak from both the spheres at a constant rate. As a result the charge approaches each other with a velocity v. Then as a function of distance x between them
View SolutionTwo identical charged spheres suspended form a common point by two massless strings of lengths l, are initially at a distance d(d gt gt l) apart because of their mutual repulsion. The charges brgin to leak from both the spheres at a constant rate. As a result, the spheres approach each other with a velocity v. Then v varies as a function of the distance x between the spheres, as
View SolutionTwo identical charged spheres suspended from a common point by two massless strings of lengths I, are initially at a distance d(d<<l) apart because of their mutual repulsion. The charges begin to leak from both the spheres at a constant rate. As a result, the spheres approach each other with a velocity v. Then v varies as a function of the distance x between as:
View SolutionTwo identical charged spheres suspended from a common point by two massless strings of length I are initially a distance dd<<1) apart because of their mutual repulsion. The charge begins to leak from both the spheres at a constant rate. As a result the charges approach each other with a velocity v. Then as a function of distance x between them,
View SolutionTwo identical charged spheres suspended from a common point by two massless strings of length I are initially a distance d(d<<1) apart because of their mutual repulsion. The charge begins to leak from both the spheres at a constant rate. As a result the charges approach each other with a velocity v. Then as a function of distance x between them,
View SolutionTwo identical charged spheres suspended from a common point by two mass-less strings of length l are initially at a distance d ( d<<l) apart because of their mutual repulsion . The charge begins to leak from both the spheres at a constant rate. As a result the charge approach each other with a velocity v. Then as a function of distance x between them .
View SolutionTwo identical charged spheres suspended from a common point by two massless strings of length I are initially a distance x (x<<l) apart because of their mutual repulsion. The charge begins to leak from both the spheres at a constant rate. As a result the charges approach each other with a velocity v. Then as a function of distance x between them,
View SolutionTwo identical charged spheres suspended from a common point by two massless strings of lengths /, are initially at a distance d(d<<I) apart because of their mutual repulsion. The charges begin to leak from both the spheres at a constant rate. As a result, the spheres approach each other with a velocity v. Then v varies as a function of the distance x between the spheres, as :
View SolutionTwo identical charged spheres suspended from a common point by two mass-less strings of length l are initially at a distance d ( d<<l) apart because of their mutual repulsion . The charge begins to leak from both the spheres at a constant rate. As a result the charge approach each other with a velocity v. Then as a function of distance x between them .
View Solutionदो एकसमान आवेशित गोले, d लम्बाइयों की दो द्रव्यमानहीन रस्सियों द्वारा एक उभयनिष्ठ बिन्दु से लटके हुए है उनके अन्योन्य प्रतिकर्षण के कारण प्रारम्भ में उनके बीच की दूरी d (d<<l) है दोनों गोलों से एक नियत दर पर आवेशों का रिसना शुरू होता है परिणामस्वरूप, गोले एक वेग v के साथ एक - दूसरे की ओर आते है तब v गोलों के मध्य दूरी x के फलन के साथ परिवर्तित होती है निम्न में से सही विकल्प है
View Solution
