A bicycle moves on a horizontal road with some acceleration. The forces of friction between the road and the front and rear wheels are F1 and F2 respectively.
Both F1andF2 act in the forward direction
Both F1andF2 act in the backward direction
F1 acts in the forward direction F2 acts in the backward direction
F1 acts in the backward direction F2 acts in the forward direction.
The correct Answer is:D
When pedalling the bicycle the force of friction F2 on the rear wheel of the bicycle is in forward direction and on the front wheel F1 is in backward direction.
Topper's Solved these Questions
- FORCE AND LAWS OF MOTIONBOOK - MTG IIT JEE FOUNDATIONCHAPTER - FORCE AND LAWS OF MOTIONEXERCISE - EXERCISE (INTEGER VALUE TYPE )
5 Videos
- FOOTSTEPS TOWARDS NEETBOOK - MTG IIT JEE FOUNDATIONCHAPTER - FOOTSTEPS TOWARDS NEETEXERCISE - Multiple Choice Question
45 Videos
- GRAVITATION BOOK - MTG IIT JEE FOUNDATIONCHAPTER - GRAVITATION EXERCISE - Olympiad/HOTS Corner
20 Videos
Similar Questions
A 60kg man is running on horizontal road with acceleration 2ms−1 friction coefficient between foot of the man and the ground is μ=0.4 then
View SolutionAn automobile is moving on a horizontal road with a speed υ If the coefficient of friction between the tyres and the road is μ show that the shortest distance in which the automobile can be stooped is υ2/2μg.
View Solution
Knowledge Check
A bicycle moves on a horizontal road with some acceleration. The forces of friction between the road and the front and rear wheels are F1 and F2 respectively.
ABoth F1andF2 act in the forward directionBBoth F1andF2 act in the backward directionCF1 acts in the forward direction F2 acts in the backward directionDF1 acts in the backward direction F2 acts in the forward direction.A car accelerates on a horizontal road due to the force exerted by
Athe engine of the carBthe driver of the carCthe earthDthe roadA body moves on a horizontal circular road of radius of r, with a tangential acceleration aT. Coefficient of friction between the body and road surface is μ. It begin to slip when it's speed is v, then :
Av2=μrgBug=v2r+aTCμ2g2=v4r2+a2TDThe force of friction makes an angle tan−1(v2aT×r) with direction of motion at point of slipping.
A car starts rest, on a horizontal circular road of radius R, the tangential acceleration of the car is a. The friction coefficient between the road and the tyre is μ Find the speed at which car will skid and also find the distance after travelling it skids.
View SolutionA vehicle of mass m is moving on a rough horizontal road with momentum p. If the coefficient of friction between the tyres and the road be μ, then the stopping distance is
View SolutionA vehicle of mass m is moving on a rough horizontal road with momentum P . If the coefficient of friction between the tyres and the road br mu, then the stopping distance is:
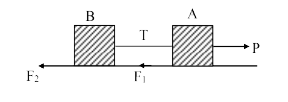
View SolutionTwo blocks A and B of the same mass are joined by a light string and placed on a horizontal surface. An external horizontal force P acts on A. The tension in the string is T. The forces of static friction acting on A and B are F1 and F2 respectively. The limiting value of F1 and F2 is F0. As P is gradually increased.
View SolutionA vehicle of mass M is moving on a rough horizontal road with a momentum P If the coefficient of friction between the tyres and the road is μ is then the stopping distance is .
View Solution