Deduce Coulomb's law from Gauss's theorem.

Text Solution

Verified by Experts

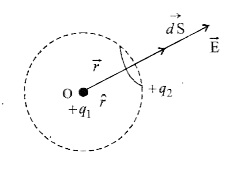
Consider two points charges $q_{1} and q_{2}$ separated by a distance r. Draw a sphere of radius r with O as the centre where O is the position of charge $q_{1}$ .
Now consider a small area element of area ds over the sphere The angle between $- \to d S$ and electric field $\to E$ is zero.
$i . e . θ = 0$
According to Gauss.s.s Theorem
$\oint \to E . - \to d S = \frac{q}{ɛ_{0}}$
$\oint E d S cos θ = \frac{q}{ɛ_{0}}$
$\oint S E d S {cos 0}^{\circ} = \frac{q_{1}}{ɛ_{0}}$
$\oint S E d S = \frac{q_{1}}{ɛ_{0}}$
Since $ɛ E$ is constant on all points of Gaussian.s surface.
$∴ ⎛ ⎜ ⎝ E \oint S d S = \frac{q_{1}}{ɛ_{0}} ⎞ ⎟ ⎠$
But $\oint S d S = 4 π r^{2}$
$∴ E \times 4 π r^{2} = \frac{q_{1}}{ɛ_{0}} \Rightarrow E = \frac{1}{4 π ɛ_{0}} \frac{q_{1}}{r^{2}}$
This is the value of electric field intensity at the position of $q_{2}$ .
Thus the force experienced by $q_{2}$ is given be
$F = q_{0} E$
$= q_{0} \times \frac{1}{4 π ɛ_{0}} \frac{q_{1}}{r^{2}}$
$∴ F = \frac{1}{4 π ɛ_{0}} \frac{q_{1} q_{2}}{r^{2}}$
This is the mathematical form of Coulomb.s Law.

|

Updated on:21/07/2023

Class 12 PHYSICS ELECTRIC CHARGES AND FIELDS

Similar Questions

Knowledge Check

Question 1 - Select One
Gauss's theorem is
A $\oint \to B \cdot d \to s = 0$
B $\oint \to B \cdot d \to s = \frac{Q}{ε_{0}}$
C $\oint \to B \cdot d \to s = μ_{0} i$
D $\oint \to B \cdot d \to s = \frac{μ_{0}}{i}$

Recommended Questions

Home
Ask Doubt
Profile