Derive a expression for electric field intensity due to an infinitely long straight uniformly charged wire or line.

Text Solution

Verified by Experts

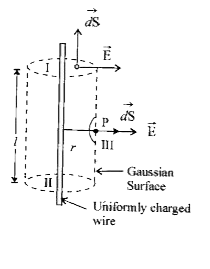
Consider an infinite and very thin straight uniformly charged wire.
Let $λ =$ be the linear charge density
$i . e ., λ = \frac{q}{l}$
$P = b e$ a point, where we want to find electric field intensity (E).
Draw an imaginary cylinder of radius r and Hength Iaroundthe charged line.
The charge enclosed by Gaussian surface, $q = λ l$
According to Gauss.s Theorem
$\int S \to E . - \to d S = \frac{q}{ɛ_{0}}$
$\int S \to E . - \to d S = \frac{λ l}{ɛ_{0}} [∴ λ = \frac{q}{l}] ... (1)$
The Gaussian surface is divided into three parts I, II and III.
$∴$ the equation (1) can be written as
$\oint S \to E . - \to d S = \oint I \to E . - \to d S + \oint I I \to E . - \to d S + \oint I I I \to E . - \to d S = \frac{λ l}{ɛ_{0}} ... (2)$
For surface I and II angle between $\to E$ and $\to d S$ is $90^{\circ}$ , and for surface III, angle betwe $\to E$ and $\to d s$ is $0^{\circ}$ .
From (2),
$∴ \oint I E d S {cos 90}^{\circ} + \oint E d S {cos 90}^{\circ} + \oint E d S {cos 0}^{\circ} = \frac{λ l}{ɛ_{0}}$
$0 + 0 + \oint I I I E d S (1) = \frac{λ l}{ɛ_{0}}$
$\oint I I I E d S = \frac{λ l}{ɛ_{0}}$
$\int I I I E d S = \frac{λ l}{ɛ_{0}}$
Since E is constant at every point on Gaussian surface
$∴ E \int I I I d S = \frac{λ}{ɛ_{0}}$
$⎛ ⎜ ⎝ \int I I I d S = 2 π r l = area of curved surface of culinder ⎞ ⎟ ⎠$
$∴ E \times 2 π r l = \frac{λ l}{ɛ_{0}}$
$∴ E = \frac{λ}{ɛ_{0} \times 2 π r l}$
$E = \frac{λ}{2 π ɛ_{0} r}$
Thus $E \propto \frac{1}{r}$
The vector form
$\to E = \frac{λ}{2 π ɛ_{0} r} ˆ n$
where $ˆ n$ is a unit vector perpendicular to curved surface of the wire.

|

Updated on:21/07/2023

Class 12 PHYSICS ELECTRIC CHARGES AND FIELDS

Similar Questions

Recommended Questions

Home
Ask Doubt
Profile