The voltage time (V−t) graph for triangular wave having peak value V0 is as shown in Fig.

The rms value of V in time interval from t=0 to T/4 is
V0√3
V02
V0√2
None of these
The correct Answer is:A
V=V0T/4t,V=4V0Tt
Vrms=√<V2>=4V0T√<t2>=4V0T
⎷∫t/40t2dt∫T/40dt=V0√3
Similar Questions
The voltage time (V-t) graph for triangular wave having peak value V0 is as shown in figure.The rms value of V in time interval from t=0 to T/4 is
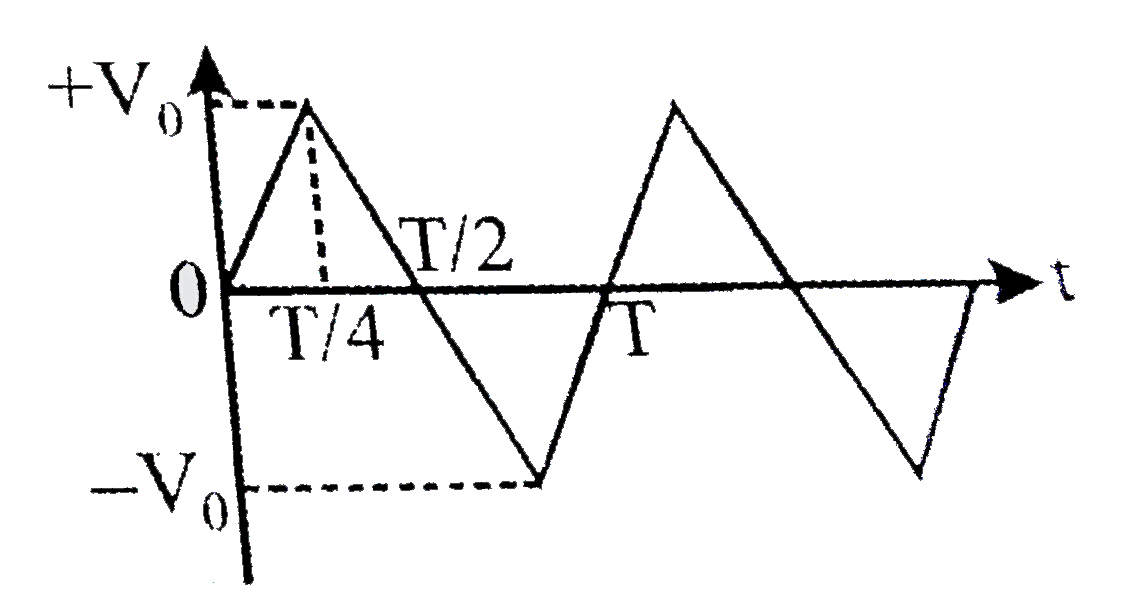 View Solution
View SolutionThe voltage time (V-t) graoh for triangular wave having peak value (V0) is as shown in fig.The rms value of V in time interval from t=0 to T/4 is:
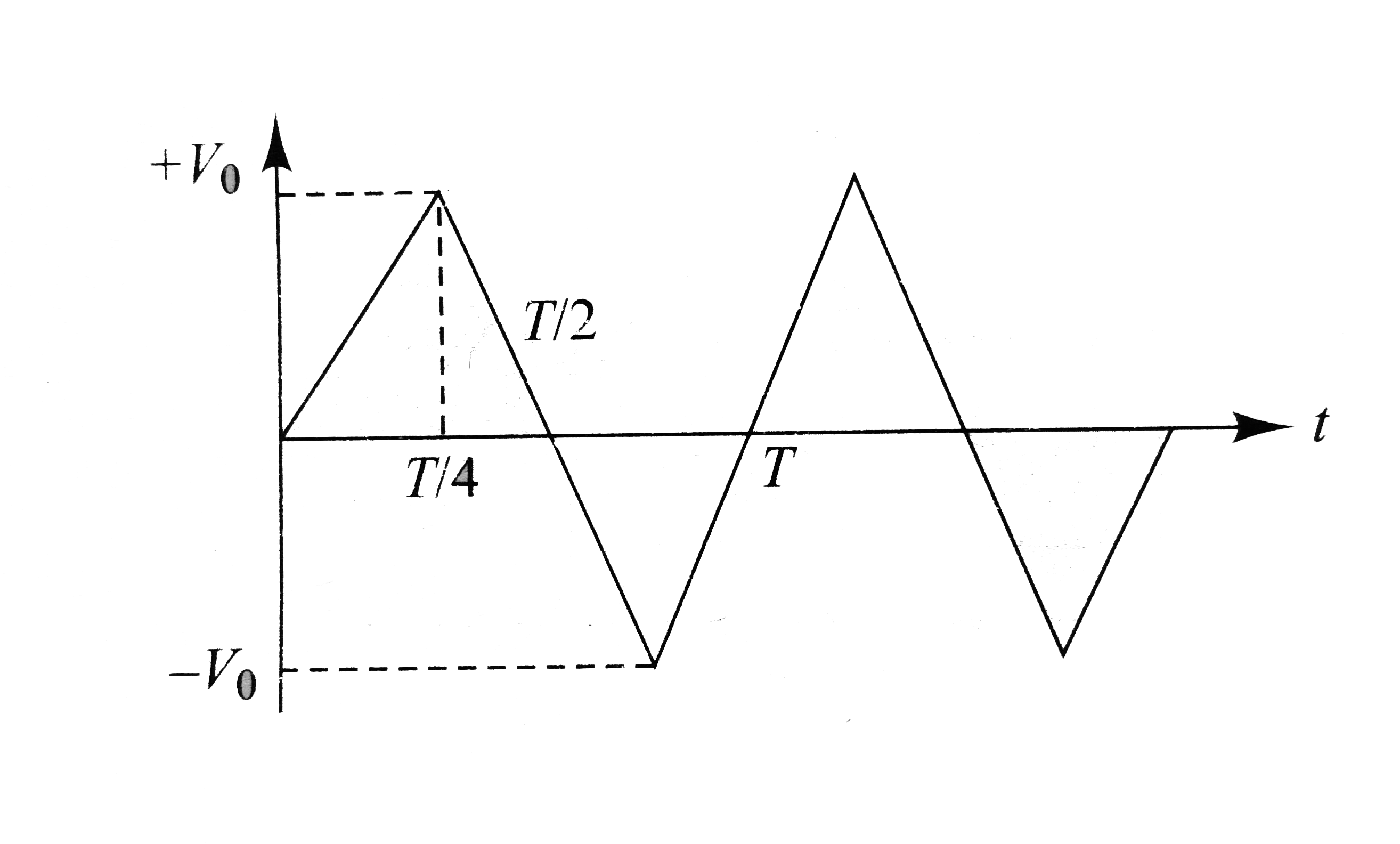 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
The voltage time (V-t) graoh for triangular wave having peak value (V0) is as shown in fig.
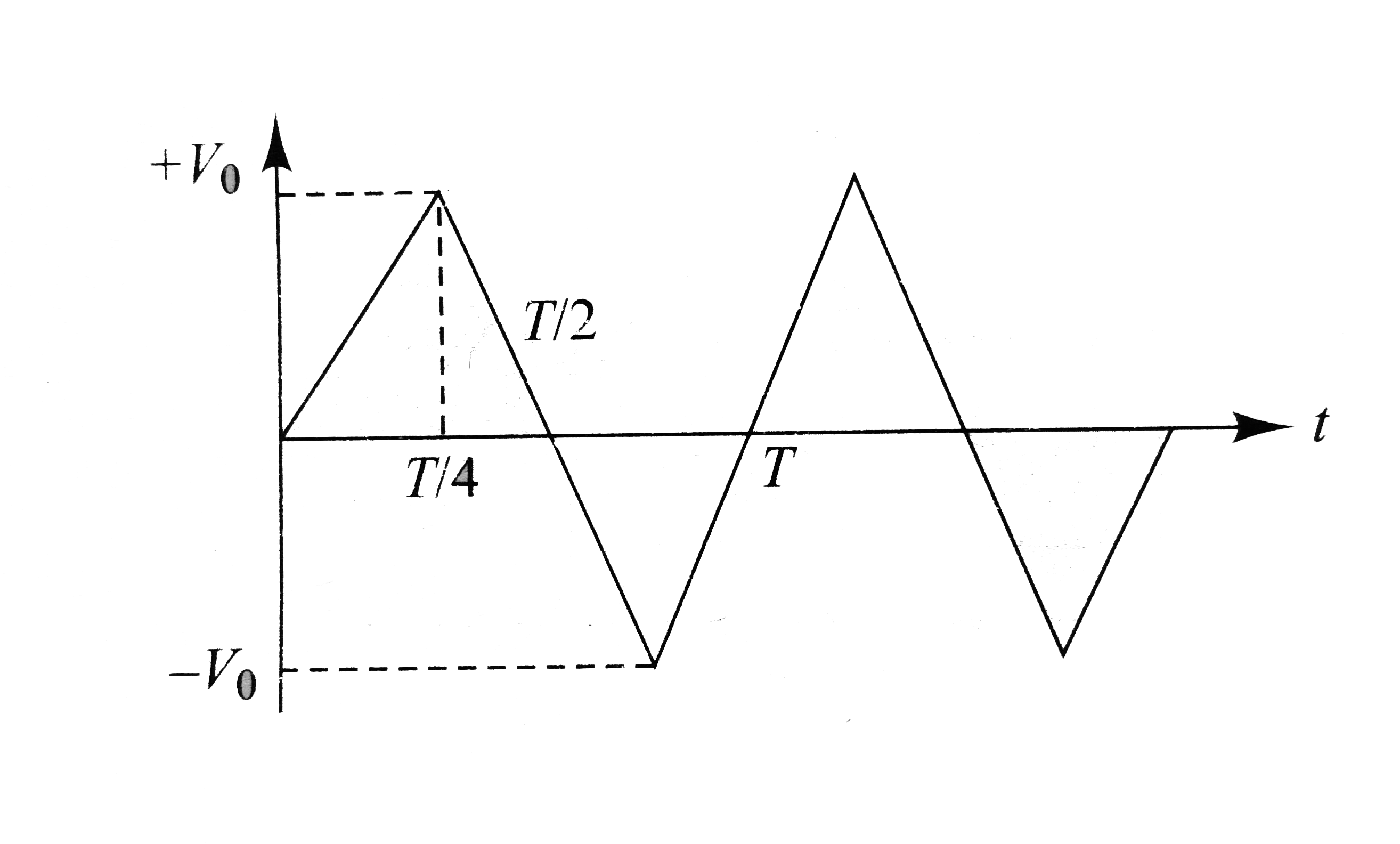 AV0√3BV02CV0√2Dnone of these.
AV0√3BV02CV0√2Dnone of these. - Question 2 - Select One
The value ofvx at times t is
Av0+atB(v0)x+aytC(v0)x+axtDNone of the above - Question 3 - Select One
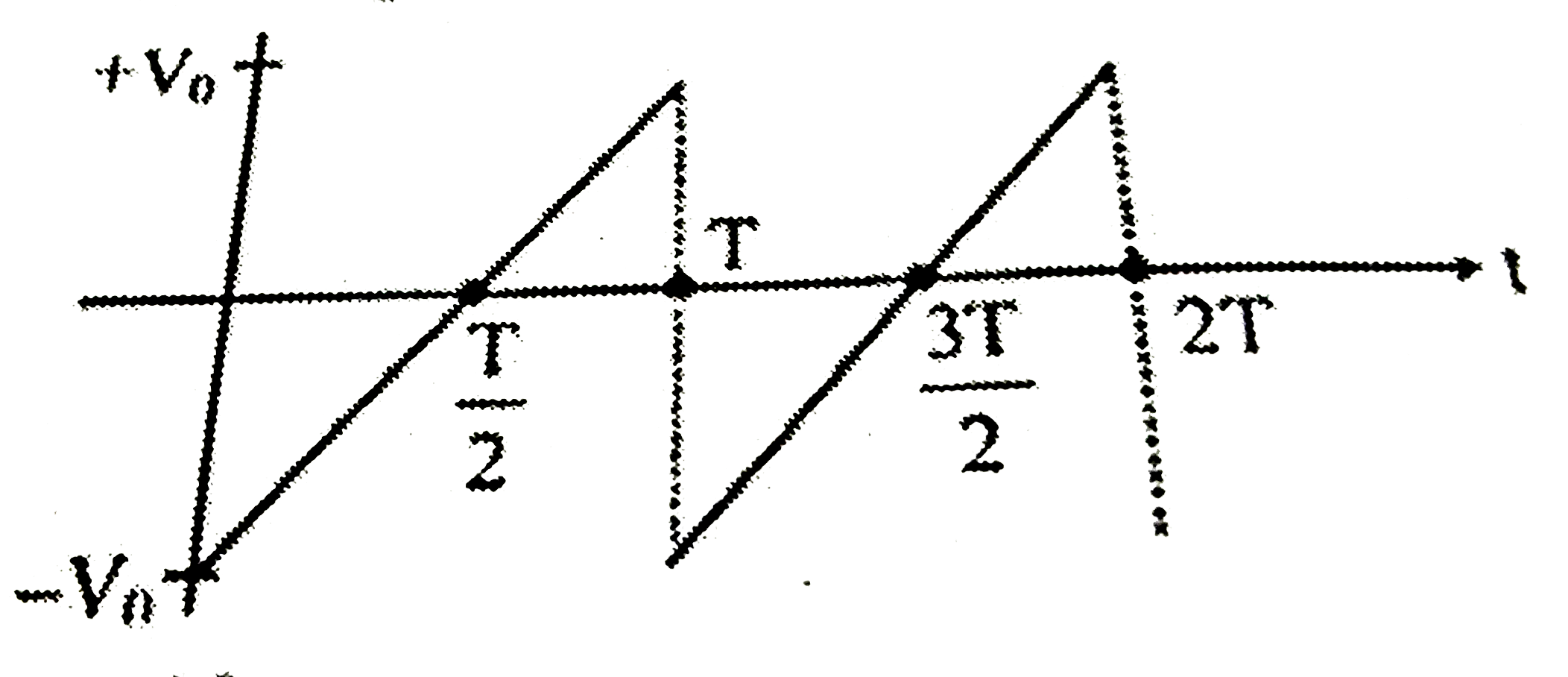
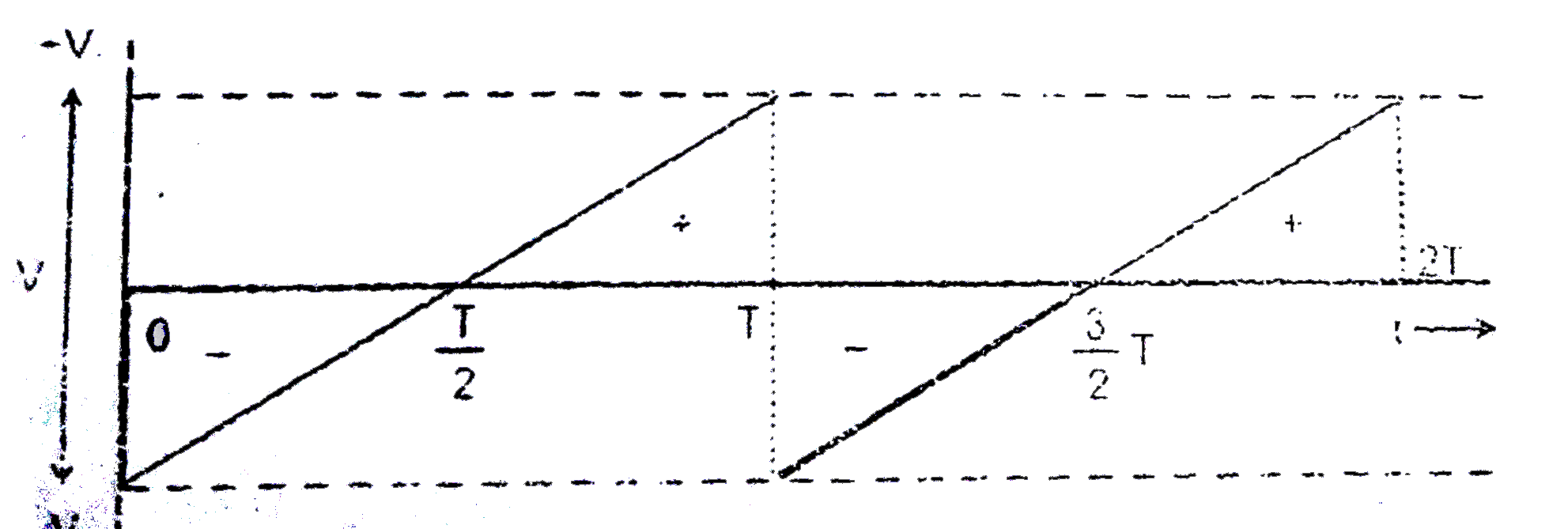
Rms value of the saw-tooth voltage of peak value V0 as shown in-
 AV02BV0√2CV03DV0√3
AV02BV0√2CV03DV0√3
The voitage time (V−t) graph for triangular wave having peak value V0 is as shown in the figare. The rins value of V in time interval from t=0 to T4 is Y0√n. Find n.
'(##CEN_KSR_PHY_JEE_C24_E01_006_Q03##)'View SolutionThe voltage time (V-t) graoh for triangular wave having peak value (V0) is as shown in fig. The rms value V in time interval t=0 to t=T/4 is
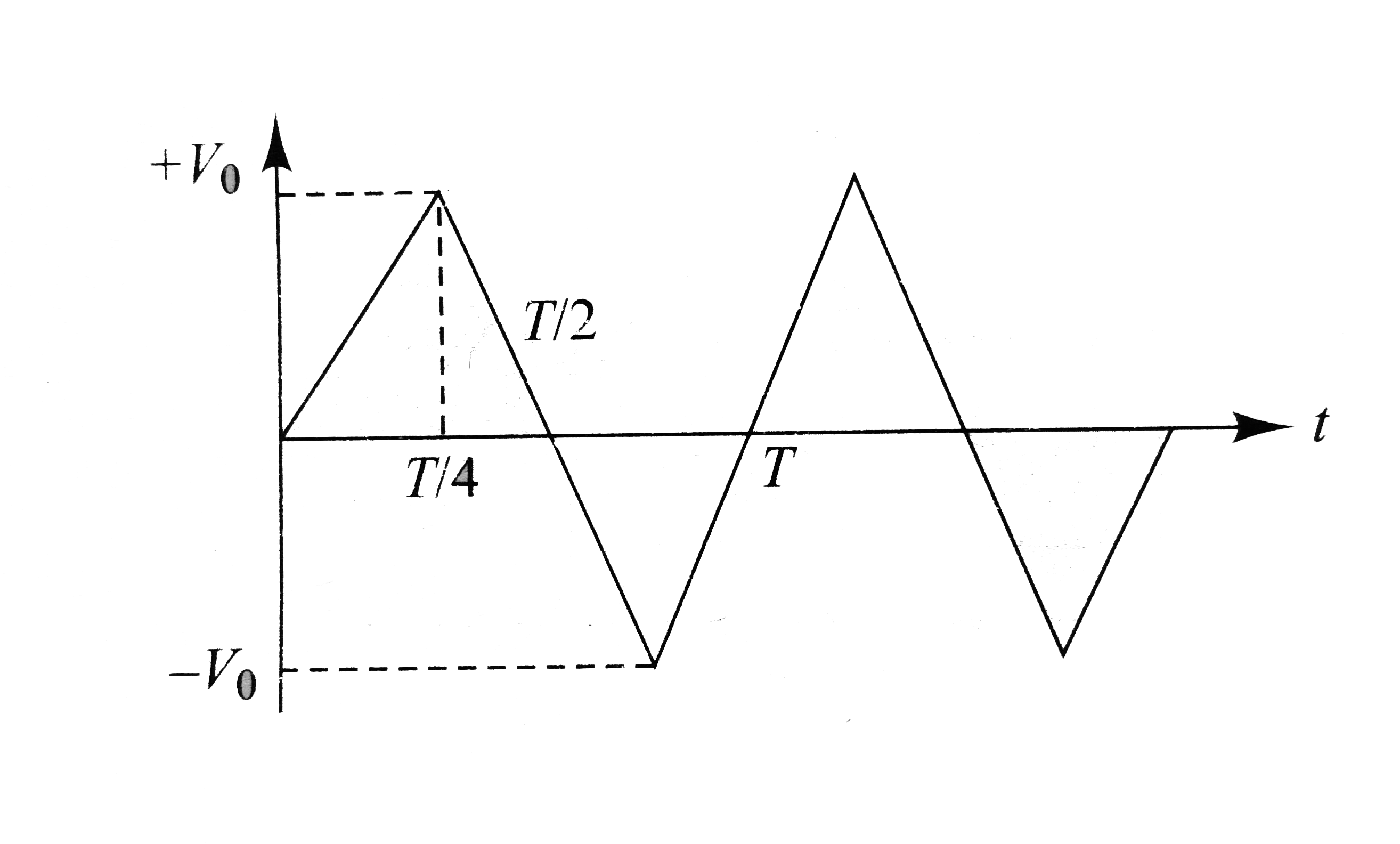 View Solution
View Solution
The velocity- time (v−t) graph shown above illustratesView SolutionThe velocity (v)-time (t) graph of a partide moving along a straight line is as shown in the figure. The displacement of the particle from t = 0 to t = 4 is
 View Solution
View SolutionFind the aveage for the saw-tooth voltage of peak value V0 from t=0 to t=2T as shown in figure.
 View Solution
View Solution

