A parallel plate capacitor of plate A and plate sepration d is charged to potential difference V and then the battery is disconnected. A slab of dielectric constatn K is then inserted between the plates of the capacitor so as to fill the space between the plates. If Q, E and W denotes respectively the magnitude of charge on each plate, the electric field between the plates (after the slab is inserted) and work done on the system, in the process of inserting the slab, then
Q=ε0AVd
Q=ε0KAVd
E=VKd
W=ε0AV22d[1−1K]
The correct Answer is:A, C, D
ON inserting the dielectric slab electric charge on plates remain unchanged at Q=ε0AVd in accordance with conservation of charge principal. But new E.F is 1K tiems of original value. Hence,E=VKd.As now p.d. V.=VK
Hence W=12QV−12QV.=QV2[1−1k]orW=ε0AVd.V2[1−1k]=ε0AV22d[1−1k]
Similar Questions
A parallel plate capacitor of plate area A and plate separation d is charged to potential difference V and then the battery is disconnected. A slab of dielectric constant K is then inserted between the plates of the capacitor so as to fill the space between the plates. If Q, E and W denote respectively, the magnitude of charge on each plate, the electric field between the plates (after the slab is inserted), and work done on the system, in question, in the process of inserting the slab, then
View SolutionA parallel - plate capacitor of plate area Aand plate separation d is charged to a potential difference V and then the battery is disconnected . A slab of dielectric constant K is then inserted between the plate of the capacitor so as to fill the space between the plate .Find the work done on the system in the process of inserting the slab.
View Solution
Knowledge Check
A parallel plate capacitor of plate area A and plate separation d is charged to potential difference V and then the battery is disconnected. A slab of dielectric constant K is then inserted between the plates of the capacitor so as to fill the space between the plates. If Q, E and W denote respectively, the magnitude of charge on each plate, the electric field between the plates (after the slab is inserted), and work done on the system, in question, in the process of inserting the slab, then
A(a) Q=ε0AVdB(b) Q=ε0KAVdC(c) E=VKdD(d) W=ε0AV22d[1−1K]Figure shows a parallel plate capacitor with plate area A and plate separation d. A potential difference is being applied between the plates. The battery is then disconnected and a dielectric slab of dieletric constant K is placed in between the plates of the capacitor as shown.
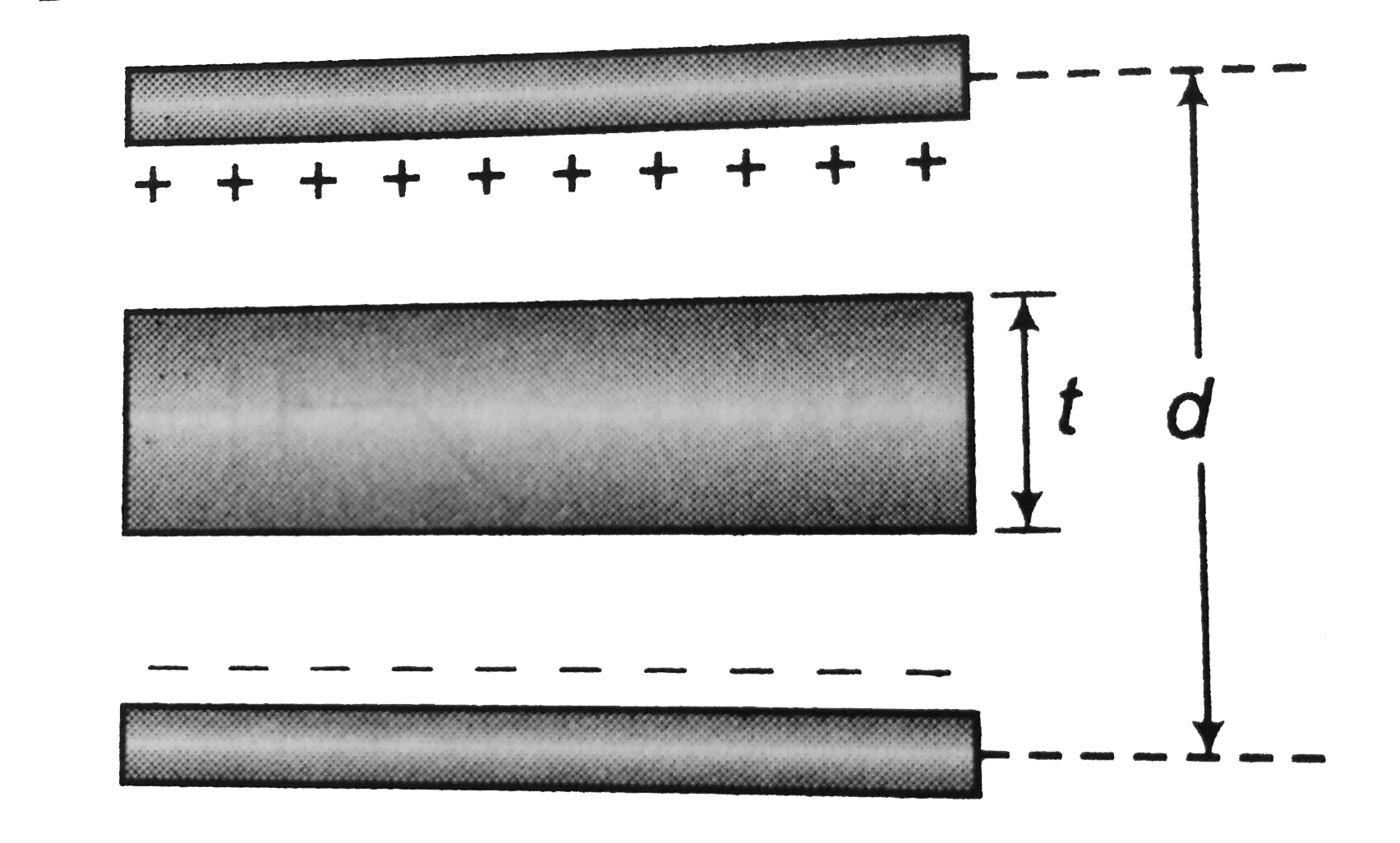
The electric field in the gaps between the plates and the electric slab will beAε0AVdBVdCKVdDVd−tA parallel plate capacitor of capacitance C0 is charged with a charge Q0 to a potential difference V0 and the battery is then disconnected Now a dielectric slab of dielectric constant k is inserted between the plates ofcapacitor. The dimensions of the slab are such that it completely fills the space between the plates, then :
ACharge on the' plates remains the sameBCharge on the plates decreases to Q0/KCCharge on _the plates increases to KQ0DPotential difference between plates remains the same
A parallel - plate capacitor of plate area A and plate separation d is charged to a potential difference V and then the battery is disconnected . A slab of dielectric constant K is then inserted between the plate of the capacitor so as to fill the space between the plate .Find the work done on the system in the process of inserting the slab.
View SolutionA parallel-plate capacitor of plate area A and plate separation d is charged by a ideal battery of e.m.f. V and then the battery is disconnected. A slab of dielectric constant 2k is then inserted between the plates of the capacitor so as to fill the whole space between the plates. Find the change in potential energy of the system in the process of inserting the slab.
View SolutionA dielectric slab is inserted between the plates of an isolated capacitor. The force between the plates will
View SolutionFigure shows a parallel plate capacitor with plate area A and plate separation d. A potential difference is being applied between the plates. The battery is then disconnected and a dielectric slab of dieletric constant K is placed in between the plates of the capacitor as shown.

The electric field in the gaps between the plates and the electric slab will be
View SolutionFigue shows a parallel plate capacitor with plate area A and plate separation d. A potential difference is being applied between the plates. The battery is then disconnected and a dielectric slab of dieletric constant K is placed in between the plates of the capacitor as shown.
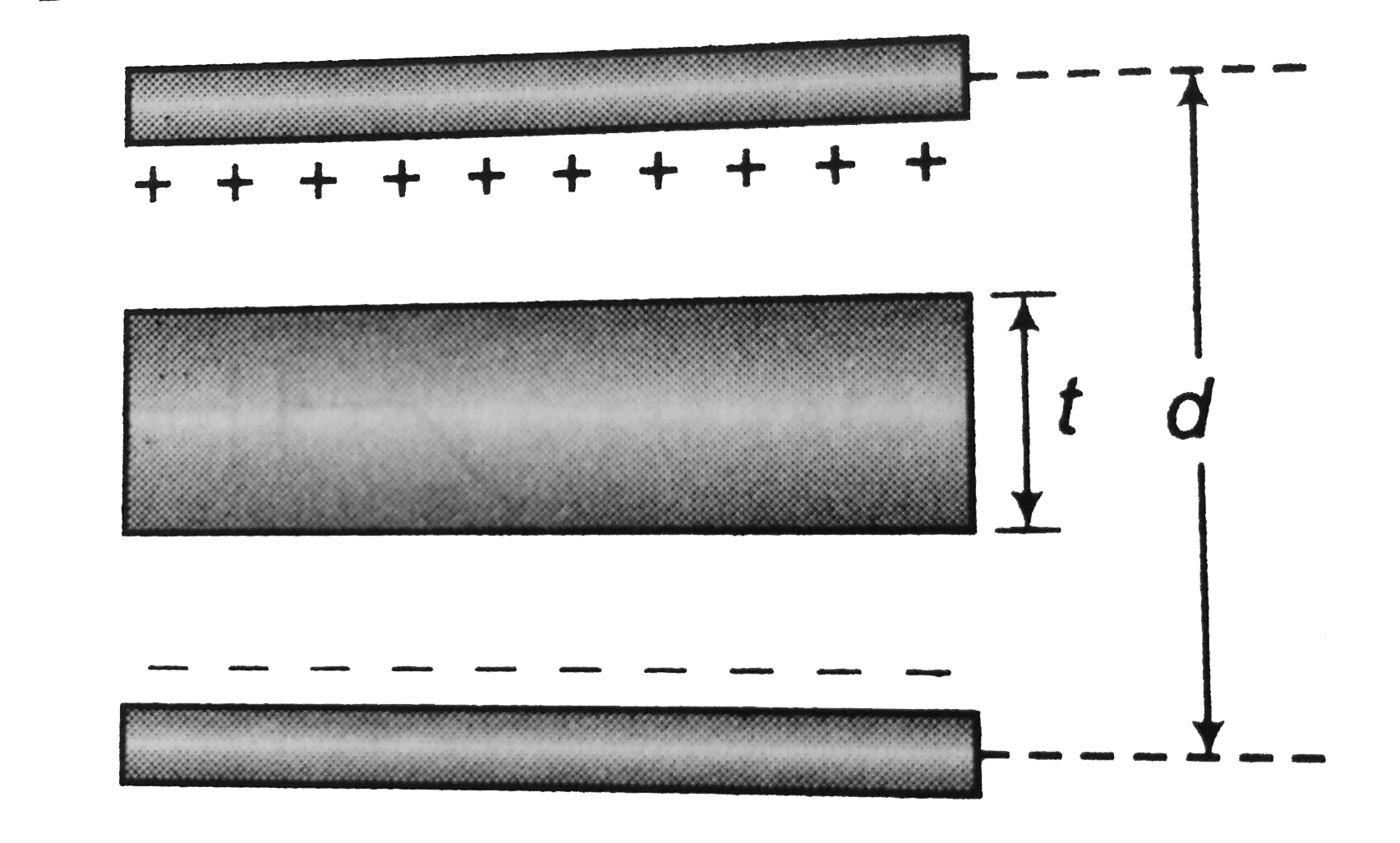
The electric field in the dielectric slab is
View Solution
