An alternate e.m.f. is applied to pure capacitance . Investigate the phase relationship between the current flowing through it and e.m.f. applied

Text Solution

Verified by Experts

Let us consider that an alternating e.m.f. $v = v_{m} sin ω t$ is applied to a pure capacitor of capacitance C as shown in the figure
$v = v_{m} sin ω t$ . . . (i)
Let .q. be the change on the capacitor at any instant, then the potential difference across the
capacitor $v_{c} = \frac{q}{C}$
But $v_{c} = v$
Substituting in eqn.(i) , we get $\frac{q}{C} = v_{m} sin ω t$
$\Rightarrow q = C v_{m} sin ω t$
Also $i = \frac{d q}{d t} = \frac{d}{d t} (C v_{m} sin ω t)$
$\Rightarrow i = C v_{m} \frac{d}{d t} (sin ω t) = C v_{m} (cos ω) ω$
$\Rightarrow i = \frac{v_{m}}{\frac{1}{C ω}} cos ω t$

$\Rightarrow i = \frac{v_{m}}{\frac{1}{C ω}} sin (ω + \frac{π}{2})$ [ $∴ cos ω t = sin (ω t + \frac{π}{2})$ ]
where $i_{m} = \frac{v_{m}}{\frac{1}{C ω}}$ is the peak value of alternating current $\frac{1}{C ω} = X_{C}$ is known as capacitive reactance of the capacitor
From equation (i) and (ii) it is clear that current leads the induced e.m.f. by an angle of $\frac{π}{2}$ . Time and phasor diagrams are shown in the figure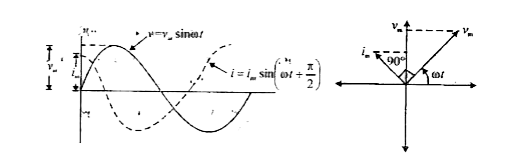
Average power in purely capacity circuit
$P = V_{r m s} I_{r m s} cos ϕ$
here $ϕ = \frac{π}{2} ∴ P = V_{r m s} I_{r m s} cos \frac{π}{2} = 0$

Updated on:21/07/2023

An alternating e.m.f. is applied to purely capacitive circuit. The phase relation between e.m.f. and current flowing in the circuit is or in a circuit containing capacitance only
Ae.m.f. is ahead of current by $π / 2$
BCurrent ahead of e.m.f by $π / 2$
CCurrent lags behind e.m.f. by $π$
DCurrent is ahead of e.m.f. by $π$
If an alternating e.m.f. is applied to a series L-R circuit, the phase angle between e.m.f. and current is given by $tan θ = \frac{ω L}{R}$ . Hence the power factor of the series L-R circuit is
A1. $\frac{ω L}{R}$
B2. $\frac{R}{ω L}$
C3. $\frac{R}{R + ω L}$
D4. $\frac{R}{\sqrt{R^{2} + ω^{2} L^{2}}}$
The phase angle between e.m.f. and current in LCR series ac circuit is
A0 to $π / 2$
B $π / 4$
C $π / 2$
D $π$