ABC is a triangle with base AB. D is a point on AB such that AB = 5 and DB = 3. What is the ratio of the area of ΔADC to the area of ΔABC ?
3/2
2/3
3/5
2/5
The correct Answer is:D
To find the ratio of the area of triangle ADC to the area of triangle ABC, we can follow these steps:
Step 1: Identify the lengths of the segments
Given:
- The length of AB = 5
- The length of DB = 3
From this, we can find the length of AD:
- Since D is a point on AB, we can calculate AD as:
AD=AB−DB=5−3=2
Step 2: Understand the area of triangles
The area of a triangle can be calculated using the formula:
Area=12×base×height
In our case, both triangles ADC and ABC share the same height (let's call it H), since they are both drawn from point C perpendicular to line AB.
Step 3: Calculate the areas of triangles ADC and ABC
- Area of triangle ABC:
AreaABC=12×AB×H=12×5×H
- Area of triangle ADC:
AreaADC=12×AD×H=12×2×H
Step 4: Set up the ratio of the areas
Now, we can find the ratio of the area of triangle ADC to the area of triangle ABC:
Ratio=AreaADCAreaABC=12×2×H12×5×H
Step 5: Simplify the ratio
The 12 and H cancel out:
Ratio=25
Conclusion
Thus, the ratio of the area of triangle ADC to the area of triangle ABC is:
25
Given:
- The length of AB = 5
- The length of DB = 3
- Since D is a point on AB, we can calculate AD as:
AD=AB−DB=5−3=2
The area of a triangle can be calculated using the formula:
Area=12×base×height
In our case, both triangles ADC and ABC share the same height (let's call it H), since they are both drawn from point C perpendicular to line AB.
- Area of triangle ABC:
AreaABC=12×AB×H=12×5×H
AreaADC=12×AD×H=12×2×H
Now, we can find the ratio of the area of triangle ADC to the area of triangle ABC:
Ratio=AreaADCAreaABC=12×2×H12×5×H
The 12 and H cancel out:
Ratio=25
Thus, the ratio of the area of triangle ADC to the area of triangle ABC is:
25
Topper's Solved these Questions
- MENSURATIONBOOK - KIRAN PUBLICATIONCHAPTER - MENSURATIONEXERCISE - TYPE - III
21 Videos
- MENSURATIONBOOK - KIRAN PUBLICATIONCHAPTER - MENSURATIONEXERCISE - TYPE - IV
169 Videos
- MENSURATIONBOOK - KIRAN PUBLICATIONCHAPTER - MENSURATIONEXERCISE - Test Yourself
28 Videos
- LCM AND HCF BOOK - KIRAN PUBLICATIONCHAPTER - LCM AND HCF EXERCISE - Test Yourself
18 Videos
- MISCELLANEOUSBOOK - KIRAN PUBLICATIONCHAPTER - MISCELLANEOUSEXERCISE - TYPE-VI
15 Videos
Similar Questions
In a triangle ABC , D & E are two points on sides AB and AC such that DE∣∣BC and AD:DB=3:5. Find the ratio of area of ΔADE to the area of trapezium BDEC.
View SolutionIf D is a point on the side AB of ΔABC such that AD:BD=3:2andE is a point on BC such that DE∣∣AC, find the ratio of the areas of ΔABCandΔDBE
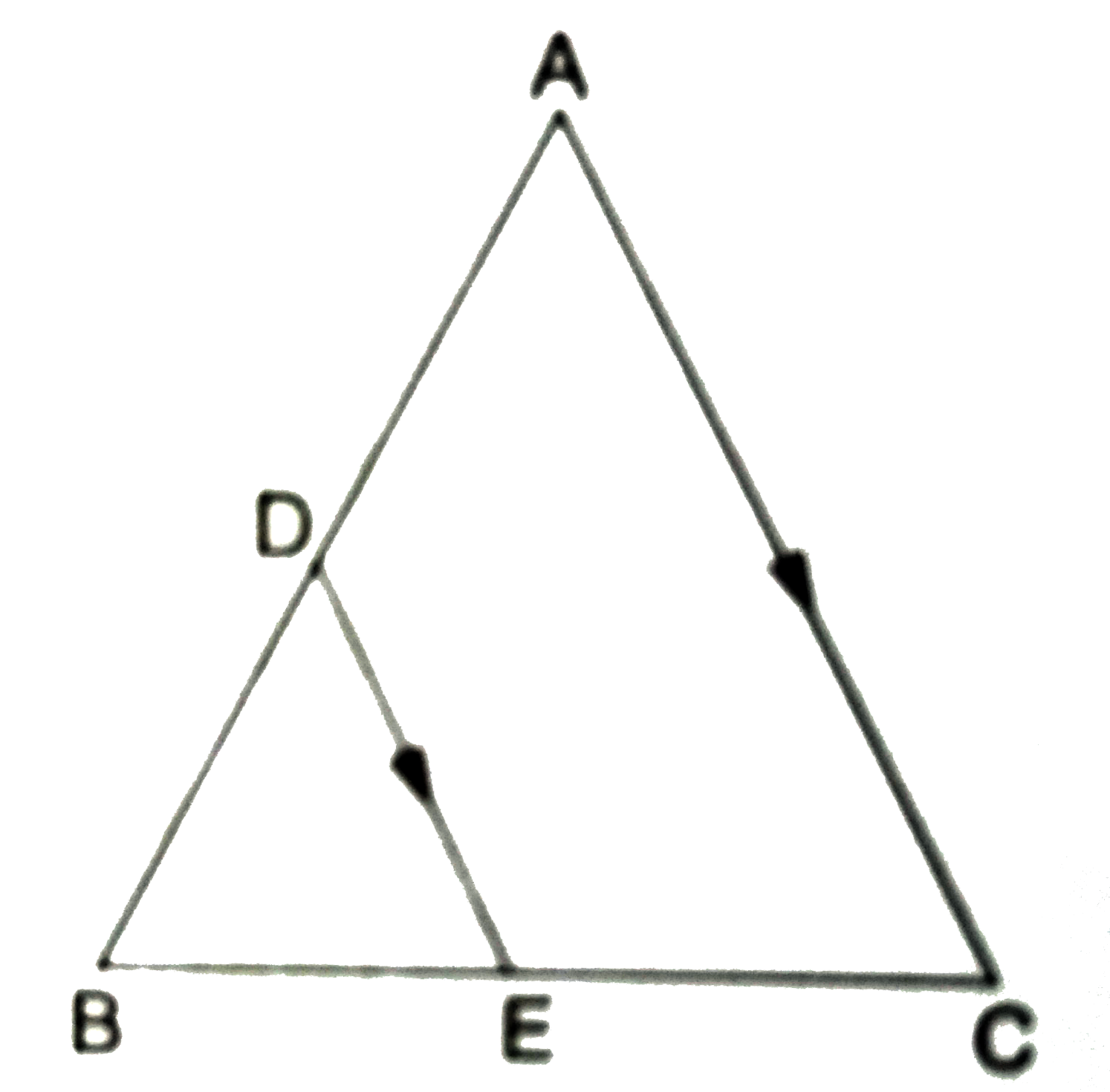
View Solution
Knowledge Check
ABC is a triangle with base AB. D is a point on AB such that AB = 5 and DB = 3. What is the ratio of the area of ΔADC to the area of ΔABC ?
A32B23C35D25In triangle ABC,D is the midpoint of AB, E is the midpoint of DB and F is the midpoint of BC. If the area of ΔABC is 96, the area of ΔAEF is
A16B24C32D36If ΔABC~ΔDEF such that AB=1.2 cm and DE =1.4 cm, the ratio of the areas of ΔABC and ΔDEF is :
A49:36B6:7C7:6D36:49
ABC is a triangle in which D, E and F are the mid-points of the sides AC, BC and AB respectively. What is the ratio of the area of the shaded to the unshaded region in the triangle?
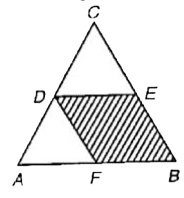
View SolutionIf ΔABC~ΔDEF such that AB = 1.2 cm and DE = 1.4 cm, the ratio of the areas of ΔABC and ΔDEF is :
View SolutionIn ΔABC,D and E are the points on sides AB and BC respectively such that DE || AC. If AD : DB = 5 : 3, then what is the ratio of the area of ΔBDE to that of the trapezium ACED ?
View SolutionTwo persons K and L enter into a business by investing their sum in the ratio of 7 : 9. After 7 months, L leaves the business. After eleven month, if total profit is Rs. 2460, what is the profit share of L (in Rs)?
View SolutionABC is a triangle in which ∠A=90∘ , AN⊥BC, BC=12 cm and AC=5 cm. Find the ratio of the areas of △ANC and △ABC.
View Solution
