If D and E are the mid-points of the side AB and AC respectively of the ΔABC in the figure given here, the shaded region of the triangle is what per cent of the whole triangular region?
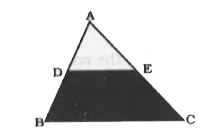
50%
25%
75%
60%
The correct Answer is:C
Step by step video & image solution for If D and E are the mid-points of the side AB and AC respectively of the DeltaABC in the figure given here, the shaded region of the triangle is what per cent of the whole triangular region? by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Updated on:21/07/2023Topper's Solved these Questions
- MENSURATIONBOOK - KIRAN PUBLICATIONCHAPTER - MENSURATIONEXERCISE - TYPE - III
21 Videos
- MENSURATIONBOOK - KIRAN PUBLICATIONCHAPTER - MENSURATIONEXERCISE - TYPE - IV
169 Videos
- MENSURATIONBOOK - KIRAN PUBLICATIONCHAPTER - MENSURATIONEXERCISE - Test Yourself
28 Videos
- LCM AND HCF BOOK - KIRAN PUBLICATIONCHAPTER - LCM AND HCF EXERCISE - Test Yourself
18 Videos
- MISCELLANEOUSBOOK - KIRAN PUBLICATIONCHAPTER - MISCELLANEOUSEXERCISE - TYPE-VI
15 Videos
Similar Questions
Knowledge Check
If D and E are the mid points of the sides AB and AC respectively of the triangle ABC in the figure given here, the shaded region of the triangle is what per cent of the whole triangular region?
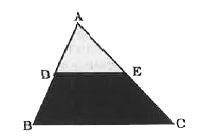 A50%B25%C75%D60%
A50%B25%C75%D60%If D and E are the mid points of the sides AB and AC respectively of the triangle ABC in the figure given here, the shaded region of the triangle is what per cent of the whole triangular region?
 A0.5B0.25C0.75D0.6
A0.5B0.25C0.75D0.6If in a triangle AB=a,AC=b and D,E are the mid-points of AB and AC respectively, then DE is equal to
Aa4−b4Ba2−b2Cb4−a4Db2−a2
If in a triangle ABC and D, E are the mid-points of AB and AC respectively, then →DE is equal to
View SolutionABC is a triangle in which D, E and F are the mid-points of the sides AC, BC and AB respectively. What is the ratio of the area of the shaded to the unshaded region in the triangle?
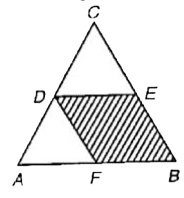
View SolutionIn a ΔABC,D,E,F are the mid -points of the sides BC, CA and AB respectively, the vector AD is equal to
View SolutionIf D, E and F are the mid-points of sides BC, CA and AB respectively of △ABC, then the ratio of the areas of △DEF to the area of △ABC is :
View SolutionIn a triangle ABC, D, E, F are the mid-points of the sides BC, CA and AB respectively , the vector →AD is equal to
View Solution
