O is centroid of ΔABC and AD, BE, CF are its three medians. If area of ΔAOE is 15 cm2, then area of quadrilateral BDOF is -
20 cm2
30 cm2
40 cm2
25 cm2
The correct Answer is:B
To solve the problem, we need to find the area of quadrilateral BDOF given that the area of triangle AOE is 15 cm² and O is the centroid of triangle ABC.
1. Identify the Medians and Centroid:
- In triangle ABC, the medians AD, BE, and CF intersect at point O, which is the centroid of the triangle. The centroid divides each median in the ratio 2:1.
2. Area of Triangle AOE:
- We are given that the area of triangle AOE is 15 cm².
3. Understanding the Areas of the Other Triangles:
- Since O is the centroid, it divides triangle ABC into six smaller triangles of equal area. Therefore, the areas of triangles AOE, BOE, COE, AOD, BOD, and COF are all equal.
- If area AOE = 15 cm², then the areas of triangles BOE, COE, AOD, BOD, and COF are also 15 cm² each.
4. Calculate the Area of Quadrilateral BDOF:
- Quadrilateral BDOF consists of triangles BOD and BOF.
- The area of triangle BOD = 15 cm² (as established).
- The area of triangle BOF = 15 cm² (as established).
- Therefore, the area of quadrilateral BDOF = Area of triangle BOD + Area of triangle BOF = 15 cm² + 15 cm² = 30 cm².
5. Conclusion:
- The area of quadrilateral BDOF is 30 cm².
Final Answer:
The area of quadrilateral BDOF is 30 cm².
- In triangle ABC, the medians AD, BE, and CF intersect at point O, which is the centroid of the triangle. The centroid divides each median in the ratio 2:1.
- We are given that the area of triangle AOE is 15 cm².
- Since O is the centroid, it divides triangle ABC into six smaller triangles of equal area. Therefore, the areas of triangles AOE, BOE, COE, AOD, BOD, and COF are all equal.
- If area AOE = 15 cm², then the areas of triangles BOE, COE, AOD, BOD, and COF are also 15 cm² each.
- Quadrilateral BDOF consists of triangles BOD and BOF.
- The area of triangle BOD = 15 cm² (as established).
- The area of triangle BOF = 15 cm² (as established).
- Therefore, the area of quadrilateral BDOF = Area of triangle BOD + Area of triangle BOF = 15 cm² + 15 cm² = 30 cm².
- The area of quadrilateral BDOF is 30 cm².
The area of quadrilateral BDOF is 30 cm².
Topper's Solved these Questions
- BOOK - LUCENT PUBLICATIONCHAPTER - CENTRE OF TRIANGLEEXERCISE - EXERCISE-6B8videos
CENTRE OF TRIANGLE
- BOOK - LUCENT PUBLICATIONCHAPTER - CENTRE OF TRIANGLEEXERCISE - EXERCISE-6B8videos
CENTRE OF TRIANGLE
- BOOK - LUCENT PUBLICATIONCHAPTER - ALGEBRAIC IDENTITIES EXERCISE - Exercise - 1B28videos
ALGEBRAIC IDENTITIES
- BOOK - LUCENT PUBLICATIONCHAPTER - CIRCLE AND ITS TANGENT LINESEXERCISE - EXERCISE 8B27videos
CIRCLE AND ITS TANGENT LINES
Similar Questions
Knowledge Check
- Question 1 - Select One
In a ΔABC, G is centroid and AD, BE, CF are medians. Find the area of quadrilateral BDGF, if the area of ΔABC=72cm2
A12 cm2B16cm2C24cm2D8cm2 - Question 2 - Select One
If O is the centroid and AD, BE and CF are the three medians of ΔABC with an area of 96cm2 then the area of ΔBOD in cm2 is
A8B12C16D24 - Question 3 - Select One
In a ΔABC,AD,BE and CF are three medians. The perimeter of ΔABC is always.
Aequal to (¯¯¯¯¯¯AB+¯¯¯¯¯¯BE+¯¯¯¯¯¯CF)Bgreater than (¯¯¯¯¯¯AD+¯¯¯¯¯¯BE+¯¯¯¯¯¯CF)Cless than (¯¯¯¯¯¯AD+¯¯¯¯¯¯BE+¯¯¯¯¯¯CF)DNone of these
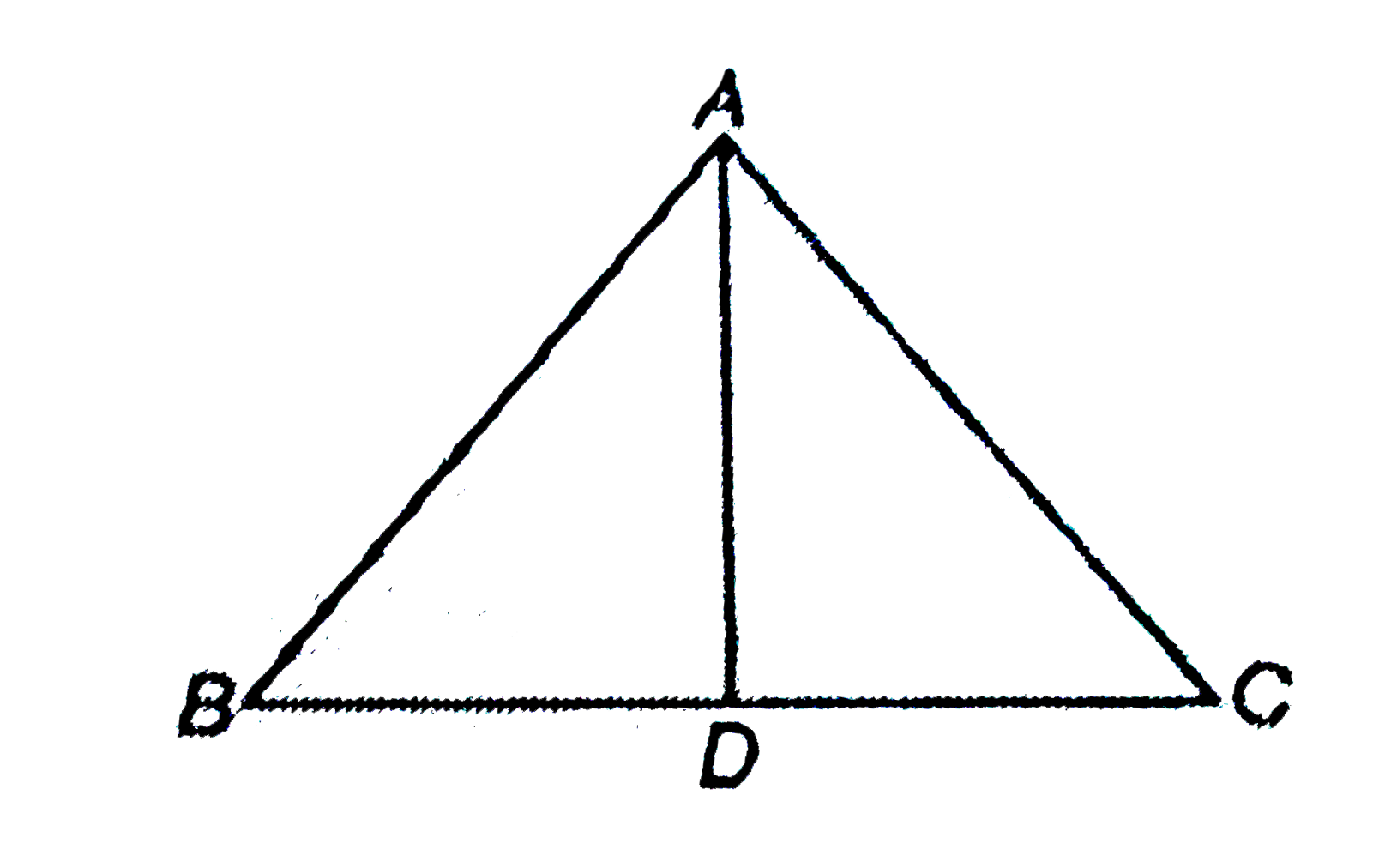
In the given figure AD is the median. If the area of ΔABD=10cm2, find the area of ΔABC
 View Solution
View SolutionIn a ΔABC all three medians AD, BE and CF intersects at o. If area of ABC IS 10 z sq units, then find area of quadrilateral BDOF .
View SolutionIf G is centre of ΔABC and median AD = 12 cm. Find AG
View SolutionThe three medians AD, BE and CF of ΔABC intersect at point G. If the area of ΔABC is 60 sq.cm. then the area of the quadrilateral BDGF is :
View SolutionIf D is the mid-point of the side BC of ΔABCand the area of ΔABD and the area of ΔACD is 16cm2, then the area of ΔABC is
View Solution