Find an expression for electrostatic potential due to an electric dipole.

Text Solution

Verified by Experts

Electrostatic Potential due to an Electric Dipole
Let us consider an electric dipole consisting of charges +q and -g separated by a distance 2a. The dipole moment $| p | = q \times 2 a$ . Let O be the centre of the dipole, P be the any point near the electric dipole inclined at an angle $θ$ as shown in the figure given below.

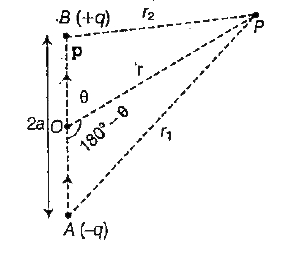
P be the point at which electric potential is required. Potential at P due to a -q charge,
$V_{1} = - \frac{q}{4 π ε_{0} r_{1}}$
Potential at P due to a +q charge,
$V_{2} = \frac{q}{4 π ε_{0} r_{2}}$
As potential is related to work done by the field, electrostatic potential also follows the superposition principle. Therefore, potential at P due to the dipole,
$V_{P} = V_{1} + V_{2}$
or $V_{P} = \frac{q}{4 π ε_{0}} (\frac{1}{r_{2}} - \frac{1}{r_{1}})$ .......(i)
Now, by geometry
$r_{1}^{2} = r^{2} + a^{2} + 2 a r cos θ$
Similarly, $r_{1}^{2} = r^{2} (1 + \frac{a^{2}}{r^{2}} + \frac{2 a}{r} cos θ)$
If $r > > a, \frac{a}{r}$ is small.
Therefore, $\frac{a^{2}}{r^{2}}$ can be neglected.
$r_{1}^{2} = r^{2} (1 + \frac{2 a}{r} cos θ)$
$\Rightarrow r_{1} = r {(1 + \frac{2 a}{r} cos θ)}^{1 / 2}$
Similarly, $\frac{1}{r_{2}} = \frac{1}{2} {(1 - \frac{2 a}{r} cos θ)}^{- 1 / 2}$
Using Binomial theorem,
$[{(1 + x)}^{n} = 1 + n x, x < < 1]$ and retaining terms upto the first order is $\frac{a}{r}$ , we obtain
$V = \frac{q}{4 π ε_{0} r} [(1 + \frac{a}{r} cos θ) - (1 - \frac{a}{r} cos θ)]$
$= \frac{q}{4 π ε_{0} r} [1 + \frac{a}{r} cos θ - 1 + \frac{a}{r} cos θ]$
$= \frac{q}{4 π ε_{0} r} (\frac{2 a cos θ}{r})$
$V = \frac{p cos θ}{4 π ε_{0} r^{2}} [∴ p = q \times 2 a]$
As, $ρ cos θ = p . ˆ r$
where, $ˆ r$ is the Unit .vector along the position vector $O P = ˆ r$ .
$∴$ Electrostatic potential at P due.to a short dipole $(a < < r)$ is
$V = \frac{p . ˆ r}{4 π ε_{0} r^{2}}$

Updated on:21/07/2023

|

Class 12 PHYSICS ELECTRIC CHARGES AND FIELDS

Similar Questions

Recommended Questions

Home
Ask Doubt
Profile