A parallel plate capacitor has plate separation d and the area of each plate is A. The space between the plates is completely filled with a dielectric constant K. Derive the expression for the capacity of the parallel plate capacitor.

Text Solution

Verified by Experts

Parallel plate capacitor consists of two thin conducting plates each of area A held parallel to each other at a suitable, distance d. One of the plates is insulated and other is earthed. There is vacuum between the plates.

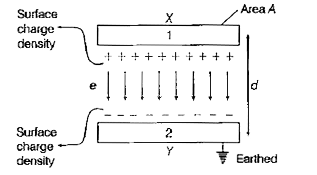
Suppose the plate X is given a charge of + q coulomb. By induction, ~q coulomb of charge is produced on the inner surface of the plate y and +q coulomb on the outer surface. Since, the plate Y is connected to the earth, the + q charge on the outer surface flows to the earth. Thus, the plates X and Y have equal and opposite charges.
Suppose the surface density of charge on each plate is $σ$ . We know that the intensity of electric field at a point between two planes parallel sheets of equal and opposite charges is $σ / ε_{0}$ , where $ε_{0}$ is the permittivity of free space. The intensity of electric field between the plates will be given by:
$α = \frac{q}{A}$
and so, $E = \frac{q}{ε_{0} A}$ .......(i)
Now, let the potential difference between the two plates be V volt. Then, the electric field between the plates is given by
$E = \frac{V}{d}$
or V = Ed
On substituting the value of E from Eq. (i), we get
$V = \frac{q d}{ε_{0} A}$
$∴$ Capacitance of the capacitor is given by
$C = \frac{q}{V} = \frac{q}{q d / ε_{0} A}$ or $C = \frac{ε A}{d}$
where, $ε_{0} = 8.85 \times 10^{- 12} F / m$
Consider a dielectric inserted between the plates fully occupying the intervening region. The dielectric is polarised by the field with surface charge densities $σ$ and $- σ_{P}$ .
So, net electric field between the plates,
$E = \frac{σ - σ_{P}}{ε_{0}}$
For linear dielectrics, we expect aP to be proportional to $E_{0}$ i.e. to $σ$ .
Thus, $(σ - σ_{P})$ is proportional to a and we can write,
$σ - σ_{P} = \frac{σ}{K}$
where, K is a constant of characteristic of the dielectric. Clearly,
$K > 1 [σ_{P} < σ]$
then, $V = \frac{σ d}{ε_{0} K} = \frac{q d}{A ε_{0} K}$
$∴$ The capacitance C .with dielectric between the plates is given by
$C = \frac{q}{V} = \frac{ε_{0} K A}{d}$

Updated on:21/07/2023