Question

A simple pendulum having a bob of mass m under goes small oscillations with amplitude ${\displaystyle {\theta }_0}$ Find the tension in the string as a function of the angle made by the string with the vertical. When in this tension maximum, and when is it minimum?

Explanation

Suppose the speed of the bob the angle `theta` is v. Using conservation of energy between the extreme position and the position with angle theta.
`=1/2mv^2=mgl(costheta-costheta_0)` ……….i

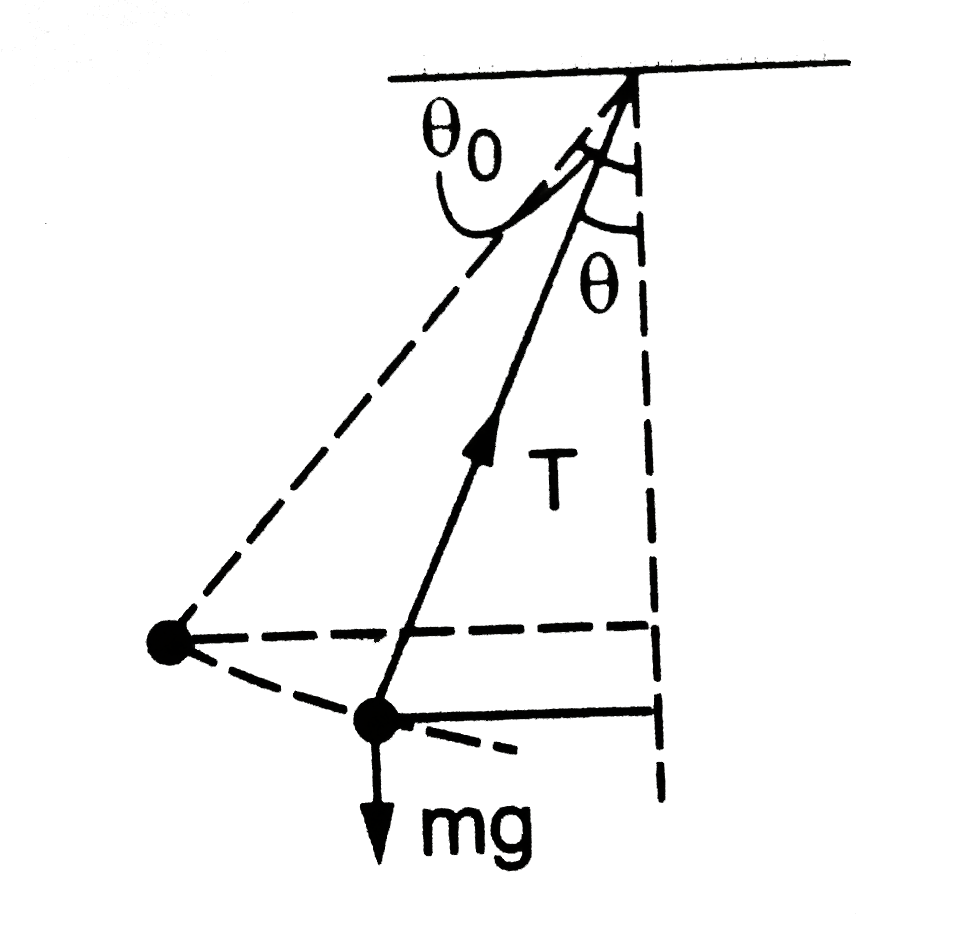
As the bob moves in a circular path, the force towards the centre shoudl the equal to `mv^2/l`. Thus,
`T-mgcostheta=mv^2/l`
`
using i
`ort T-mgcostheta=2mg(costheta-costheta_0)`
or `T=3mgcostheta-2mgcostheta)`
Now `costheta` is maximum at theta=0 and decreases as `|theta| increases (for |theta|lt90^@)`.
Thus the tension is maximum when `theta=0, ` i.e. at the mean position and is minimum when `theta=+-theta_0` i.e., at extreme positions