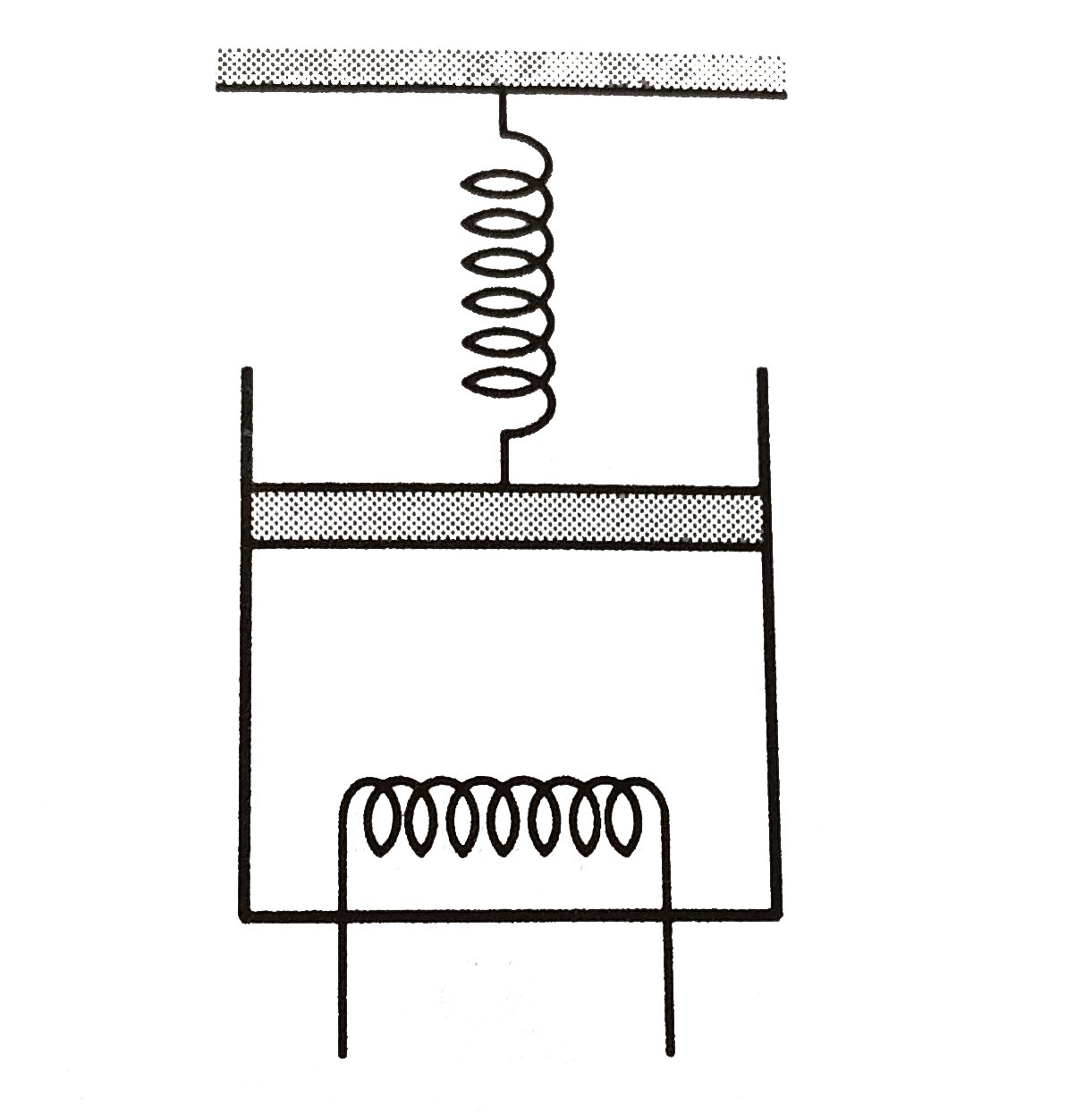
2.00 mole of a monatomic ideal gas (U=1.5nRT) is enclosed in an adiabatic, fixed , vertical cylinder fitted with a smooth, light adiabatic piston. The piston is connected to a vertical spring of spring constant 200Nm−1 as shown in .the area of cross section of the cylinder is 20.0cm2. initially, the spring is at its natural length and the temperature of the gas is 300K at its natural length. the atmosphere pressure is 100 kPa. the gas is heated slowly for some time by means of an electric heater so as to move the position up through 10 cm. find (a) the work done by the gas (b) the final temperature of the gas and (c ) the heat supplied by the heater.
 Solution in Tamil
Solution in Tamil
(a) the force by the gas on the piston is
F=p0A+ks
Where p0=100kPa is the atmospheric pressure, A=20cm2 is the area of cross section, k=200Nm1 is the spring constant and x is the compression of the spring. The work done by the gas as the piston moves throughl=10cmis<br>W=int_(0)^(l) Fdx<br>=p_(0)Al+(1)/(2)kl^(2)<br>=(100×103Pa)×(20×10−4m2)×(10×10−2m)
+12(200Nm−1)×(100×10−4m2)
=20J+1J=21J.
(b) The initial tempreture is T1=300K. Let the final tempreture be T2 We have
nRT1=p0V0
and nRT2=pV_(2)=(p0+klA)(V0+Al)
=nRT1+p0Al+kl2+(klnRT1)/(Ap_(0))<br>or,T_(2)=T_(1)+(p_(0)Al+kl^(2))/(nR)+(klT_(1))/(Ap_(0))<br>=(300 K)+(20 J+2 J)/((2.0 mol)(8.3 JK^(-1) mol^(-1))<br>+((200 Nm^(-1))xx(10xx10^(-2)m)xx(300 K))/((20xx10^(4)m^(2))xx(100xx10^(3)Pa))<br>=300 K+1.325 K+30 K<br>=331 K.<br>(c)the∫ernale≠rgyisU=1.5nRT.<br>thechan≥∈∫ernale≠rgyis<br>DeltaU=1.5nRDeltaT<br>=1.5xx(2.00 mol)xx(8.3 J K^(-1) mol^(-1)xx(31 K)<br>=772 J
From the first law,
ΔQ=ΔU+ΔW
772J+21J=793J.
Topper's Solved these Questions
- LAWS OF THERMODYNAMICSBOOK - HC VERMACHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Objective I
9 Videos
- LAWS OF THERMODYNAMICSBOOK - HC VERMACHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Objective II
5 Videos
- LAWS OF THERMODYNAMICSBOOK - HC VERMACHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Short Answer
14 Videos
- KINETIC THEORY OF GASESBOOK - HC VERMACHAPTER - KINETIC THEORY OF GASESEXERCISE - All Questions
120 Videos
- NEWTON'S LAWS OF MOTIONBOOK - HC VERMACHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - Exercises
42 Videos
Similar Questions
The first law of theromodynamics is a statement of
View SolutionThe pressure p and volume V of an ideal gas both increase in a process.
View SolutionShould the internal energy of a system necessarily increase if heat is added to it?
View SolutionCalculate the rms speed of nitrogen at STP (pressure =1 atm and temperature =00C. The density of nitrgoen in these conditions is 1.25kgm−3
View SolutionA block of mass 2 kg placed on a long frictionless horizontal table is pulled horizontally by a constant foerce F. It is found to move 10 m in the first two seconds. Find the magnitude of F.
View SolutionAn ideal monatonic gas is confined in a cylinder by a spring-loaded piston of cross section 8.0×10−3m2.initially the gas is at 300K and occupies a volume of 2.4×10−3m−3 and the sprinng is in its relaxed state. The gas is heated by a small heater until the piston moves out slowly by 0.1m. Calculate the final tenoerature of the gas. The force constant if the spring is 8000Nm−1,and the atmospheric pressure is 1.0×105Nm−2.Htecyl∈derandtheπs→narethermally∈sylated.Theπs→nandthespr∈garemass≤ssandthereisnoictionbetweentheπs→nandthecyl∈der.Neg≤ctanyheat−lossthroughthe≤adwiresoftheheater.Theheat∩acityoftheheatercoilis¬ligib≤.

View Solution2.00 mol of a monatomic ideal gas (U=1.5nRT) is enclosed in an adiabatic, fixed , vertical cylinder fitted with a smooth, light adiabatic piston.the piston is connected to a vertical spring of spring constant 200 N m^(-1) as shown in .the area of cross section of the cylinder is 20.0 cm^(2) . initially, the spring is at its natural length and the temperature of the gas is at its natural length and the temperature of the gas is 300 K. the atmosphere pressure is 100 kPa. the gas is heated sloewly for some time by means of an electric heater so as to move the poston up through 10 cm. find (a) the work done by the gas (b) the final temperature of the gas and (c ) the heat supplied by the heater.
View SolutionA monatomic ideal gas, initially at temperature T_1, is enclosed in a cylinder fitted with a frictionless piston. The gas is allowed to expand adiabatically to a temperature T_2 by releasing the piston suddenly. If L_1 and L_2 are the length of the gas column before expansion respectively, then (T_1)/(T_2) is given by
View SolutionMethod 3 of W Mass of a piston shown in Fig. is m and area of cross-section is A. Initially spring is in its natural length. Find work done by the gas.
View SolutionAn ideal gas at NTP is enclosed in an adiabatic vertical cylinder havin an area of cross section A=27cm2 between two light movable pistons as shown in figure. Spring with force constant K=37−−N/m is in a relaxed state initially. Now the lower position is moved upwards a distance h/2, h being the initial length of gas column . It is observed that the upper piston moves up by a distance h/16. Final temperature of gas4/3×273K. Take γ for the gas to be 3/2.

When the lower pistone is moved upwards a distance h/2, the compression.
View SolutionA gas fills the right portion of a horizontal cylinder whose radius is 5.00 cm . The initial pressure of the gas is 101 kPa . A frictionless movable piston separates the gas from the left portion of the cylinder, which is evacuated and contains an ideal spring, as the drawing shows. The piston is initially unstrained, and the length of the gas-filled portion is 20.0 cm . Wheen the pin is removed and the gas is allowed to expand, the length of the gas-filled chamber doubles when it finally reaches equilibrium. The initial and final temperatures are equal. Determine the spring constant of the spring.
View SolutionAn ideal monoatomic gas is enclosed in a fixed horizontal adiabatic cylinder of cross sectional area A. The cylinder is fitted with an adiabatic piston of mass m (attached to one end of a spring as shown) which can move horizontally without friction inside the cylinder. In equilibrium, the spring is in natural length and pressure and volume are P_0 and V_0 respectively. The piston is slightly displaced from equilibrium and released. Then, frequency of small oscillation is
View SolutionA monoatomic ideal gas, initially at temperature T_(1) is enclosed in a cylinder fitted with a frictionless piston. The gas is allowed to expand adiabatically to a temperature T_(2) by releasing the piston suddenly. If L_(1) and L_(2) are the lengths of the gas column. before and after expansion respectively, then (T_(1))/(T_(2)) is given by
View SolutionTwo moles of an ideal monatomic gas are confined within a cylinder by a massless and frictionless spring loaded piston of cross-sectional area 4 xx 10^(-3) m^2 . The spring is, initially, in its relaxed state. Now the gas is heated by an electric heater, placed inside the cylinder, for some time. During this time, the gas expands and does 50 J of work in moving the piston through a distance 0.10 m. The temperature of the gas increases by 50 K. Calculate the spring constant and the heat supplied by the heater.
View SolutionS is a piston of mass M which can slide inside a cylinder without friction. The walls of the cylinder is adiabatic and the piston is diathermic and area of cross section of cylinder is A. Length of the cylinder is 2l_0 . Initial pressure of each chamber is P_0 . Volume of the chambers are equal. Initially spring is unstretched and has spring constant k. The spring is fixed with piston and wall of the cylinder as shown in the figure. Find out its time period when piston is given small displacement.
View SolutionAn ideal gas at NTP is enclosed in an adiabatic vertical cylinder having area of cross section A=27 cm^2 , between two light movable pistons as shown in the figure. Spring with force constant k = 3700 N/m is in a relaxed state initially. Now the lower piston is moved upwards a height h/2, h being the initial length of gas column. It is observed that the upper piston moves up by a distance h/16. Find h taking gamma for the gas to be 1.5. Also find the final temperature of the gas.
View Solution
