A conducting circular loop of radius r is rotated about its diameter at a constant angular velocity ω in a magnetic field B perpendicular to the axis of rotation. In what position of the loop is the induced emf zero?Solution in Tamil
suppose, the normal to the loop is parallel to the magnetic field at t=o
at time t, the normal will make an angleθ=ωtwiththisposition<br>Thefluxofthemag≠ticfieldthroughthel∞pisPhi B pir^2 cos omega tsotˆthe∈ducedemfattimetis epsilon = omega B pi r ^2 sin omega t
This is zero when omegat = n pi, i
e
, when theta, pi 2pi,
, etc
these are the positons when the plane of the loop is normal to the magnetic field
It may be noted that at these positions, the flux has the maximum magnitude
Topper's Solved these Questions
- BOOK - HC VERMACHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Questions for short answer10videos
ELECTROMAGNETIC INDUCTION
- BOOK - HC VERMACHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Objective 112videos
ELECTROMAGNETIC INDUCTION
- BOOK - HC VERMACHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXERCISE98videos
ELECTROMAGNETIC INDUCTION
- BOOK - HC VERMACHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - Exercises75videos
ELECTRIC FIELD AND POTENTIAL
- BOOK - HC VERMACHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Exercise9videos
ELECTROMAGNETIC WAVES
Similar Questions
A short magnet is moved along the axis of a conducting loop. Show that the loop repels the magnet if the magnet is approaching the loop and attracts the magnet if it is going away from the loop.
04:28View SolutionA rod of length l rotates with a small but uniform angular velocity ω about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. The potential difference between the centre of the rod and an end is
04:43View SolutionCalculate the dimensions of (a) ∫→E.d→l,(b)vBlanddΦ0dt. The symbols have their usual meanings.
01:44View SolutionFind the dimensional formula of ε0 .
01:53View SolutionShow that the dimensions of the displacement current (ε0dΦEdt) are that of an electric current.
04:59View SolutionA uniform circular loop of radius a and resistance R palced perpendicular to a uniform magnetic field B . One half of the loop is rotated about the diameter with angular velocity omega as shown in Fig. Then, the current in the loop is
04:04View SolutionA conducting loop rotates with constant angular velocity about its fixed diameter in a uniform magnetic field, whose direction is perpendicular to that fixed diameter.
03:22View SolutionA non-conducting disk of radius R is rotating about its own axis with constant angular velocity omega in a perpendicular uniform magnetic field B as shown in figure. The emf induced between centre and rim of disk is
04:50View SolutionA circular wire of radius r rotates about its own axis with angular speed w in a magnetic field B perpendicular to its plane, then the induced e.m.f. is
05:22View SolutionA very small circular loop of radius a is initially (at t = 0) coplanar and concentric with a much larger fixed circular loop of radius b. A constant current I flows in the larger loop. The smaller loop is rotated with a constant angular speed omega about the common diameter. The emf induced in the smaller loop as a function of time t is
04:37View SolutionA square loop of side a is rotating about its diagonal with angular velocity ω in a perpendicular magnetic field →B It has 10 turns. The emf induced is
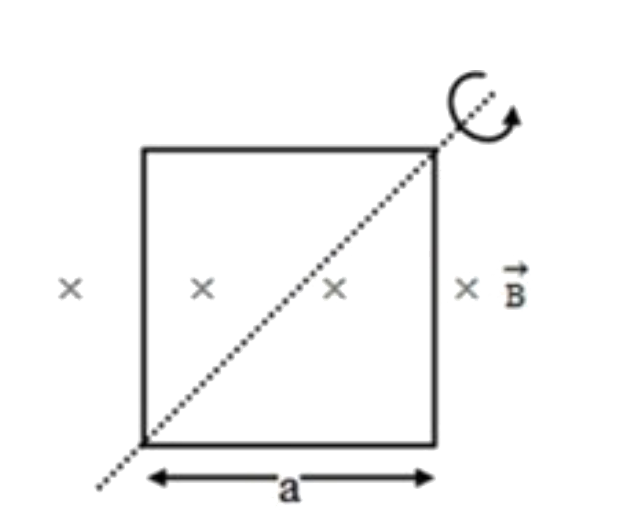 03:51View Solution
03:51View SolutionA planar wire loop is placed perpendicular to uniform magnetic field . Wire loop starts rotating in its own plane about its axis with uniform angular velocity ω. If B is magnetic field intensity then what will be the induced emf in the loop ?
View SolutionA circular loop of metal wire of radius r is placed perpendicular to uniform magnetic field B. Half of the loop is folded about the diameter with constant angular velocity omega . If resistance of the loop is R then current in the loop is
05:16View SolutionA circular loop is kept perpendicular to a uniform magnetic field. Loop is rotated about its diameter with constant angular velocity.
06:00View SolutionA very small circular loop of radius a is initially (at t = 0) coplanar and concentric with a much larger fixed circular loop of radius b. A constant current I flows in the larger loop. The smaller loop is rotated with a constant angular speed ω about the common diameter. The emf induced in the smaller loop as a function of time t is
02:36View SolutionA very small circular loop of radius a is initially (at t = 0) coplanar and concentric with a much larger fixed circular loop of radius b. A constant current l flows in the larger loop. The smaller loop is rotated with a constant angular speed omega about the common diameter. The emf induced in the smaller loop as a function of time t is
02:07View Solution

