Consider an electric field →E=E0ˆx , where E0 is a constant . The flux through the shaded area (as shown in figure ) due to this field is
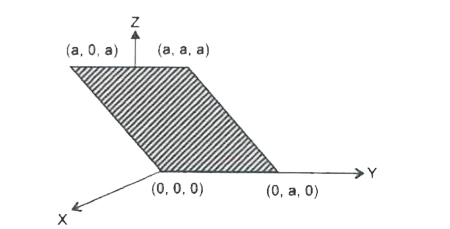
2E0a2
√2E0a2
E0a2
E0a2√2
The correct Answer is:C
Flux through shaded area =E0 (Projected area) E0a2 .
Topper's Solved these Questions
- ELECTROSTATICSBOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATICSEXERCISE - RECENT COMPETITIVE QUESTIONS
39 Videos
- ELECTROSTATICSBOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATICSEXERCISE - LEVEL -II
74 Videos
- ELECTRONS & PHOTONSBOOK - MODERN PUBLICATIONCHAPTER - ELECTRONS & PHOTONSEXERCISE - RECENT COMPETITIVE QUESTIONS
20 Videos
- GRAVITATIONBOOK - MODERN PUBLICATIONCHAPTER - GRAVITATIONEXERCISE - Recent Competitive Questions
8 Videos
Similar Questions
Knowledge Check
Conisder an electric field →E=E0ˆx where E0 is a constant .
The flux through the shaded area (as shown in the figure) due to this field is
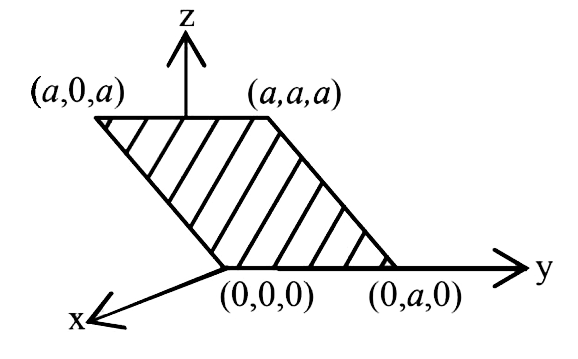 A(a) 2E0a2B(b) √2E0a2C(c) E0a2D(d) E0a2√2
A(a) 2E0a2B(b) √2E0a2C(c) E0a2D(d) E0a2√2If →E=E0Xaˆi(x−mt) then flux through the shaded area is:
 AE0a2BZeroCE0a3D−E0a3
AE0a2BZeroCE0a3D−E0a3Find the potential V of an electrostatic field →E=a(yˆi+xˆj), where a is a constant.
Aaxy+CB−axy+CCaxyD−axy
The potential field of an electric field →E=(yˆi+xˆj) is
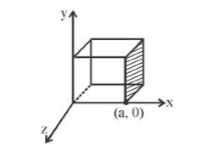
View SolutionGiven the components of an electric field as Ex=αx,Ey=0 and Ez=0, where α is a dimensional constant. Calculate the flux through each face of the cube of side a, as shown in the figure.

View SolutionThe potential field of an electric field →E=(yˆi+xˆj) is
View SolutionThe potential field of an electric field →E=(yˆi+xˆj) is
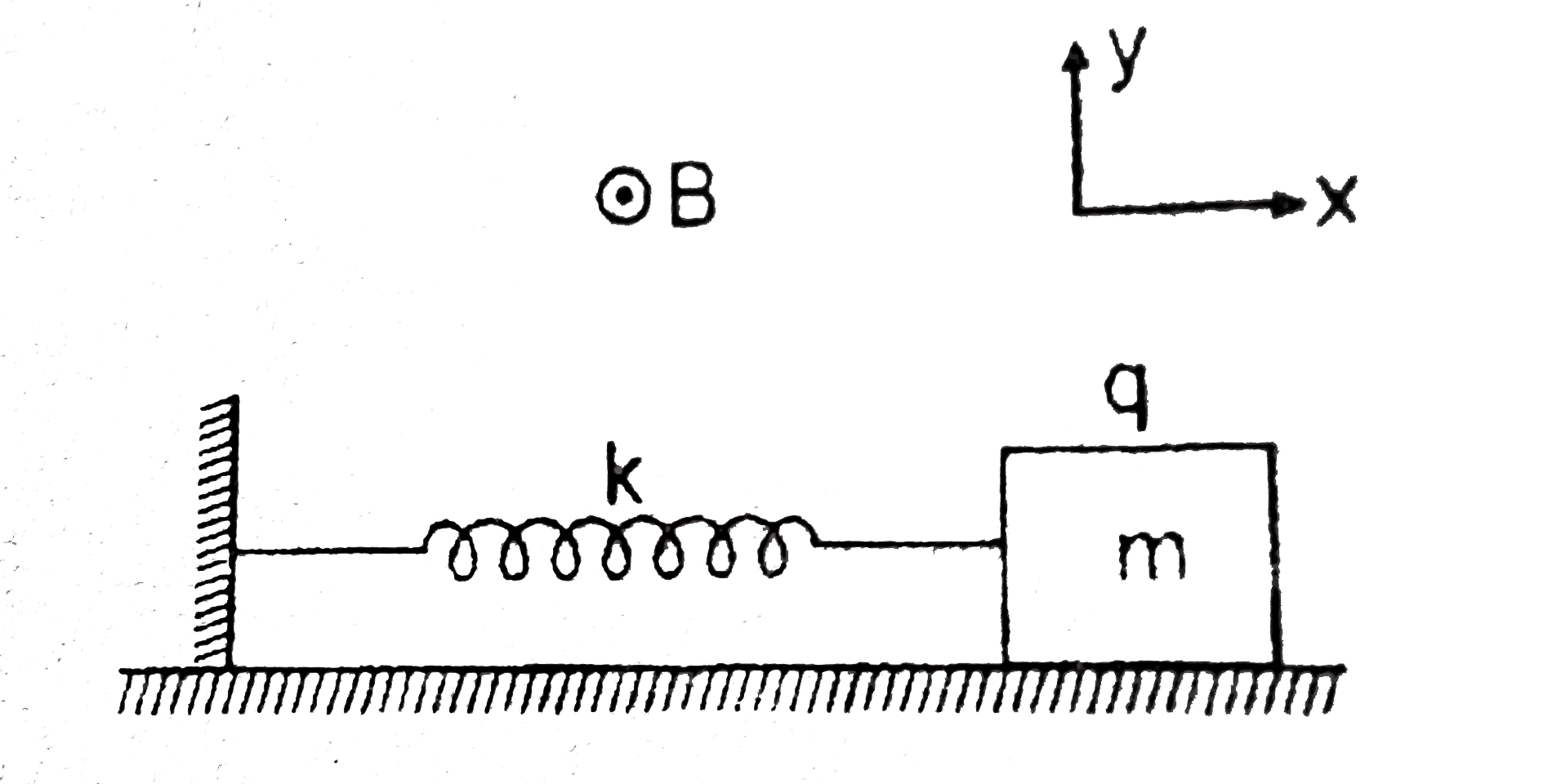
View SolutionA spring of spring constant 'K' is fixed at one end has a small block of mass m and charge q is attached at the other end. The block rests over a smooth horizontal surface. A uniform and constant magnetic field B exists normal to the plane of paper as shown in figure. An electric field →E=E0ˆi(E0 is a positive constant) is switched on at t=0 sec. The block moves on horizontal surface without ever lifting off the surface. Then the normal reaction acting on the block is :
View Solution
