Velocity time equation of a particle moving in a straight line is v=2t−4 for t≤2sandv=4−2t for t>2s. The distance travelled by the particle in the time interval from t=0 to t=4s is
8m
12m
16m
18m
The correct Answer is:A
For t≤2s,v=2t−4∴S1=ut+12at2=(−4)(2)(2)2=−8+4=−4m
At t=0,v0=−4
For t>2s,v=4−2t∴S2=ut+12at2=0+12(−2)(2)2=−4m
At=2,v0=0
∴ Distance , s=|s1|+|s2|=8m
Similar Questions
Velocity time equation of a particle moving in a straight line is v=2t−4 for t≤2s and v=4−2t for t>2.The distance travelled by the particle in the time interval from t=0 to t=4s is (Here t is in second and v in m/s)
View SolutionThe instantaneous velocity of a particle moving in a straight line is given as v=αt+βt2, where α and β are constants. The distance travelled by the particle between 1s and 2s is :
View Solution
Knowledge Check
Velocity time equation of a particle moving in a straight line is v=2t−4 for t≤2s and v=4−2t for t>2.The distance travelled by the particle in the time interval from t=0 to t=4s is (Here t is in second and v in m/s)
A12mB16mC4mD8mThe displacement of a particle moving in a straight line, is given by s=2t2+2t+4 where s is in metres and t in seconds. The acceleration of the particle is.
A2m/s2B4m/s2C6m/s2D8m/s2The velocity 'v' of a particle moving along straight line is given in terms of time t as v=3(t2−t) where t is in seconds and v is in m/s.
The distance travelled by particle from t=0 to t=2seconds is :A2mB3mC4mD6m
The velocity 'v' of a particle moving along straight line is given in terms of time t as v=3(t2−t) where t is in seconds and v is in m/s.
The distance travelled by particle from t=0 to t=2seconds is :
View Solutionvelocity-time graph of a particle moving in a straight line is as shown in figure. At time t=0, velocity of the particle is zero. Find
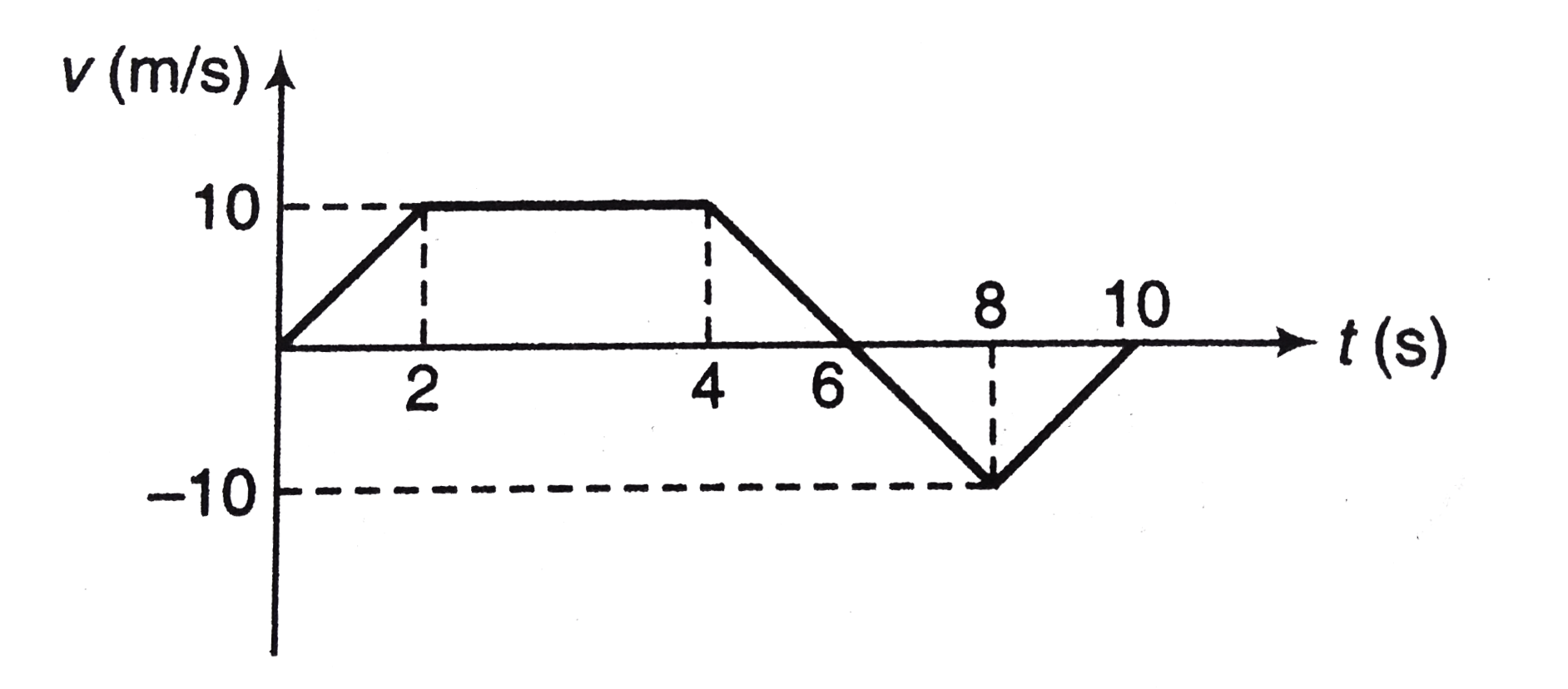
(a) average acceleration in a time interval from t=6s to t=12s,
(b) velocity of the particle at t=14s.
View SolutionA particle is moving along the x-axis such that s=6t−t2, where s in meters and t is in second. Find the displacement and distance traveled by the particle during time interval t=0 to t=5s.
View SolutionIf the distance s travelled by a particle in time t is s=asint+bcos2t, then the acceleration at t=0 is
View SolutionIfS=2t3−4t2+12t, then distance travelled during the time -interval [0,1] is
View Solution
