In the adjoining figure, in ΔABC, point D is on side BC such that, ∠BAC=∠ADC. Prove that, CA2=CB×CD.
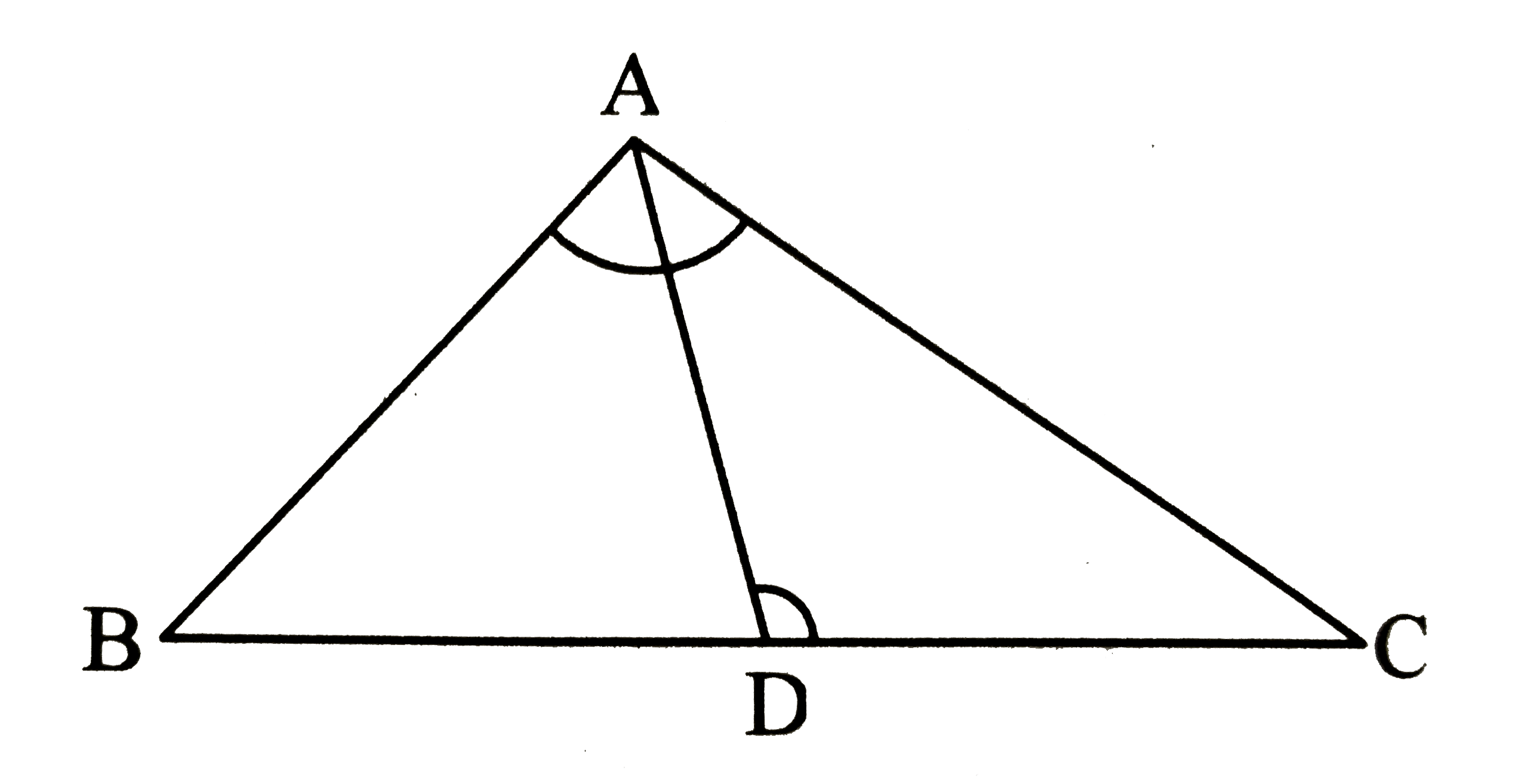 Solution in Marathi
Solution in Marathi
Step by step video & image solution for In the adjoining figure, in DeltaABC, point D is on side BC such that, angleBAC = angleADC. Prove that, CA^2 = CB xx CD. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SIMILARITY Book:BAL BHARTIChapter:SIMILARITY Exercise:PRACTICE SET 1.4
Explore 6 Videos
- SIMILARITY Book:BAL BHARTIChapter:SIMILARITY Exercise:PROBLEM SET 1
Explore 12 Videos
- SIMILARITY Book:BAL BHARTIChapter:SIMILARITY Exercise:PRACTICE SET 1.2
Explore 5 Videos
- QUADRATIC EQUATIONSBook:BAL BHARTIChapter:QUADRATIC EQUATIONSExercise:Problem Set - 2
Explore 27 Videos
- TRIGONOMETRYBook:BAL BHARTIChapter:TRIGONOMETRYExercise:Problem set 6
Explore 18 Videos
Similar Questions
Ratio of corresponding sides of two similar triangles is 3:5, then find ratio of their areas.
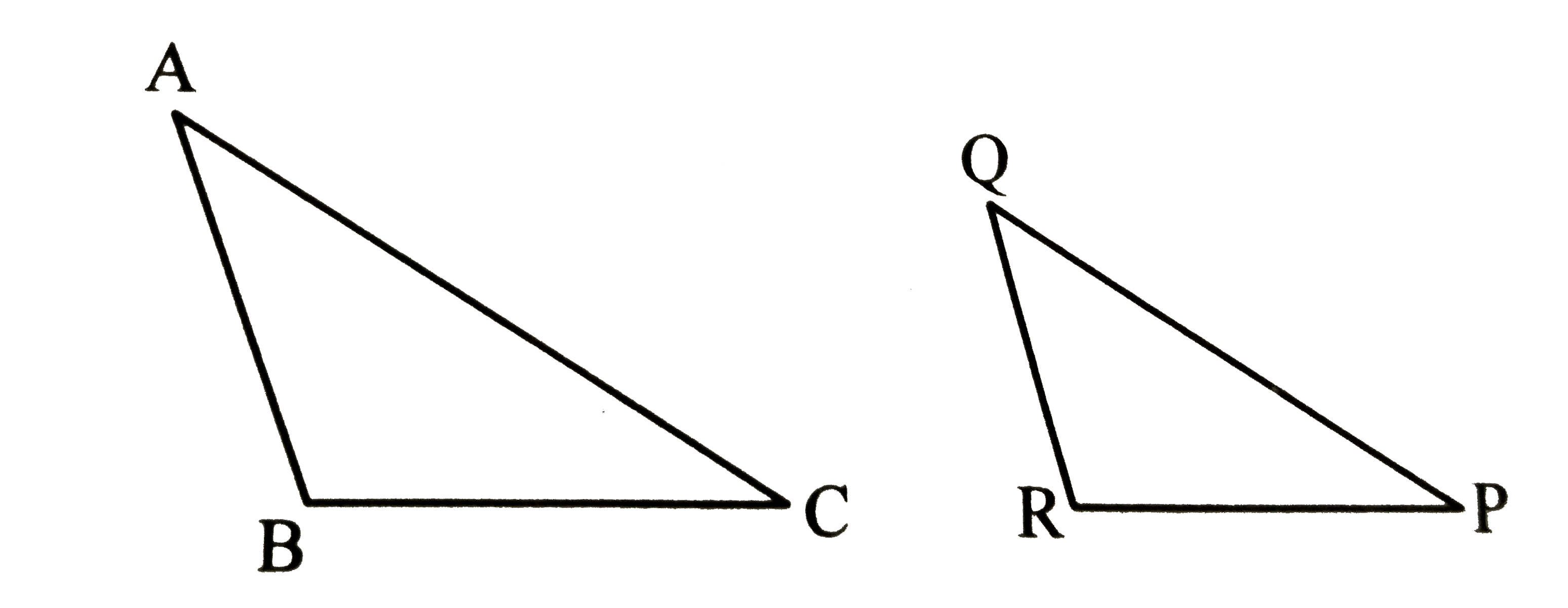
View SolutionIn ΔABCandΔPQR, in a one to one correspondence.
ABQR=BCPR=CAPQ, then
View SolutionIn ΔMNP, NQ is a biscetor of ∠N. If MN = 5, PN = 7, MQ = 2.5, then find QP.
View SolutionWhich of the following equation is quadratic?
(i) x2+2x+11=0.
View SolutionIf sinθ = 11/61 find the values of cosθ using trigonometric identity.
View SolutionHow many lines of symmetry does the above figure have ?
View SolutionDetermine the point of symmetry of a regular hexagon.
View SolutionDtermine the images of the following figure about the given line :
View SolutionMatch the following Column A to Column B

View SolutionMatch the following Column A to Column B

View SolutionMatch the following Column A to Column B

View SolutionFind the area of the figure given below.

View SolutionThe area of the shaded part in the figure given below is ______.

View SolutionThe inequation represented by the graph given below is :
View SolutionThe inequation that best describes the graph given below is _________
View SolutionThe inequation that best describes the following graph is _________
View Solution
