A block 1 of mass m1 , constrained to move along a plane inclined at angle θ to the horizontal, is connected via a massless inextensible string that passes over a massless pulley, to a second block 2 of mass m2 . Assume the coefficient of static friction between the block and the inclined plane is μk and the coefficient of kinetic friction is μk
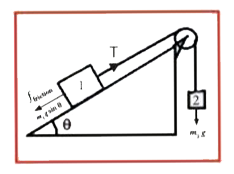
What is the relation between the masses of block 1 and block 2 such that the system just starts to slip?
By the principle of homogeneity, a/V2 is of the dimensions of pressure and b is of the dimensions of volume.
[a] = [pressure] [V2]=[ML−1T−2][L6]=[ML5T−2]
[b] =[V]=L3
Topper's Solved these Questions
- APPENDIX 1BOOK - NCERT TAMILCHAPTER - APPENDIX 1EXERCISE - SOLVED EXAMPLE UNIT-4
9 Videos
- APPENDIX 1BOOK - NCERT TAMILCHAPTER - APPENDIX 1EXERCISE - SOLVED EXAMPLE UNIT-5
14 Videos
- APPENDIX 1BOOK - NCERT TAMILCHAPTER - APPENDIX 1EXERCISE - SOLVED EXAMPLE UNIT-2
9 Videos
- GRAVITATIONBOOK - NCERT TAMILCHAPTER - GRAVITATIONEXERCISE - ADDITIONAL EXERCISES
4 Videos
Similar Questions
Knowledge Check
A block is stationary on an inclined plane If the coefficient of friction between the block and the plane is μ then
 .Aμ>tanθBf=mgsinθCf=μmgcosθDthe reaction of the ground on the block is mg cos θ
.Aμ>tanθBf=mgsinθCf=μmgcosθDthe reaction of the ground on the block is mg cos θA block rests on a rough inclined plane making an angle of 30° with the horizontal. The coefficient of static friction between the block and inclined plane is 0.8. If the frictional force on the block is 10 N, the mass of the block is
A2 kgB4 kgC1.6 kgD2.5 kgTwo block of masses m1 and m2 are connected with a massless unstretched spring and placed over a plank moving with an acceleration 'a' as shown in figure. The coefficient of friction between the blocks and platform is μ
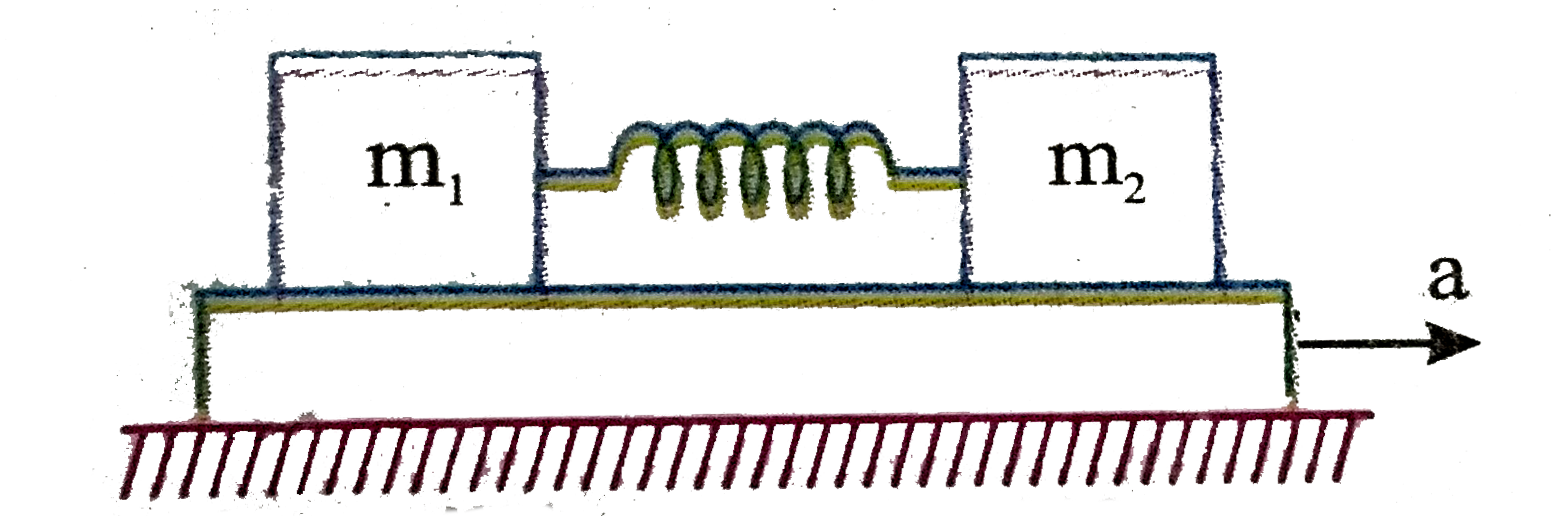 Aspring will be stretched if a>μgBspring will be commpressed if a≤μgCspring will neither be compressed nor be stretched for a<μg only.Dspring will be in its natural length under all conditions.
Aspring will be stretched if a>μgBspring will be commpressed if a≤μgCspring will neither be compressed nor be stretched for a<μg only.Dspring will be in its natural length under all conditions.
A block of mass m moves with constant speed down the inclined plane of inclination θ. Find the coefficient of kinetic friction.

View SolutionA block slides down an inclined plane that makes an angle θ with the horizontal. If the coefficient of kinetic friction is μ, find the acceleration of the block.
View SolutionCoefficient of kinetic friction and coefficient of static friction between two blocks is μk and μs respectively. In general
View SolutionCoefficient of kinetic friction and coefficient of static friction between two blocks is μk and μs respectively. In general
View SolutionA solid cylinder of mass m is wrapped with an inextensible light string and, is placed on a rough inclined plane as shown in the figure. The frictional force acting between the cylinder and the inclined plane is :
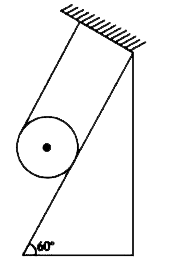 [The coefficient of static friction, μs is 0.4]
[The coefficient of static friction, μs is 0.4]
View Solution
