PQRS and PABC are two parallelograms of equal area. Prove that QC∣∣BRSolution in Punjabi
Step by step video & image solution for PQRS and PABC are two parallelograms of equal area. Prove that QC||BR by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Topper's Solved these Questions
Similar Questions
In the figure. Show that ABCD is a parallelogram.
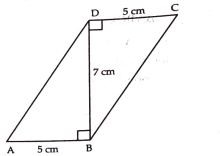 Calculate the area of ||gm ABCD.08:23View Solution
Calculate the area of ||gm ABCD.08:23View SolutionThe radius of a circle is 8 cm and the length of its chords is 12 cm. Fid the distance of the from the centre.
04:28View SolutionIn the adjoining figure, PQRS is a parallelogram. A and B are the mid-points of PQ of SR respectively. If PS= BR, then prove that quadrilateral ADBC is a reactangle.
 05:20View Solution
05:20View SolutionIn the adjoining figure, PQRS and PABC are two parallelogram of equal area. Prove that QC||BR.
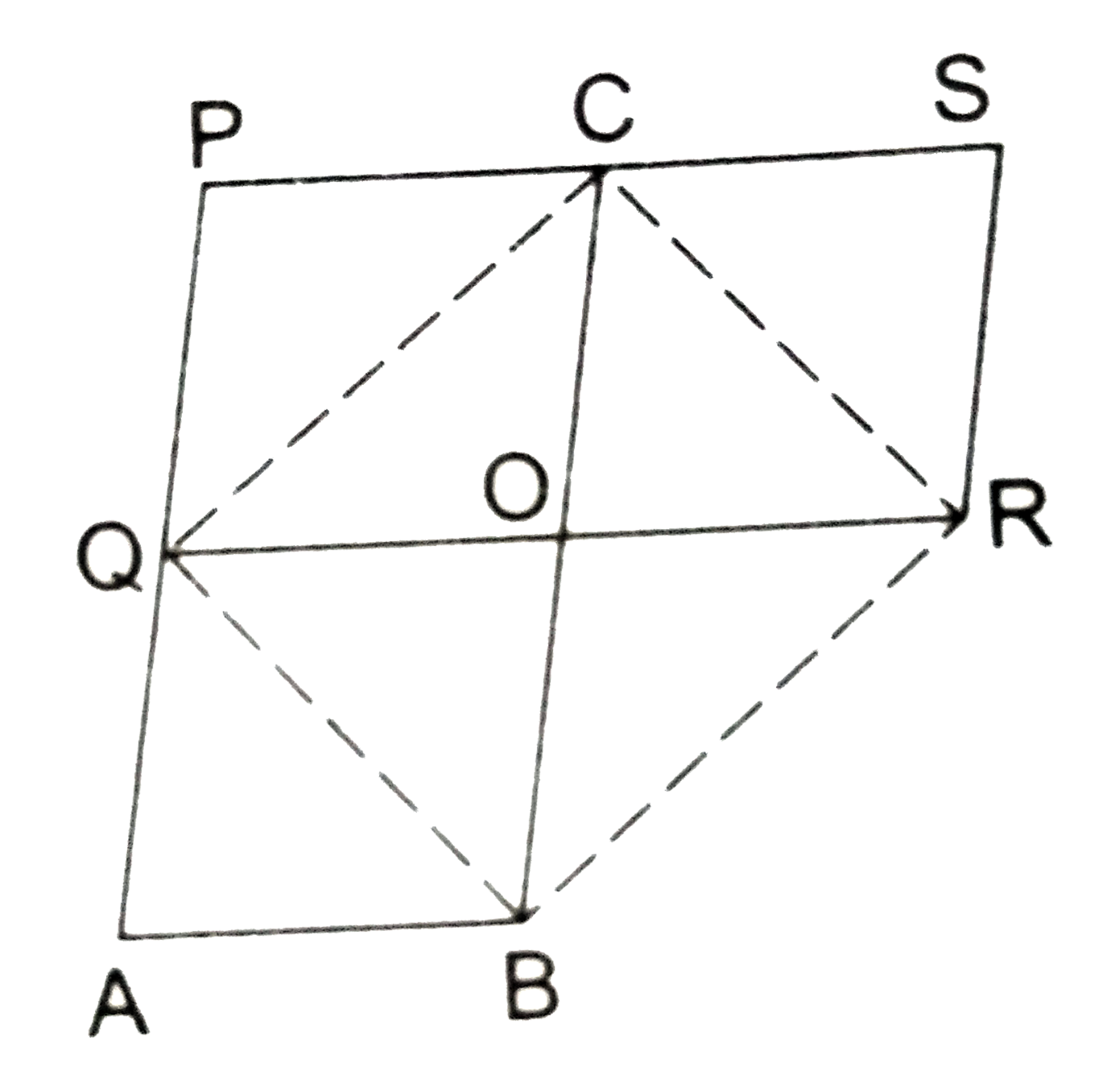 02:42View Solution
02:42View Solutionसिद्ध कीजिए कि समान्तर चतुर्भुज की सम्मुख भुजाओ के मध्य बिन्दुओं को जोड़ने वाला रेखाखण्ड समान्तर चतुर्भुज को बराबर क्षेत्रफल वाले दो समान्तर चतुर्भुजों में बांटती है।
03:47View Solutionसिद्ध कीजिए कि समान्तर चतुर्भुज के विकर्ण उस समान्तर चतुर्भुज को बराबर क्षेत्रफल के चार त्रिभुजों में बाँटते है।
04:43View Solution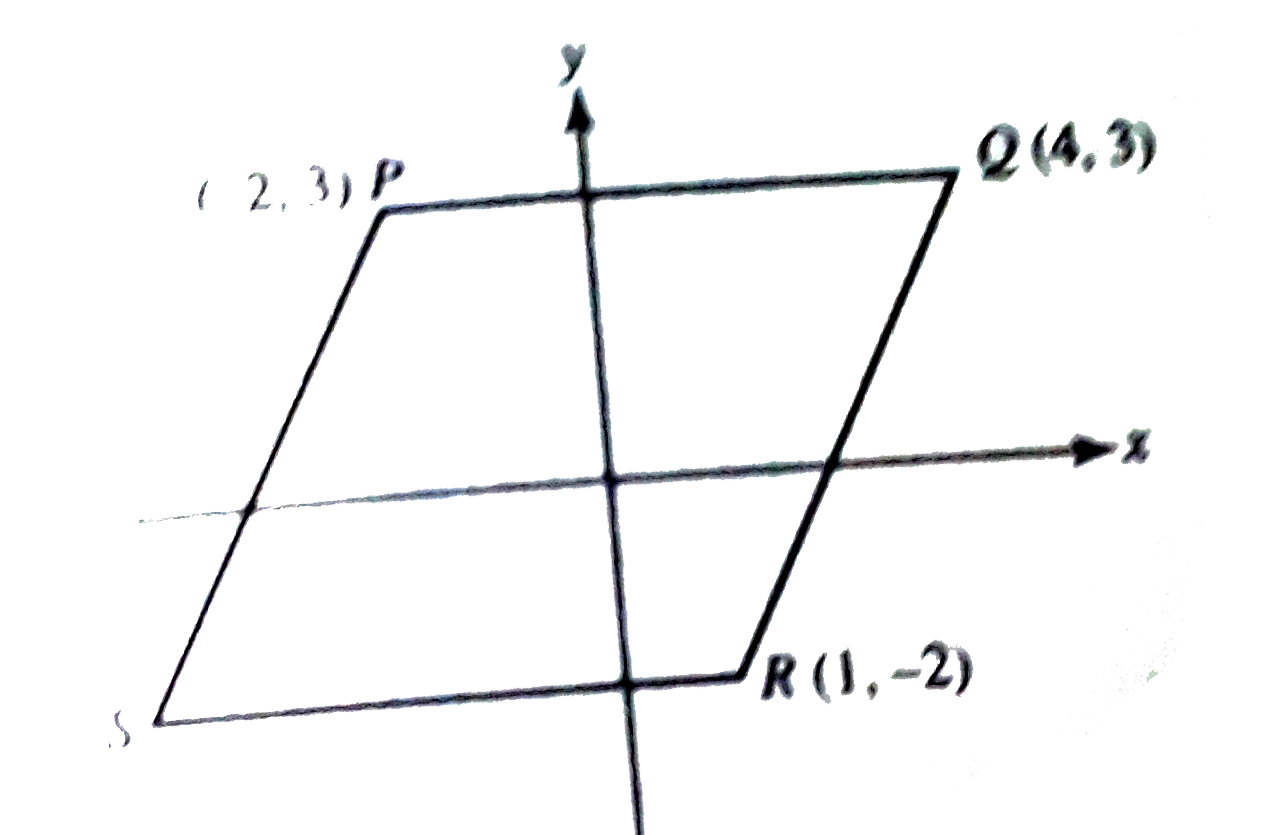
In the diagram above , PQRS is a parallelogram. What is the area of PQRS ?04:53View SolutionProve that two diagonals of a parallelogram divides it into four triangles of equal areas.
05:42View SolutionProve that if each diagonal of a quadrilateral bisects it into two triangles of equal areas, then it is a parallelogram.
View SolutionProve that twice the area of a parallelogram is equal in the area of another parallelogram formed by taking as its adjacent sides of the diagonals of the former parallelogram.
02:50View Solutionif parallelogram ABCD is congruent to parallelogram PQRS then (area(ABCD))/(area(PQRS)) =
04:54View SolutionPQRS and ABRS are two parallelograms. X is a point on the shoulder of BR (PQRS) y || = (ABRS) y ||
03:15View SolutionPQRS and ABRS are two parallelograms. X is a point on the shoulder of BR (/_\AXS) Y || = 1/2(PQRS) Why ||
06:32View SolutionProve that A diagonal of a parallelogram divides it into two triangles of equal area.
09:03View Solutionprove that the line segment joining the midpoints of two opposite sides of a parallelogram divides the parallelogram into two parallelograms with equal area.
03:10View SolutionIn the adjoining figure, PQRS is a parallelogram. A and B are the mid-points of PQ of SR respectively. If PS= BR, then prove that quadrilateral ADBC is a reactangle.
 00:53View Solution
00:53View Solution

