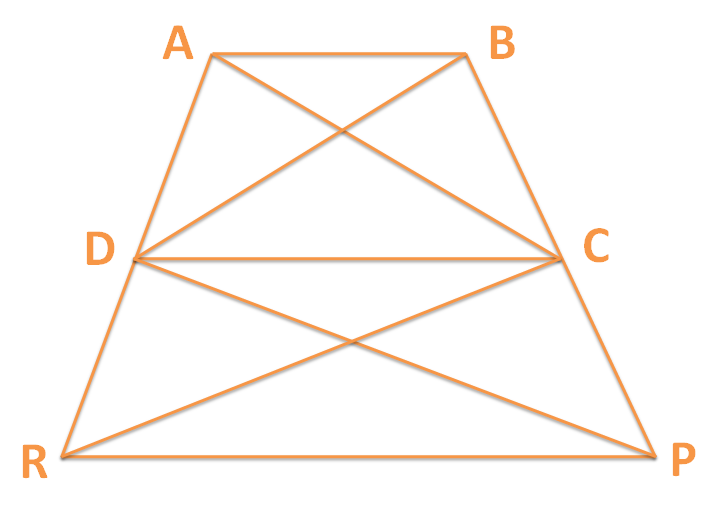
ar(DRC) = ar(DPC) and ar(BDP) = ar(ARC). Show that both the quadrilaterals ABCD and DCPR are trapeziums.Solution in Punjabi
ar(DRC) = ar(DPC) and ar(BDP) = ar(ARC). Show that both the quadrilaterals ABCD and DCPR are trapeziums.Solution in Punjabi
Step by step video & image solution for ar(DRC) = ar(DPC) and ar(BDP) = ar(ARC). Show that both the quadrilaterals ABCD and DCPR are trapeziums. by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
In the figure. Show that ABCD is a parallelogram.
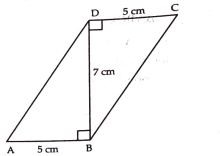 Calculate the area of ||gm ABCD.
Calculate the area of ||gm ABCD.
View SolutionThe radius of a circle is 8 cm and the length of its chords is 12 cm. Fid the distance of the from the centre.
View SolutionDetermine the point of symmetry of a regular hexagon.
View SolutionMatch the following Column A to Column B

View SolutionMatch the following Column A to Column B

View SolutionMatch the following Column A to Column B

View SolutionThe inequation represented by the graph given below is :
View SolutionThe inequation that best describes the graph given below is _________
View SolutionThe inequation that best describes the following graph is _________
View Solutionआकृति में ar(DRC)=ar(DPC) है और ar(BDP)=ar(ARC) है । दर्शाइए की दोनों चतुर्भुज ABCD और DCPR समलंब है ।
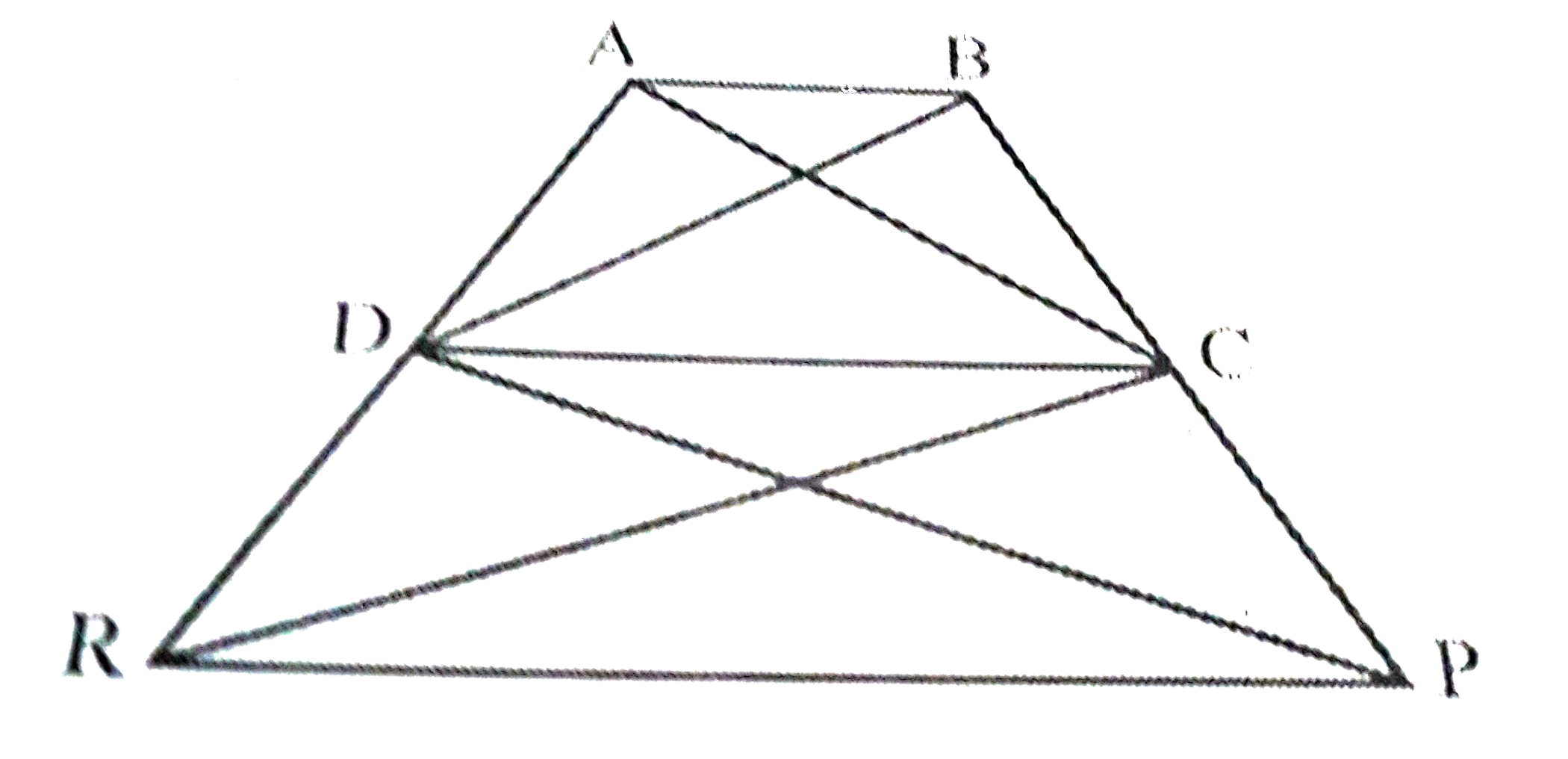
View Solutionआकृति में, क्षेत्रफल (DRC) = क्षेत्रफल (DPC) है और क्षेत्रफल (BDP) = क्षेत्रफल (ARC) है। दर्शाइए कि दोनों चतुर्भुज ABCD और DCPR समलंब हैं।
View SolutionIn the given figure , ar (DRC) = ar ( DPC) and ar(BDP) = ar(ARC) . Show that both the quadrillaterals ABCD and DCPR are trapeziums.
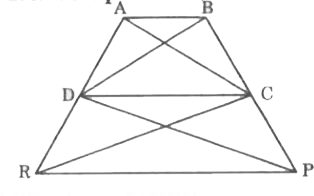
View SolutionProve that V (DRC) = V (DPC) and V (BDP) = V (ARC) in Figure 11.29 but that ABCD and DCPR are quadrilateral.
View SolutionIn the figure, if ar ΔRAS=arΔRBSand[ar(ΔQRB)=ar(ΔPAS) then show that both the quadrilaterals PQSR and RSBA are trapeziums.
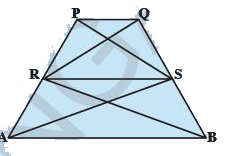
View SolutionIn the figure, if ar (DeltaRAS)_ = ar (DeltaRBS) and [ar (DeltaQRB) = ar(DeltaPAS) then show that both the quadrilaterals PQSR and RSBA are trapeziums.
View SolutionIn the figure, if ar ΔRAS=arΔRBSand[ar(ΔQRB)=ar(ΔPAS) then show that both the quadrilaterals PQSR and RSBA are trapeziums.

View Solution