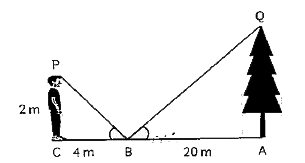
Magnification of figures is a process of enlarging the apparent size, not the physical size, of something. The enlarged figure is quantified by a calculated number. If two triangles are similar then their corresponding sides are in the same ratio. Basically a bigger triangle is a enlargement of the smaller triangle. This basic rule of similar triangles is applicable in solving many real life problems like relating the height and shadow length of various objects at a particular instant in a day.
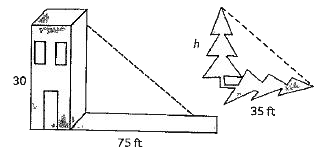
See the figure below and evaluate the height of the tree.
If the shadows of a lamp-post and a at the same time of a days are 18 ft. and 6 ft. respectively then what is the height of the lamp-post.
25 m
40 m
20 m
10 m
The correct Answer is:D
Step by step video, text & image solution for Magnification of figures is a process of enlarging the apparent size, not the physical size, of something. The enlarged figure is quantified by a calculated number. If two triangles are similar then their corresponding sides are in the same ratio. Basically a bigger triangle is a enlargement of the smaller triangle. This basic rule of similar triangles is applicable in solving many real life problems like relating the height and shadow length of various objects at a particular instant in a day. See the figure below and evaluate the height of the tree. If the shadows of a lamp-post and a at the same time of a days are 18 ft. and 6 ft. respectively then what is the height of the lamp-post. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
- BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 5EXERCISE - PART - A (SECTION - I)21videos
SAMPLE PAPER 5
- BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 5EXERCISE - PART - A (SECTION - II)20videos
SAMPLE PAPER 5
- BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 5EXERCISE - SECTION - B20videos
SAMPLE PAPER 5
- BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 4EXERCISE - PART-B(SECTION-V)5videos
SAMPLE PAPER 4
- BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 6EXERCISE - SECTION- C10videos
SAMPLE PAPER 6
Similar Questions
Knowledge Check
- Question 1 - Select One
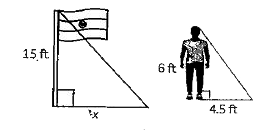
Magnification of figures is a process of enlarging the apparent size, not the physical size, of something. The enlarged figure is quantified by a calculated number. If two triangles are similar then their corresponding sides are in the same ratio. Basically a bigger triangle is a enlargement of the smaller triangle. This basic rule of similar triangles is applicable in solving many real life problems like relating the height and shadow length of various objects at a particular instant in a day.
Evaluate for x
A10.5 ftB11.25 ftC12 ftD9 ft - Question 2 - Select One
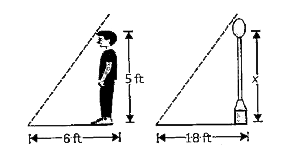
Magnification of figures is a process of enlarging the apparent size, not the physical size, of something. The enlarged figure is quantified by a calculated number. If two triangles are similar then their corresponding sides are in the same ratio. Basically a bigger triangle is a enlargement of the smaller triangle. This basic rule of similar triangles is applicable in solving many real life problems like relating the height and shadow length of various objects at a particular instant in a day.
Evaluate x, by considering the figure below.
A15 ftB10 ftC12 ftD18 ft - Question 3 - Select One
Magnification of figures is a process of enlarging the apparent size, not the physical size, of something. The enlarged figure is quantified by a calculated number. If two triangles are similar then their corresponding sides are in the same ratio. Basically a bigger triangle is a enlargement of the smaller triangle. This basic rule of similar triangles is applicable in solving many real life problems like relating the height and shadow length of various objects at a particular instant in a day.
Evaluate x, by considering the figure below.
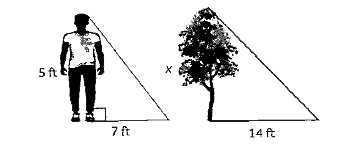A12 ftB15 ftC10 ftD7 ft
If two triangles are similar; prove that the ratio of the corresponding sides is same as the ratio of corresponding medians.
View SolutionIf two triangles are similar; prove that the ratio of corresponding sides is equal to the ratio of corresponding altitudes.
View SolutionTheorem 6.3 : If in two triangles, corresponding angles are equal, then their corresponding sides are in the same ratio (or proportion) and hence the two triangles are similar.
View SolutionMagnification of figures is a process of enlarging the apparent size, not the physical size, of something. The enlarged figure is quantified by a calculated number. If two triangles are similar then their corresponding sides are in the same ratio. Basically a bigger triangle is a enlargement of the smaller triangle. This basic rule of similar triangles is applicable in solving many real life problems like relating the height and shadow length of various objects at a particular instant in a day.
What is the height of the tree i.e. h ?
View SolutionSides of two similar triangles are in the ratio 4:9. Areas of these triangles are in the ratio
View Solution