When the remainder obtained by dividing 60606 by 106 is subtracted from the remainder obtained on dividing 70707 by 107, then the resultant number is
7
8
5
8
The correct Answer is:A
To solve the problem, we need to find the remainders of two divisions and then subtract one from the other. Here’s a step-by-step breakdown of the solution:
Step 1: Find the remainder of 60606 when divided by 106.
To do this, we perform the division:
1. Divide 60606 by 106:
- Calculate how many times 106 fits into 60606.
- 106 goes into 60606 approximately 572 times (since 106×572=60632 which is too high, we try 571).
- 106×571=60526.
2. Calculate the remainder:
- Subtract 60526 from 60606:
60606−60526=80
So, the remainder when 60606 is divided by 106 is 80.
Step 2: Find the remainder of 70707 when divided by 107.
Now, we perform the division for the second number:
1. Divide 70707 by 107:
- Calculate how many times 107 fits into 70707.
- 107 goes into 70707 approximately 660 times (since 107×660=70620).
2. Calculate the remainder:
- Subtract 70620 from 70707:
70707−70620=87
So, the remainder when 70707 is divided by 107 is 87.
Step 3: Subtract the two remainders.
Now, we subtract the remainder obtained from the first division from the remainder obtained from the second division:
87−80=7
Conclusion:
The resultant number after performing the operations is 7.
---
To do this, we perform the division:
- Calculate how many times 106 fits into 60606.
- 106 goes into 60606 approximately 572 times (since 106×572=60632 which is too high, we try 571).
- 106×571=60526.
- Subtract 60526 from 60606:
60606−60526=80
So, the remainder when 60606 is divided by 106 is 80.
Now, we perform the division for the second number:
- Calculate how many times 107 fits into 70707.
- 107 goes into 70707 approximately 660 times (since 107×660=70620).
2. Calculate the remainder:
- Subtract 70620 from 70707:
70707−70620=87
So, the remainder when 70707 is divided by 107 is 87.
Now, we subtract the remainder obtained from the first division from the remainder obtained from the second division:
The resultant number after performing the operations is 7.
Similar Questions
Knowledge Check
- Question 1 - Select One
When the remainder obtained on dividing 80808 by 108 is divided by the remainder obtained on dividing 90909 by 109, then the quotient is
A8B12C3D6 - Question 2 - Select One
The remainder obtained when 5125 is divided by 13 is
A3B7C12D11 - Question 3 - Select One
When
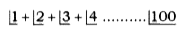 , is divided by 5, the remainder obtained is ?A0B1C2D3
, is divided by 5, the remainder obtained is ?A0B1C2D3
When
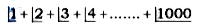 is divided by 12, the remainder obtained is? View Solution
is divided by 12, the remainder obtained is? View SolutionWhen
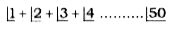 , is divided by 12, the remainder obtained is ?View Solution
, is divided by 12, the remainder obtained is ?View SolutionWhen 221 is divided by 9, the remainder obtained is ?
View SolutionWhen 1177 is divided by 7, the remainder obtained is ?
View SolutionWhen 740 is divided by 400, the remainder obtained is ?
View Solution


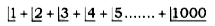 is divided by 10, the remaindeer obtained is ?
is divided by 10, the remaindeer obtained is ?