The length of the tangent drawn from a point 8 cm away from the centre of a circle of radius 6 cm is .
cm
cm
cm
cm
To find the length of the tangent drawn from a point 8 cm away from the center of a circle with a radius of 6 cm, we can use the Pythagorean theorem. Here’s a step-by-step solution:
Step 1: Identify the elements of the problem
- Let O be the center of the circle.
- Let P be the point outside the circle from which the tangent is drawn.
- Let A be the point of tangency on the circle.
- The distance OP (from the center O to point P) is 8 cm.
- The radius OA (from the center O to point A) is 6 cm.
Step 2: Draw the right triangle
Since the radius OA is perpendicular to the tangent PA at point A, we can form a right triangle OAP where:
- OA is one leg (radius) = 6 cm
- PA is the other leg (length of the tangent) which we need to find.
- OP is the hypotenuse = 8 cm
Step 3: Apply the Pythagorean theorem
According to the Pythagorean theorem:
OP2=OA2+PA2
Step 4: Substitute the known values
Substituting the known values into the equation:
82=62+PA2
Step 5: Calculate the squares
Calculating the squares:
64=36+PA2
Step 6: Rearrange the equation to solve for PA^2
Rearranging gives:
PA2=64−36
PA2=28
Step 7: Take the square root
Now, take the square root to find PA:
PA=√28
PA=√4×7
PA=2√7
Step 8: Approximate the value
To get a decimal approximation:
PA≈2×2.64575≈5.2915 cm
Final Answer
The length of the tangent drawn from point P to the circle is approximately 5.29 cm.
---
- Let O be the center of the circle.
- Let P be the point outside the circle from which the tangent is drawn.
- Let A be the point of tangency on the circle.
- The distance OP (from the center O to point P) is 8 cm.
- The radius OA (from the center O to point A) is 6 cm.
Since the radius OA is perpendicular to the tangent PA at point A, we can form a right triangle OAP where:
- OA is one leg (radius) = 6 cm
- PA is the other leg (length of the tangent) which we need to find.
- OP is the hypotenuse = 8 cm
According to the Pythagorean theorem:
OP2=OA2+PA2
Substituting the known values into the equation:
82=62+PA2
Calculating the squares:
64=36+PA2
Rearranging gives:
PA2=64−36
PA2=28
Now, take the square root to find PA:
PA=√28
PA=√4×7
PA=2√7
To get a decimal approximation:
PA≈2×2.64575≈5.2915 cm
The length of the tangent drawn from point P to the circle is approximately 5.29 cm.
Similar Questions
The length of the tangent drawn from a point 8cm away from the centre of a circle of radius 6cm is √7cm (b) 2√7cm (c) 10cm (d) 5cm
View SolutionFind the length of the tangent drawn from a point whose distance from the centre of a circle is 25 cm. Given that the radius of the circle is 7 cm.
View Solution
Knowledge Check
The length of a tangent drawn from a point 10 cm away from the centre of the circle of radius 5 cm is
A5 cmB5√3 cmC2√3 cmD√15 cmThe length of the tangent drawn to a circle of radius 4 cm from a point 5 cm away from the centre of the circle is
A3cmB4√2 cmC5√2cmD3√2 cm
The length of a tangent from a point A at distance 5 cm from the centre of the circle is 4 cm. Find the radius of the circle.
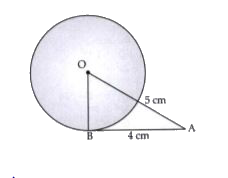
View SolutionThe length of a tangent from a point A at distance 5 cm from the centre of the circle is 4 cm . Find the radius of the circle.
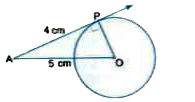
View SolutionThe length of a tangent from a point A at distance 5 cm from the centre of the circle is 4 cm. Find the radius of the circle.
View SolutionThe length of tangent from a point A at a distance of 5 cm from the centre of the circle is 4 cm. What will be the radius of the circle ?
View SolutionThe length of tangent from a point A at a distance of 12 cm from the centre of the circle is 9 cm. What is the radius of the circle ?
View SolutionThe length of the tangent to a circle from a point P, which is 25 cm away from the centre, is 24 cm. What is the radius of the circle.
View Solution
