In figure , is shown a sector OAP of a circle with centre O , containing ∠θ . AB is perpendicular to the radius OA and meets OP produced at B . Prove that the perimeter of shaded region is r[tanθ+secθ−1−πθ180]
![In figure , is shown a sector OAP of a circle with centre O , containing angletheta . AB is perpendicular to the radius OA and meets OP produced at B . Prove that the perimeter of shaded region is r [ tan theta + sec theta - 1- (pi theta)/(180)]](https://d10lpgp6xz60nq.cloudfront.net/physics_images/OSW_CBSE_OFA_MAT_X_DIK_QES_E06_032_Q01.png)
Step by step video & image solution for In figure , is shown a sector OAP of a circle with centre O , containing angletheta . AB is perpendicular to the radius OA and meets OP produced at B . Prove that the perimeter of shaded region is r [ tan theta + sec theta - 1- (pi theta)/(180)] by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- DIKSHA QUESTIONS BOOK - OSWAL PUBLICATIONCHAPTER - DIKSHA QUESTIONS EXERCISE - UNIT -VI: Area Related to Circle (Surface Areas and volumes) (MULTIPLE CHOICE QUESTIONS)
3 Videos
- DIKSHA QUESTIONS BOOK - OSWAL PUBLICATIONCHAPTER - DIKSHA QUESTIONS EXERCISE - UNIT -VI: Area Related to Circle (Surface Areas and volumes) (VERY SHORT ANSWER TYPE QUESTIONS)
4 Videos
- DIKSHA QUESTIONS BOOK - OSWAL PUBLICATIONCHAPTER - DIKSHA QUESTIONS EXERCISE - UNIT -VI: Area Related to Circle (Areas Related to Circles ) ( SHORT ANSWER TYPE QUESTIONS )
17 Videos
- COORDINATE GEOMETRYBOOK - OSWAL PUBLICATIONCHAPTER - COORDINATE GEOMETRYEXERCISE - SELF ASSESSMENT
20 Videos
- INTRODUCTION TO TRIGONOMETRY BOOK - OSWAL PUBLICATIONCHAPTER - INTRODUCTION TO TRIGONOMETRY EXERCISE - Self - Assessment
15 Videos
Similar Questions
In the given figure, is shown a sector OAP of a circle with centre 0, containing ∠θ. AB is perpendicular to the radius OA and meets OP produced at B. Prove that the perimeter of shaded region is r[tanθ+secθ+πθ180−1]
View SolutionIn Fig. 4, a sector OAP of a circle with centre O, containing ∠0. AB is perpendicular to the radius OA and meets OP produced at B. Prove that the perimeter of shaded region is r.
[tan 0 + sec 0 + π0180∘−1]
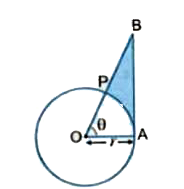
View SolutionFigure shows a sector of a circle, centre O, containing an angle θ. Prove that (i) Perimeter of the shaded region is r(tanθ+secθ+πθ180−1) (ii) Area of shaded region is r22(tanθ−πθ180)
View SolutionFig. 15.17, shows a sector of a circle, centre O , containing an angle θo , prove that: (FIGURE) (i) Perimeter of the shaded region is r (tanθ+secθ+πθ180−1) (ii) Area of the shaded region is r22 (tanθ−πθ180)
View SolutionFigure shows a sector of a circle of radius r containing an angle θ∘ The area of the sector is Acm2 and the perimeter is 50cm. Prove that θ=360π(25r−1) and A=25r−r2
View SolutionIn figure , AB and CD are two diameters of a circle ( with centre O) perpendicular to each other and OD is the diameter of the smaller circle . If OA = 7 cm , find the area of the shaded region.
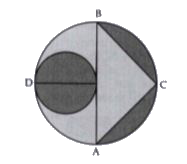
View SolutionIf Figure - 4, AB and CD are two diameter of a circle (with centre O) perpendicular to each other and OD is the diameter of the smaller circle. IF OA = 7 cm, then find the area of the shaded region.

View SolutionIn the given figure, AB and CD are two diameters of circles ( with centre O) Perpendicular to each other and OD is the diameter of the smallest circle. If OA = 7cm, Find the area of the shaded region.
View SolutionIn the given figure, AB and PQ are perpendicular diameters of the circle whose centre is O and radius OA = 7 cm. Find the area of the shade region.

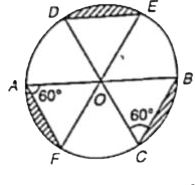
View SolutionIn the adjoining figure O is the centre of the circle with radius 'r' AB, CD and EF are the diameters of the circle. ∠OAF=∠OCB=60∘. What is the area of the shaded region?
View Solution
