A block of mass m is connected to two indentical springs of spring constant k which are in turn connected to fixed supports as shown in the figure. Find the time period for small oscillations of the block.

2π√mk
2π√m2k
2π√2mk
π√m2k
The correct Answer is:B
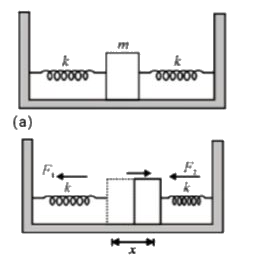
(b) Let the mass m be displaced by a small distance x to the right from its mean position as shown in figure.
(b) Due to it the spring on the left side gets starched by a length x while that on the right side gets compressed by the same length. The forces acting on the mass are F1=kx towards left hand are F2=kx towards left hand are
The net forces acting on the mass is, F=F1+F2=−2kx
Here, F∝x and −ve sign shows that force is toward the mean position, therefore the motion executed by the particle is simple harmonic. Its acceleration is: F=F1+F2=−2kx
The standard equation of SHM is:
a=−ω2x
Comparing (i) and (ii), we get:
ω2=2km or ω=√2km
Time period, T=2πω=2π√m2k
Topper's Solved these Questions
Similar Questions
Two identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in the figure. The time period of oscillation is

View SolutionTwo identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in the figure. The time period of oscillation is

View Solution
Knowledge Check
A spring of spring constant K is cut equal parts of which t part are places in particle and connected will mass m shown in figure The time period of oscilation motion of mass m is
AT=2π√mnrkBT=2π√nrmKCT=2π√rmnKDT=2π√nmrKTwo springs of force constant k and 2k are connected to a mass shown in figure the frequency of oscillation of the mass is
 A12π√mkB12π√2kmC12π√3kmD12π√mk
A12π√mkB12π√2kmC12π√3kmD12π√mkTwo springs of force constant k and 2k are connected to a mass shown in figure the frequency of oscillation of the mass is
 A12π√mkB12π√2kmC12π√3kmD12π√mk
A12π√mkB12π√2kmC12π√3kmD12π√mk
A block of mass m is connected to another .block of mass M by a massless spring of spring constant k. A constant force f starts action as shown in figure, then:

View SolutionA block of mass m is connected to another .block of mass M by a massless spring of spring constant k. A constant force f starts action as shown in figure, then:

View SolutionTwo spring of spring constants k1 and k2 ar joined and are connected to a mass m as shown in the figure. Calculate the frequency of oscillation of mass m.

View SolutionA block of mass m is connected with two ideal pullies and a massless spring of spring constant K as shown in figure. The block is slightly displaced from its equilibrium position. If the time period of oscillation is μπ√mK. Then find the value of μ.

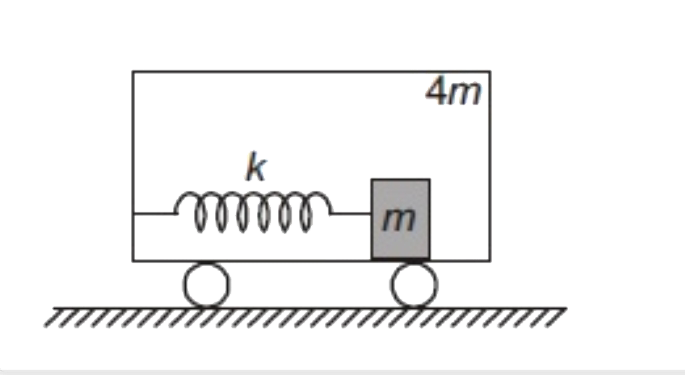
View SolutionA block of mass m is attached to a cart of mass 4m through spring of spring constant k as shown in the figure. Friction is absent everywhere. The time period of oscillations of the system, when spring is compressed and then released, is
View Solution
