Consider two particles of equal mass m. If one particle is stationary while the other collides with this particle obliquely with velocity v. Now, what is the angle between the velocity vectors of the two particles after the collision?
45∘
30∘
90∘
none of these
The correct Answer is:C
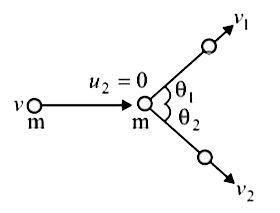
Given that mass of each particle = m
initial velocity of one moving particle = v
initial velocity of other particle = 0 (because it is stationary)
Let after collision,
One particle moves with velocity v1 with angle θ1 w.r.t. -axis, and other particle moves with velocity v2 with angel θ2 w.r.t. x axis.
Now, by momentum conservation in x-direction (initial momentum) along x-axis.
= (final momentum) along y-axis (pi)x−axis=(pf)x−axis
⇒mv+m(0)=mv1cosθ1+mv2cosθ2
⇒v=v1cosθ1+v2cosθ2...(1)
Now, by momentum conservation in y-direction
(pi)y-axis=(pf)y-axis
⇒m(0)+m(0)=mv1sin
θ1–mv2sinθ2
⇒0=v1sinθ1–v2sinθ2...(2)
and by law of conservation of kinetic energy
(Kinetic energy)before collision=(Kinetic energy)after collision
⇒12mv2+12m(0)2=12mv21+12mv2
⇒v2=v21+v22 ...(3)
Addition of square of eq. (1) and eq. (2)
⇒v2+02=(v1cosθ1+v2cosθ2)2+(v1sinθ1−v2sinθ2)2
⇒v2=v21cosθ21+v22cosθ22+2v1v2cosθ1cosθ2+v21sinθ21+v22sinθ22−2v1v2sinθ1sinθ2
⇒v2=v21(cosθ21+sinθ22)+v22(cosθ22+sinθ22)
2v1v2(cosθ1cosθ2−sinθ2sinθ2)
⇒v2=v21+v22+2v1v2
(cosθ1cosθ2−sinθ1sinθ2)
Using eq.(3)
⇒v21+v22=v21+v22+2v1v2(cosθ1cosθ2−sinθ1sinθ2)
⇒2v1v2cos(θ1+θ2)=0
⇒cos(θ1+θ2)=0
⇒θ1+θ2=cos−1(0)=90
So, angle between the velocity vectors of the two particles after the collision is 90∘ .
Topper's Solved these Questions
Similar Questions
A particle of mass m and veocity →v collides elastically with a stationary particle of mass m Calculate the angle between velocity vector of the two partiicless after the collision.
View SolutionA particle of mass m1 moves with velocity v1 and collides with another particle at rest of equal mass. The velocity of the second particle after the elastic collision is
View Solution
Knowledge Check
The velocity vector of a stationary particle is __________
Azero vectorBvector with magnitude of velocity vectorCscalarDscalar with magnitude of velocity vector
A particle of mass m strikes another particle of same at rest. Find the angle between velocities of particles after the collision , if the collision is elastic.
View SolutionA particle of mass m moving with a constant velocity v hits another identical stationary particle. If coefficient of restitution is e then ratio of velocities of two particles after collision is
View SolutionA particle of mass m moving with a constant velocity v hits another identical stationary particle. If coefficient of restitution is e then ratio of velocities of two particles after collision is
View SolutionParticle 1 with mass m and velocity v and particle 2 with mass 2m and velocity -2v are moving toward each other along an x axis when they undergo a one-dimensional elastic collision. After the collision, what are the velocities of (a) particle 1 and (b) particle 2? What is the velocity of the center of mass of the two-particle system (c) before and (d) after the collision?
View SolutionTwo particles of mass 2m and m moving with speed v and 2v respectively pependicular to each other collides perfectly inelastically, then

Find the angle with the horizontal to which the particles move after collision.
View SolutionTwo particles of mass 2m and m moving with speed v and 2v respectively pependicular to each other collides perfectly inelastically, then

Find the angle with the horizontal to which the particles move after collision.
View SolutionA particle of mass m moving with velocity v0 collides with sphere of same mass at rest , as shown. If the surface of contact is smooth and the collision is elastic, find the velocities of particle and sphere after the collision.
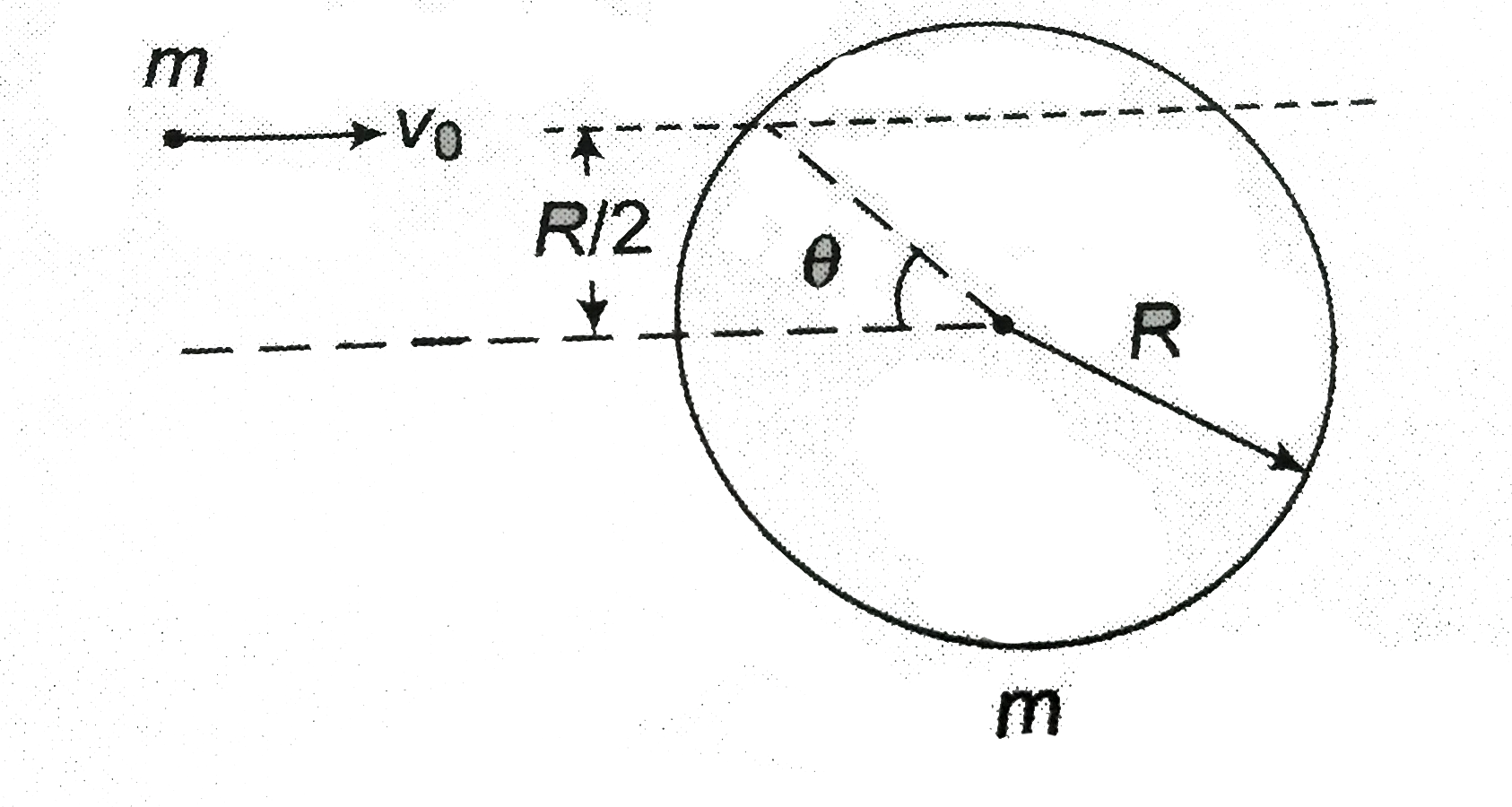
View Solution
