In a special arrangement of Young's double - slits d is twice the distance between the screen and the slits D, i.e. d = 2D. For the setup, the value of D such that the first minima on the screen fall at a distance D from the centre O is found to be λN, where λ is the wavelength of light used. What is the value of N ? [Take √5=2.24]
The correct Answer is:2.48
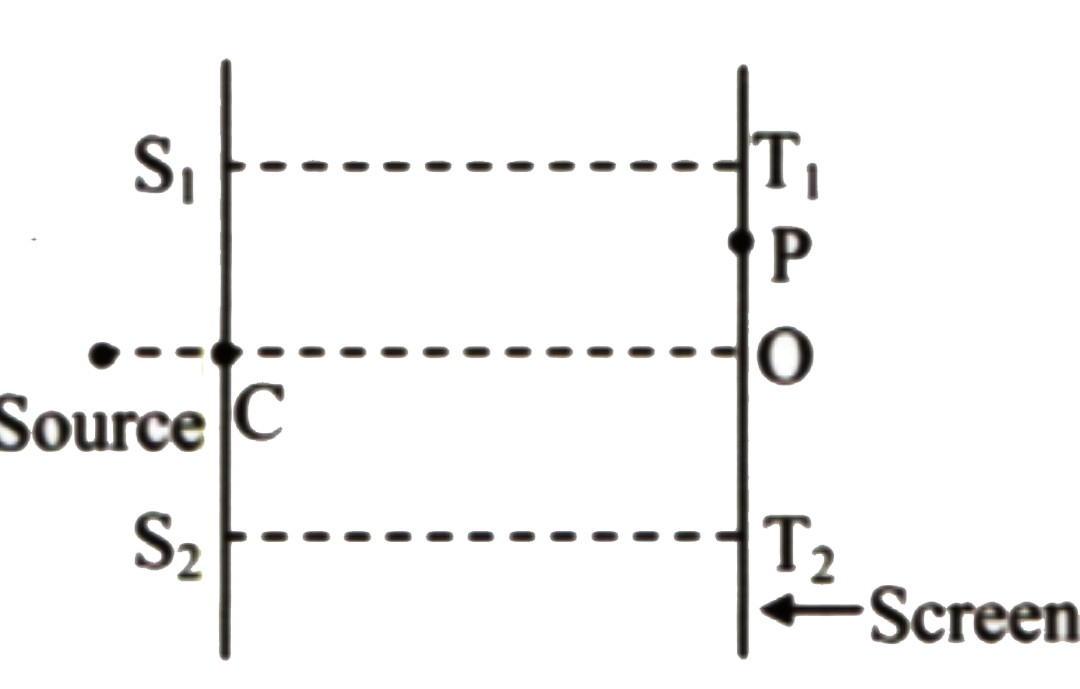
From diagram as provided in question,
OP =x
CO =D
S1C=S2C=D
T1P=T1O−OP=D−x
T2P=T2O+OP=D+x
Now S1p=√(S1T1)2+(T1P)2
=√D2+(D−x)2
S2P=√(S2T2)2+(T2P)2
=√D2+(D+x)2
For fist minimum to occur, Path difference
S2P−S1P=λ2
⇒√D2+(D+x)2
−√D2+(D−x)2=λ2
The first minimum falls at a distance D from the centre, i.e., x = D
[D2+4D2]12−D=λ2
∴D(√5−1)=λ2
⇒D=λ2(2.24−1)=λ2.48
Hence, the required value of N = 2. 48
Topper's Solved these Questions
Similar Questions
In a special arrangement of Young's double - slits d is twice the distance between the screen and the slits D, i.e. d = 2D. For the setup, the value of D such that the first minima on the screen fall at a distance D from the centre O is found to be λN, where λ is the wavelength of light used. What is the value of N ? [Take √5=2.24]

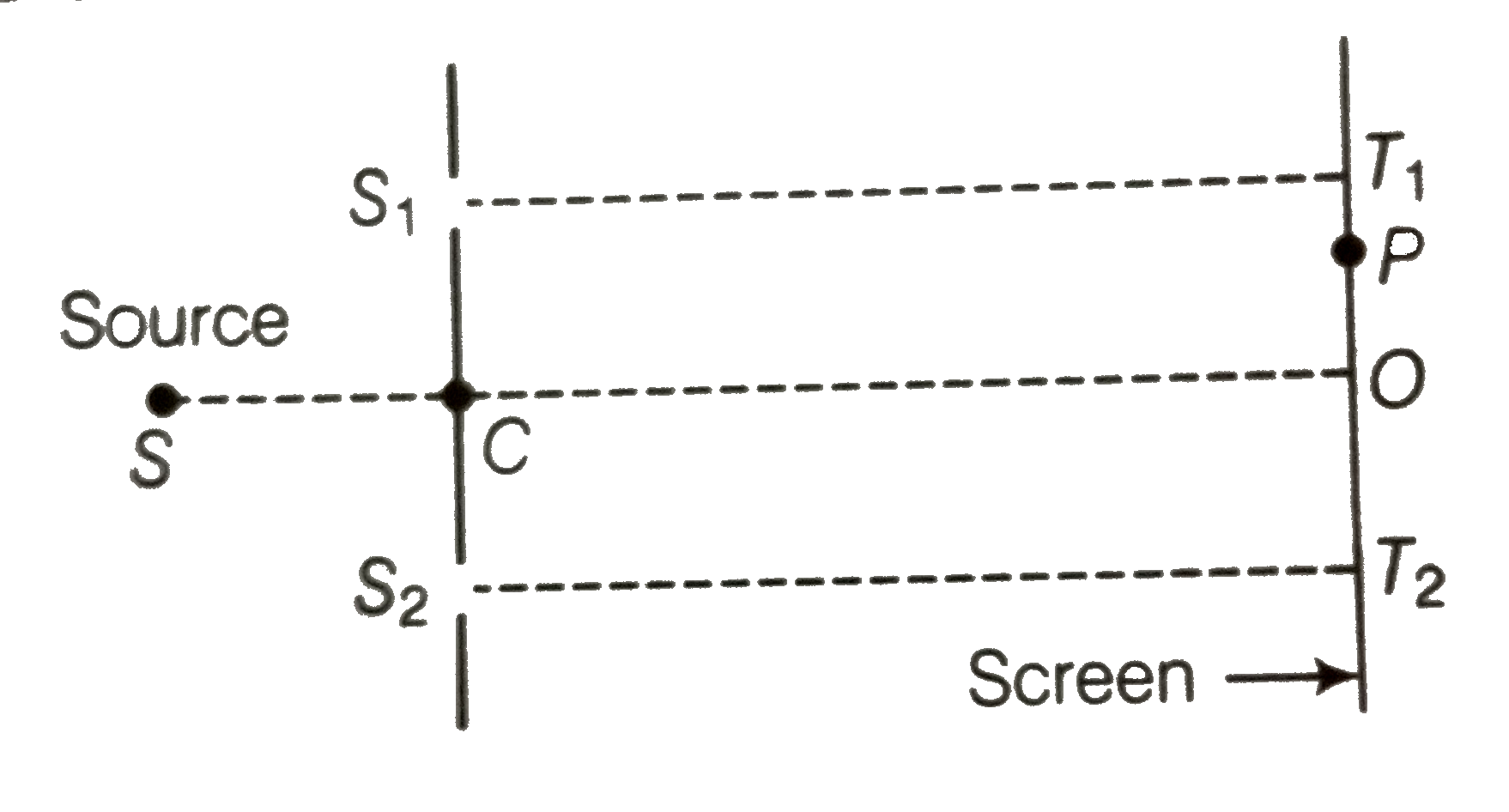
View SolutionConsider a two slit interference arrangements (figure) such that the distance of the screen from the slits is half the distance between the slits. Obtain the value of D in terms of λ such that the first minima on the screen falls at a distance D from the centre O.
View Solution
Knowledge Check
In a YDSE arrangement, the distance of screen from the slits is half the distance between the slits. If 'λ' is the wavelength of then the value of D such that first minima on the screen falls at a distance D from then centre 'O' is
 AD=λ√5BD=λ2CD=λ5√2DD=λ2√5
AD=λ√5BD=λ2CD=λ5√2DD=λ2√5In Young's experiment the distance between two slits is d3 and the distance between the screen and the slits is 3D. The number of fringes in 13 metre on the screen, formed by monochromatic light of wavelength 3λ, will be:
Ad9DλBd27DλCd81DλDdDλIn young's double slit experiment, distance between the slit S1andS2 is d and the distance between slit and screen is D . Then longest wavelength that will be missing on the screen in front of S1 is
Ad2DBd22DCDd2DNone of these
In Young's double slit experiment, how many maxima can be obtained on a screen (including central maxima). If d=5λ2 (where λ is the wavelength of light)?
View SolutionIn Young's double slit experiment, how many maxima can be obtained on a screen (including central maxima). If d=5λ2 (where λ is the wavelength of light)?
View SolutionIn Young's double-slit experiment , d is separation between the slits. Separation between plane of the slits and screen is D. Wavelength of light used is λ. Number of fringes per unit distance on the screen is
View SolutionIn a Young's double slit experiment, the separation of the two slits is doubled. To keep the samme spacing of fringes, the distance D of the screen from the slits should be made
View SolutionIn a Young’s double slit experiment, the separation of the two slits is doubled. To keep the same spacing of fringes, the distance D of the screen from the slits should be made
View Solution
