Question

The upper limit of the median class of the following frequency distribution.
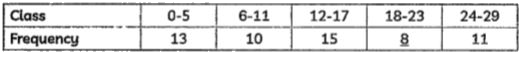
$\begin{array}{cccccc}\text{Class}& 0-5& 6-11& 12-17& 18-23& 24-29\\ \text{Frequency}& 13& 10& 15& 8& 11\end{array}$

Answer

17.5

Explanation

To find the upper limit of the median class from the given frequency distribution, we will follow these steps:

Step 1: Identify the Class Intervals and Frequencies
The given frequency distribution is:
- Class Intervals: 0-5, 6-11, 12-17, 18-23, 24-29
- Frequencies: 13, 10, 15, 8, 11

Step 2: Calculate the Cumulative Frequency
We will calculate the cumulative frequency for each class interval.

- For the first class (0-5): Cumulative Frequency = 13
- For the second class (6-11): Cumulative Frequency = 13 + 10 = 23
- For the third class (12-17): Cumulative Frequency = 23 + 15 = 38
- For the fourth class (18-23): Cumulative Frequency = 38 + 8 = 46
- For the fifth class (24-29): Cumulative Frequency = 46 + 11 = 57

So, the cumulative frequencies are:
- 0-5: 13
- 6-11: 23
- 12-17: 38
- 18-23: 46
- 24-29: 57

Step 3: Determine the Total Frequency (n)
The total frequency \( n \) is the sum of all frequencies:
\( n = 13 + 10 + 15 + 8 + 11 = 57 \)

Step 4: Calculate \( \frac{n}{2} \)
Now, we calculate \( \frac{n}{2} \):
\( \frac{n}{2} = \frac{57}{2} = 28.5 \)

Step 5: Identify the Median Class
We need to find the cumulative frequency that is just greater than \( 28.5 \). Looking at our cumulative frequencies:
- 0-5: 13
- 6-11: 23
- 12-17: 38 (this is the first cumulative frequency greater than 28.5)

Thus, the median class is 12-17.

Step 6: Determine the Upper Limit of the Median Class
The upper limit of the median class (12-17) is:
\( 17 \)

Final Answer
The upper limit of the median class is 17.

---