Expression of orbital magnetic moment is
μi=evr
μi=evr2
μi=evr2
μi=ev2r
The correct Answer is:D
μi=evr2
μi=lA
=ev2πrπr2
The ratio of e2me is known as gyromagnetic ratio which is constant. The value is 8.8×1010Ckg−1.
Topper's Solved these Questions
- BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 7EXERCISE - SECTION-C6videos
SAMPLE PAPER 7
- BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 7EXERCISE - SECTION-C6videos
SAMPLE PAPER 7
- BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 6EXERCISE - Section-C6videos
SAMPLE PAPER 6
- BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 9 EXERCISE - SECTION - C3videos
SAMPLE PAPER 9
Similar Questions
An electron in a hydrogen atom is in an excited state and corresponding principal quantum number is n. Obtain the expression for orbital magnetic moment of electron. Charge on electron is e, mass of electron is m.
View SolutionAn electron in the ground state of hydrogen atom is revolving in anti-clockwise direction in a circular orbit of radius R.

(i) The expression for the orbital magnetic moment of the electrton is ehnπm. Find n □
(ii) The atom is placed in a uniform magnetic induction B such that the normal to the plane of electron’s orbit makes an angle of 30∘ with the magnetic induction. The torque experienced by the orbiting electron is ehBnπm. Find n □View Solution
Knowledge Check
- Question 1 - Select One
Expression of orbital magnetic moment is
Aμi=evrBμi=evr2Cμi=evr2Dμi=ev2r - Question 2 - Select One
The angle made by orbital angular momentum of electron with the direction of the orbital magnetic moment is
A120∘B60∘C180∘D90∘
Show how a current loop acts as a magnetic dipole. Derive an expression for its magnetic moment.
View SolutionObtain an expression for the magnetic moment of an electron moving with a speed ‘v’ in a circular orbit of radius ‘r’. How does the magnetic moment change when :
(i) the frequency of revolution is doubled?
(ii) the orbital radius is halved?View SolutionDerive an expression for the magnetic moment (→μ) of an electron revolving around the nucleus in termsof its angular momentum (→l). What is the direction of the magnetic moment of the electron with respect to its angular momentum?
View SolutionAn electron in the ground state of hydrogen atom is revolving in anti-clockwise direction in the circular orbit of radius R as shown in figure.
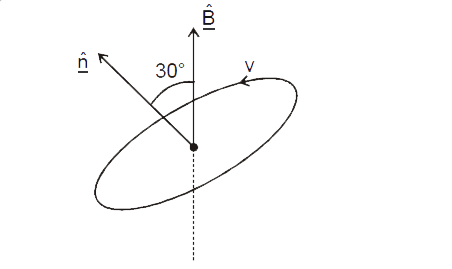
Obtain an expression for the orbital magnetic dipole moment of the electron.View SolutionDefine the term: magnetic dipole moment of a current loop. Write the expression for the magnetic moment when an electron revolves at a speed v around an orbit of radius r in hydrogen atom.
View SolutionDefine the term: magnetic dipole moment of a current loop. Write the expression for the magnetic moment when an electron revolves at a speed v around an orbit of radius r in hydrogen atom.
View Solution

