Show that a boat must move at an angle with respect to river water in order to cross the river in minimum time.
Motion of a boat in a river: Let a boat can travel with a speed of VbE in still water W.r. to earth. It is used to cross a river which flows with a speed of VWE with respect to earth. Let width of river is W.
We can cross the river in two different ways.
1) in shortest path
2) in shortest time.
To cross the river in shortest time:
Shortest time, t =width of river velocity of boat=WVbE
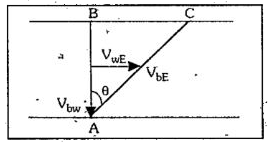
To cross the river in shortest time.boat must be rowed along the width of river i.e., boat must be rówed perpendicular to the bank or 90∘ with the flow of water. Because width of river is the shortest distance. So velocity must be taken in that direction, to obtain shortest time. In this case VBEandVWE are perpendicular. and boat will travel along AC. The distance BC is called drift: So to cross the river in shortest time angle with flow of water =90∘.
Topper's Solved these Questions
- MODEL PAPER 12BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - MODEL PAPER 12EXERCISE - SECTION - C
3 Videos
- MODEL PAPER 12BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - MODEL PAPER 12EXERCISE - SECTION - C
3 Videos
- MODEL PAPER 11BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - MODEL PAPER 11EXERCISE - Section - C
3 Videos
- MODEL PAPER 13BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - MODEL PAPER 13EXERCISE - SECTION - C
4 Videos
Similar Questions
Crossing River In Minimum Time
View SolutionA boat can be rowed at 5 m/s on still water. It is used to cross a 200m wide river from the south bank to the north bank. The river current has uniform velocity of 3 m/s due east.
(a) In which direction must it be steered to cross the river perpendicular to current ?
(b) How long will it take to cross the river in a direction perpendicular to the river flow ?
(c) In which direction must the boat be steered to cross the river in minimum time ? How far will it drift ?
View Solution
Knowledge Check
A boat crossing a river moves with a velocity v relative to still water. The river is flowing with a velocity v/2 with respect to the bank. The angle with respect to the flow direction with which the boat should move to minimize the drift is–
A30∘B60∘C150∘D120∘STATEMENT-1: A man can cross river of width d in minimum time t. On increasing river velocity, minimum time to cross the river by man will remain uncharged.
STATEMENT-2: Velocity of river is perpendicular to width of river. So time to cross the river is independent of velcoity of river.AStatement-I is true, Statement-II is true, Statement-II is correct explanation for Statement-IBStatement-I is true, Statement-II is true, Statement-II is NOT a correct explanation for Statement-ICStatement-I is true, Statement-II is falseDStatement-I is false, Statement-II is true.
A boat can be rowed at 5 m/s on still water. It is used to cross a 200m wide river from the south bank to the north bank. The river current has uniform velocity of 3 m/s due east.
(a) In which direction must it be steered to cross the river perpendicular to current ?
(b) How long will it take to cross the river in a direction perpendicular to the river flow ?
(c) In which direction must the boat be steered to cross the river in minimum time ? How far will it drift ?
View SolutionA boat is moving with a velocity (3ˆi+ˆj) with respect to ground. The water in the river is moving with a velocity −3ˆi−4ˆj with respect to ground. What is the relative velocity of boat with respect to river ?
View SolutionThe river 500 m wide is flowing with a current of 4kph. A boat starts from one bank of the river wishes to cross the river at right angle to stream direction. Boatman can row the boat at 8 kph. In which direction he should row the boat. What time he ''ll take to cross the river ?
View SolutionA rever 500m wide flows at a rate at a rate of 4kmh−1. A swinmmer who can swin at 8kmh−1. In still water, wishes to cross the river straight . (i) Along what direection must he strike? (ii) What should be his resultant velocity. (ii) What is the time of crossing the river?
View SolutionA man wishes to cross a river which is flowing at a certain speed . Man uses a motor boat whose velocity with respect to water is more than speed of flow. Minimum possible time the boat takes to cross the river is t1 , but drift along the length of river is x . When boat takes shortest route to cross the river , then it takes time t2 . Find the velocity of boat with respect to water .
View SolutionSTATEMENT-1: A man can cross river of width d in minimum time t. On increasing river velocity, minimum time to cross the river by man will remain unchanged.
STATEMENT-2: Velocity of river is perpendicular to width of river. So time to cross the river is independent of velcoity of river.
View Solution
