The magnetic field at the point of intersection of diagonals of a square loop of side L carrying a current I is measured, Let the measured value be Nμ0IπL. What will be the value of N ?
Text Solution
The correct Answer is:02.82
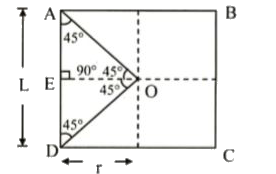
Here r=OE=L2.
Magnetic field at the centre O due to the current element AD is given as
BAD=μ0I4πr[sin45∘+sin45∘]=μ0I4πr.2√2
Similarly
BDC=BBC=BAB=BAD
Bnet=4BAD=4.μ0I4πr2√2=μ0Iπ(L2).2√2
=2√2μ0IπL
∴Bnet=2.82μ0IπL
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
The magnetic field at the point of intersection of diagonals of a square wire loop of side L, carrying a current I is
Aμ0IπLB2μ0IπLC√2μ0IπLD2√2μ0IπLThe magnetic field at the centre of an equilateral triangular loop of side 2L and carrying a current i is
A9μ0i4πLB3√3μ0i4πLC2√3μ0iπLD3μ0i4πLMagnetic field at the centre of coil of n turns, bent in the form of a square of side 2l, carrying current i, is
A0.5×10−5TB1.25×10−4TC3.0×10−5TD4×10−5T
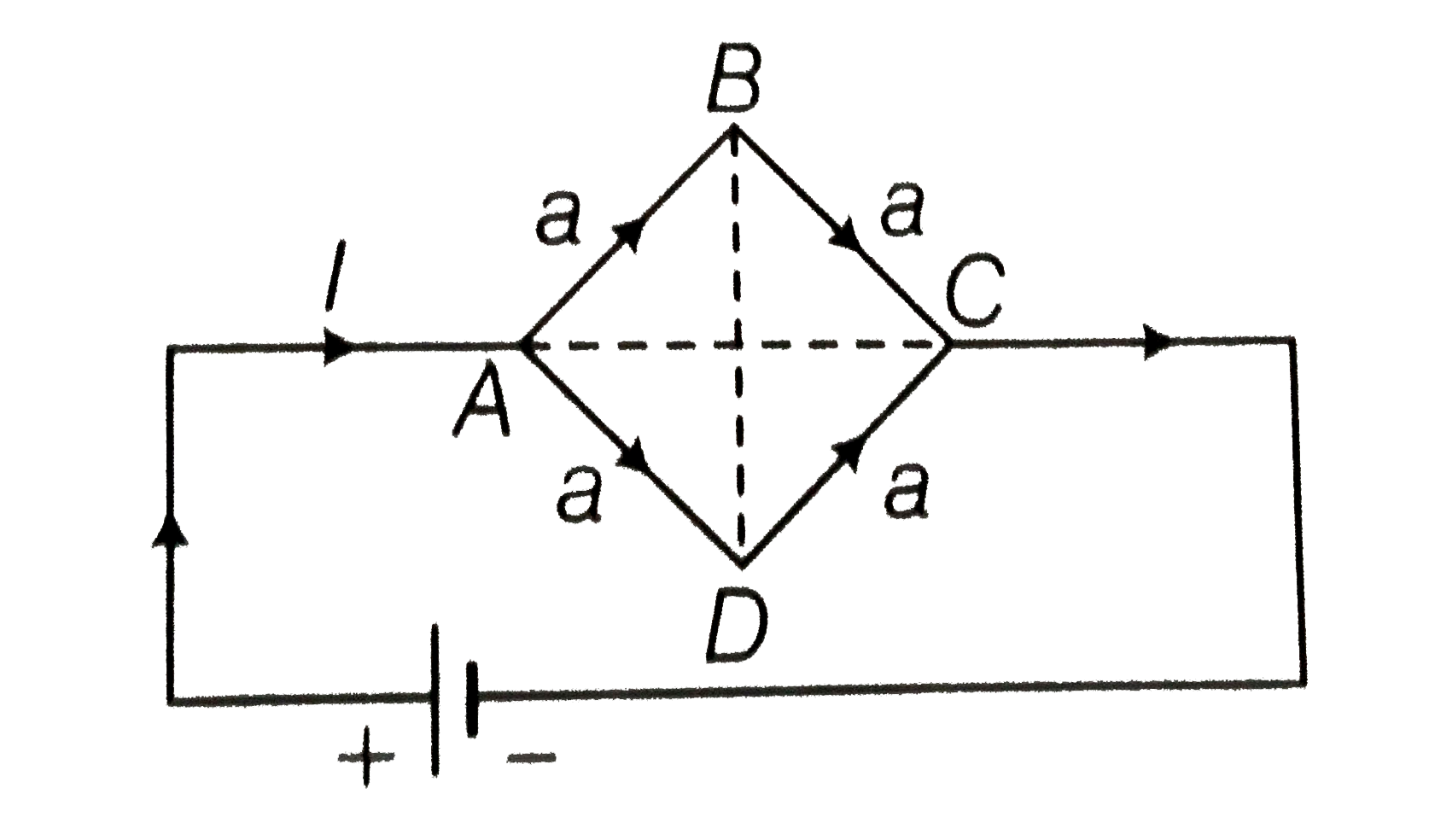
Magnetic field induction at the centre O of a square loop of side a carrying current I as shown in figure.
View SolutionThe magnetic field at centre of a hexagonal coil of side l carrying a current I is

View SolutionFind the magnetic field B at the centre of a rectangular loop of length l and width b, carrying a current i.
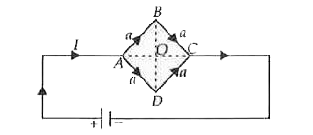
View SolutionMagnetic field induction at the centre of a square loop of side 'a' carrying current I as shown in figure is
View SolutionThe magnetic field at centre of a hexagonal coil of side l carrying a current I is

View Solution
