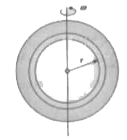
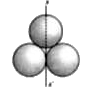
Find the moment of inertia of the system of three solid spheres of mass M and radius R are shown in the figure about the $\times .$

A

$\frac{7}{2} M R^{2}$

B

$\frac{14}{5} M R^{2}$

C

$\frac{16}{5} M R^{2}$

D

$\frac{21}{5} M R^{2}$

Text Solution

Verified by Experts

The correct Answer is:C

Moment of inertia of a sphere about its
axis, $I_{1} = \frac{2}{5} M R^{2}$
For sphere 2,

Using parallel ais theorem,
$I = I_{C M} + M d^{2}$
$\Rightarrow I_{2} = \frac{2}{5} M R^{2} + M R^{2}$
$\Rightarrow I_{2} = \frac{7}{5} M R^{2}$
Moment of inertial of second and third sphere is same.
$I_{3} = I_{2} = \frac{7}{5} M R^{2}$
Total moment of inertia of the system,
$I = \frac{2}{5} M R^{2} + \frac{7}{5} M R^{2} + \frac{7}{5} M R^{2}$
$I = \frac{16}{5} M R^{2}$

|

Updated on:21/07/2023

Class 12 PHYSICS QUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Find the moment of inertia of a hemisphere of mass $M$ and radius $R$ shown in the figure, about an axis $A A'$ tangential to the hemisphere.

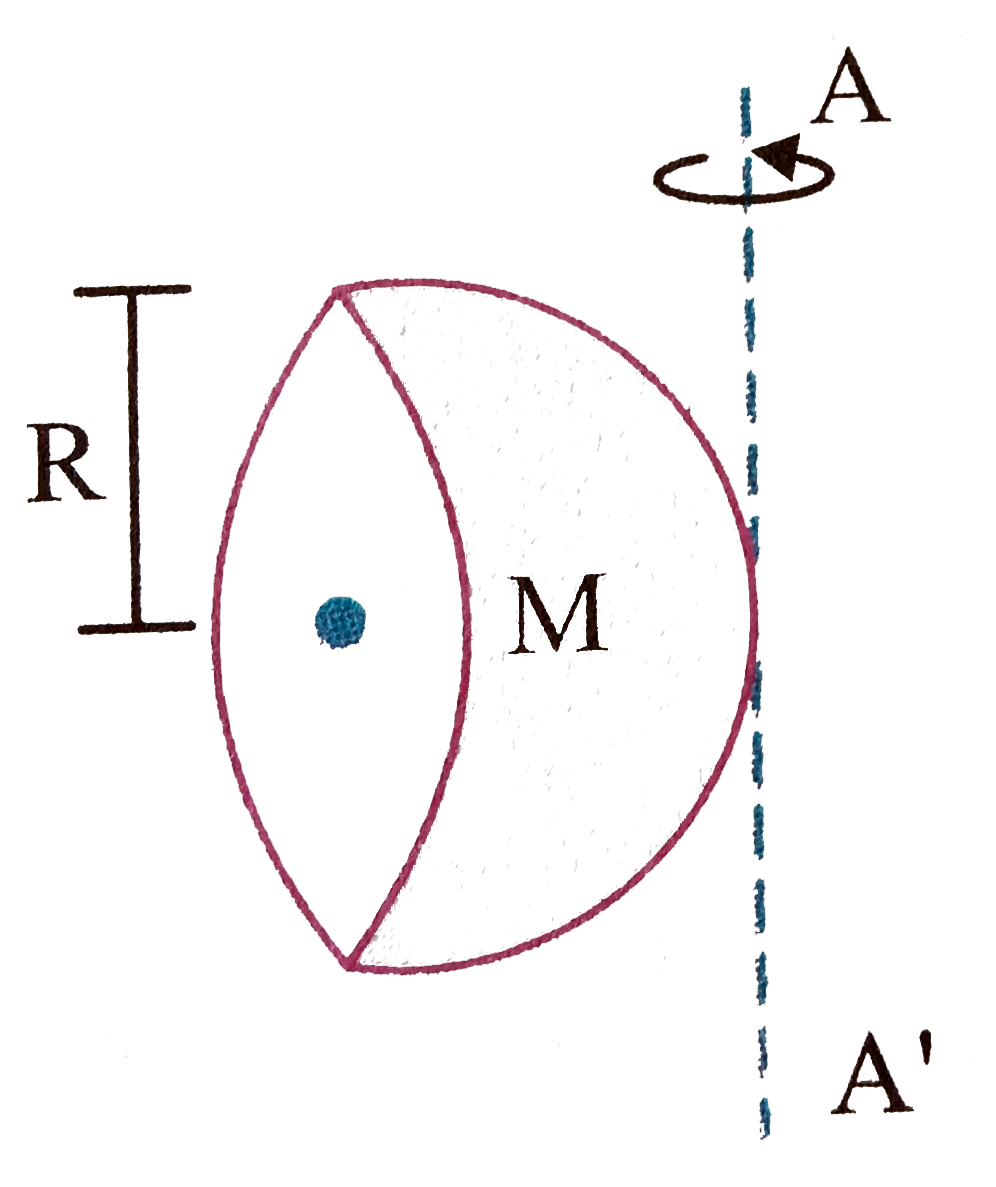A $I = (\frac{9}{20}) m R^{2}$
B $I = (\frac{13}{20}) m R^{2}$
C $I = (\frac{7}{20}) m R^{2}$
D $I = (\frac{3}{20}) m R^{2}$
Question 2 - Select One
The moment of inertia of a solid sphere of mass ‘M' and radius 'R' about a tangent to the sphere is
A $\frac{2}{5} M R^{2}$
B $\frac{6}{3} M R^{2}$
C $\frac{4}{5} M R^{2}$
D $\frac{7}{5} M R^{2}$
Question 3 - Select One

Find the moment of inertia of a solid sphere of mass $M$ and radias $R$ about an axis XX shown in figure.
A $\frac{7}{5} M R^{2}$
B $\frac{4}{5} M R^{2}$
C $\frac{3}{5} M R^{2}$
D $\frac{2}{5} M R^{2}$

NTA MOCK TESTS-NTA TPC JEE MAIN TEST 52-PHYSICS

Home
Ask Doubt
Profile