Two large non conducting plates having surface charge densities +σ and −σ respectively, are fixed d distnce apart. A small test charge q of mass m is attached to two non conducting springs each of spring constant k as shown in the figure. The sum of lengths of both springs in underformed state is d. The charge q is released from rest with both the springs nondeformed. then charge q will (neglect gravity)
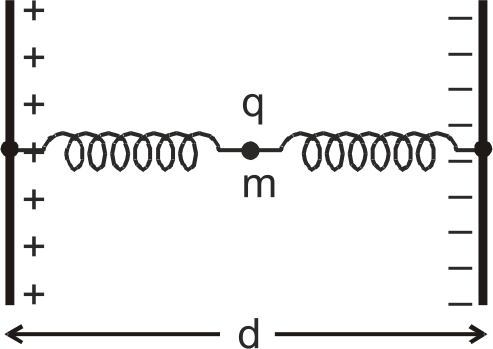
perform SHM with angular frequence √km
not perform SHM with amplitude σq2kε0
not perform SHM but will have a periodic moton
remain stationary
The correct Answer is:B
Eelctric fiedl between plastes is uniform hence electrostatic force due to this field is constant. Angular frequency does not change by constant force equilibrium position will be at the point where 2kx0=q×σ∈0,x0=σq2k∈0
x0 is also the amplitude of oscillation.
Topper's Solved these Questions
Similar Questions
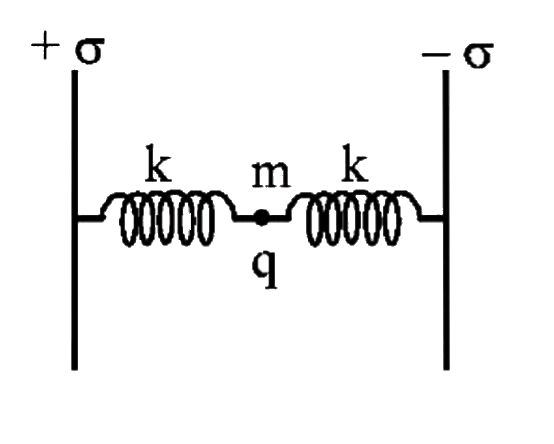
Two large insulating plates having surface charge densities +σ and −σ are fixed some distance apart in a gravity -free region and two ideal insulating springs of force constant k are connected to the plates as shown in the figure. A particle of charge q and mass m which is attached to the junction of the spring is released from rest, then the partice will cross its equilibrium position with a speed
View SolutionElectric field due to an infinite non-conducting sheet of surface charge density σ, at a distance r from it is
View Solution
Knowledge Check
- Question 1 - Select One
Two infinitely long parallel sheets having surface charge densities +σand−σ respectively are separated by a small distance. The electric field in the region between the plates is
A0Bσ2∈0Cσ∈0D2σ∈0 - Question 2 - Select One
Three non-conducting large parallel plates have surface charge densities σ,−2σand4σ respectively as shown in the figure. The electric field at the point P is
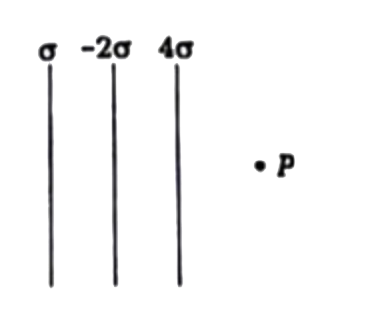Aσε0Bσ2ε0C3σ2ε0D3σε0 - Question 3 - Select One
Two equal negative charges are fixed at points and on y-axis. A positive charge Q is released from rest at the point on the x-axis. The charge Q will:
Aexecute simple harmonic motion about the originBmove to the origin and remain at restCmove to infinityDsame as at point 25 cm aways form the surface
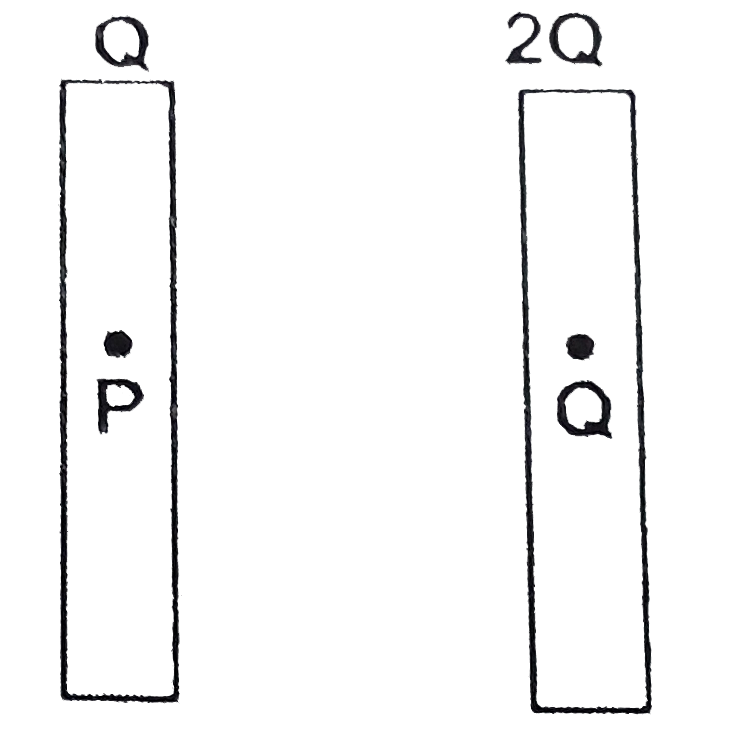
Force between two conducting large plates , with charges Q and Q2 as shown in the figure, is (Area of each face of plate =A)
_PHY_E01_022_Q01.png)
View SolutionTwo large parallel conducting sheets (placed at finite distance) are given charges Q and 2Q respectively. Find out charges appearing on all the surfaces.
View SolutionTwo blocks, of masses M and 2M, are connected to a light spring of spring constant K that has one end fixed, as shown in figure. The horizontal surface and the pulley are frictionless. The blocks are released from when the spring is non deformed. The string is light.
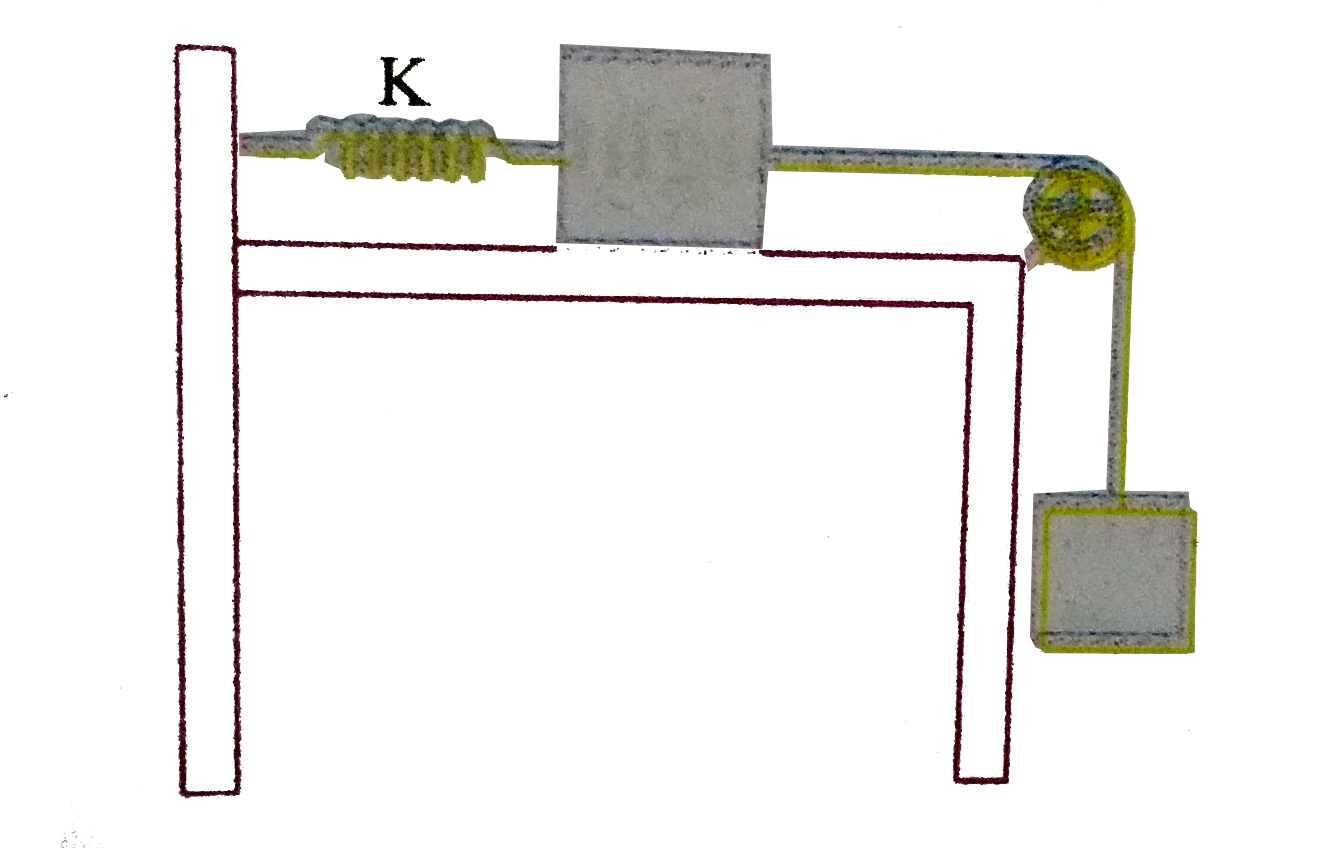.View SolutionTwo masses m and 2m are attached to two ends of an ideal spring as shown in figure. When thye spring is in the compressed state, the energy of the spring is 60 J, if the spring is released, then at its natural length
View Solution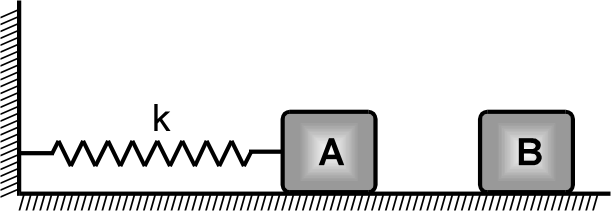
In the figure shown A & B are two charged particles having charges q and – q respectively are placed on a nonconducting fixed horizontal smooth plane. B is fixed and A is attached to a non conducting massless spring of spring constant k. The other end of the spring is fixed. Mass of A is m, A and B are in equilibrium when the distance between them is r. Choose the correct optionsView Solution