Read the following statements and choose the correct answer.
(a) For a freely falling body the average velocity is proportional to square root of height of fall.
(b) For a freely falling body the displacements in successive equal time intervals are in the ratio 1:4:9:....
(c) For a vertically projected body the displacement during last second of time of flight changes with velocity of projection.
(d) For a body projected from the top of the tower the displacement of the body is negative when the body crosses the point of projection
a,c,d are true
Both a,b are true
b and c are true
c and d are true
The correct Answer is:A
To solve the question, we need to analyze each of the statements provided regarding the motion of freely falling bodies and vertically projected bodies. Let's evaluate each statement one by one.
Step 1: Analyze Statement (a)
Statement (a): For a freely falling body, the average velocity is proportional to the square root of the height of fall.
- When a body is dropped from a height h, the average velocity vavg can be calculated using the formula:
vavg=ht
where t is the time taken to fall. Using the second equation of motion,
h=ut+12gt2
where u=0 (initial velocity), we can find t:
h=12gt2⟹t=√2hg
Substituting this back into the average velocity equation gives:
vavg=h√2hg=√gh2
Thus, the average velocity is indeed proportional to the square root of the height h. This statement is true.
Step 2: Analyze Statement (b)
Statement (b): For a freely falling body, the displacements in successive equal time intervals are in the ratio 1:4:9:...
- The displacement in successive time intervals can be calculated using:
sn=ut+12gt2
For t=1,2,3:
- s1=12g(12)=g2
- s2=12g(22)=2g
- s3=12g(32)=9g2
The displacements in successive intervals are:
- First interval: s1=g2
- Second interval: s2−s1=2g−g2=3g2
- Third interval: s3−s2=9g2−2g=5g2
The ratios of displacements are not 1:4:9. This statement is false.
Step 3: Analyze Statement (c)
Statement (c): For a vertically projected body, the displacement during the last second of time of flight changes with the velocity of projection.
- The displacement during the last second can be calculated using the formula:
s=u−12g
This clearly shows that the displacement depends on the initial velocity u. This statement is true.
Step 4: Analyze Statement (d)
Statement (d): For a body projected from the top of the tower, the displacement of the body is negative when the body crosses the point of projection.
- If we take upward as positive, then when the body falls below the point of projection (the height from which it was thrown), the displacement will be negative. This statement is true.
Conclusion
The correct statements are (a), (c), and (d). The only incorrect statement is (b).
Final Answer
The correct answer is: (a), (c), and (d) are true; (b) is false.
---
Statement (a): For a freely falling body, the average velocity is proportional to the square root of the height of fall.
vavg=ht
where t is the time taken to fall. Using the second equation of motion,
h=ut+12gt2
where u=0 (initial velocity), we can find t:
h=12gt2⟹t=√2hg
Substituting this back into the average velocity equation gives:
vavg=h√2hg=√gh2
Thus, the average velocity is indeed proportional to the square root of the height h. This statement is true.
Statement (b): For a freely falling body, the displacements in successive equal time intervals are in the ratio 1:4:9:...
sn=ut+12gt2
For t=1,2,3:
- s1=12g(12)=g2
- s2=12g(22)=2g
- s3=12g(32)=9g2
- First interval: s1=g2
- Second interval: s2−s1=2g−g2=3g2
- Third interval: s3−s2=9g2−2g=5g2
Statement (c): For a vertically projected body, the displacement during the last second of time of flight changes with the velocity of projection.
s=u−12g
This clearly shows that the displacement depends on the initial velocity u. This statement is true.
Statement (d): For a body projected from the top of the tower, the displacement of the body is negative when the body crosses the point of projection.
The correct statements are (a), (c), and (d). The only incorrect statement is (b).
The correct answer is: (a), (c), and (d) are true; (b) is false.
Topper's Solved these Questions
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - EXERCISE -II
80 Videos
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - PRACTICE EXERCISE
58 Videos
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - very Short answer type question
15 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - QUESTION FOR DESCRIPTIVE ANSWER
7 Videos
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH SERIESCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - EXERCISE-III
49 Videos
Similar Questions
Knowledge Check
For a freely falling body the potential energy at the top ..........
Ais zeroBis completely converted to KECis equal to gravitational potential energyDis minimum
For a freely falling body, Find the ratio of the times taken to fall successive equal distances.
View SolutionIn the case of a body freely falling from small height
View SolutionA body is dropped from the top of the tower and falls freely.
The distance coverd by it after n seconds is directly proportional to .
View SolutionA body is dropped from the top of the tower and falls freely.
The velocity of the body after n seconds is proportional to .
View SolutionThe distance travelled by a freely falling body is proportional to
View SolutionA freely falling body travels _____ of total distance in 5th second
View SolutionThe velocity versus time graph of a body in a straight line is as shown in figure. The displacment of the body in five seconds is
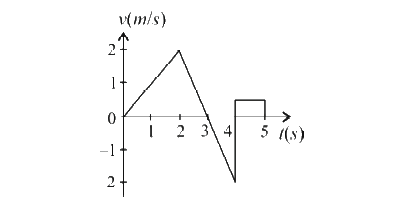
View Solution
