Show that the trajectory of an object thrown at certain angle with the horizontal is a parabola .
To show that the trajectory of an object thrown at a certain angle with the horizontal is a parabola, we can derive the equations of motion for the projectile and manipulate them to arrive at the standard form of a parabolic equation. Here’s a step-by-step solution:
Step 1: Define the Initial Conditions
Let the object be thrown with an initial velocity u at an angle θ with the horizontal. We can resolve the initial velocity into horizontal and vertical components:
- Horizontal component:
ux=ucosθ
- Vertical component:
uy=usinθ
Step 2: Analyze Horizontal Motion
In horizontal motion, there is no acceleration (assuming air resistance is negligible), so the horizontal velocity remains constant:
vx=ux=ucosθ
The horizontal displacement x after time t is given by:
x=ux⋅t=(ucosθ)t
From this, we can express time t in terms of x:
t=xucosθ
Step 3: Analyze Vertical Motion
In vertical motion, the only acceleration acting on the object is due to gravity g (acting downwards). The vertical displacement y after time t can be described by the second equation of motion:
y=uyt−12gt2
Substituting uy and t:
y=(usinθ)t−12gt2
Now, substituting t=xucosθ into the equation for y:
y=(usinθ)(xucosθ)−12g(xucosθ)2
Step 4: Simplify the Equation
Now simplify the equation:
y=sinθcosθx−12gx2(ucosθ)2
y=xtanθ−g2u2cos2θx2
Step 5: Identify the Parabolic Form
The equation we derived is:
y=mx−kx2
where m=tanθ and k=g2u2cos2θ. This is a quadratic equation in x, which is the standard form of a parabola.
Conclusion
Thus, we have shown that the trajectory of an object thrown at an angle θ with the horizontal is a parabola.
---
Let the object be thrown with an initial velocity u at an angle θ with the horizontal. We can resolve the initial velocity into horizontal and vertical components:
ux=ucosθ
- Vertical component:
uy=usinθ
In horizontal motion, there is no acceleration (assuming air resistance is negligible), so the horizontal velocity remains constant:
x=ux⋅t=(ucosθ)t
t=xucosθ
In vertical motion, the only acceleration acting on the object is due to gravity g (acting downwards). The vertical displacement y after time t can be described by the second equation of motion:
y=(usinθ)t−12gt2
y=(usinθ)(xucosθ)−12g(xucosθ)2
Now simplify the equation:
y=sinθcosθx−12gx2(ucosθ)2
y=xtanθ−g2u2cos2θx2
The equation we derived is:
y=mx−kx2
where m=tanθ and k=g2u2cos2θ. This is a quadratic equation in x, which is the standard form of a parabola.
Thus, we have shown that the trajectory of an object thrown at an angle θ with the horizontal is a parabola.
Topper's Solved these Questions
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - VERY SHORT ANSWER TYPE QUESTIONS
10 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - NUMERICAL EXERCISE (LEVEL -1)
33 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - QUESTION FOR DESCRIPTIVE ANSWER
7 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - EXERCISE-3 (Circular Motion)
7 Videos
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - very Short answer type question
15 Videos
Similar Questions
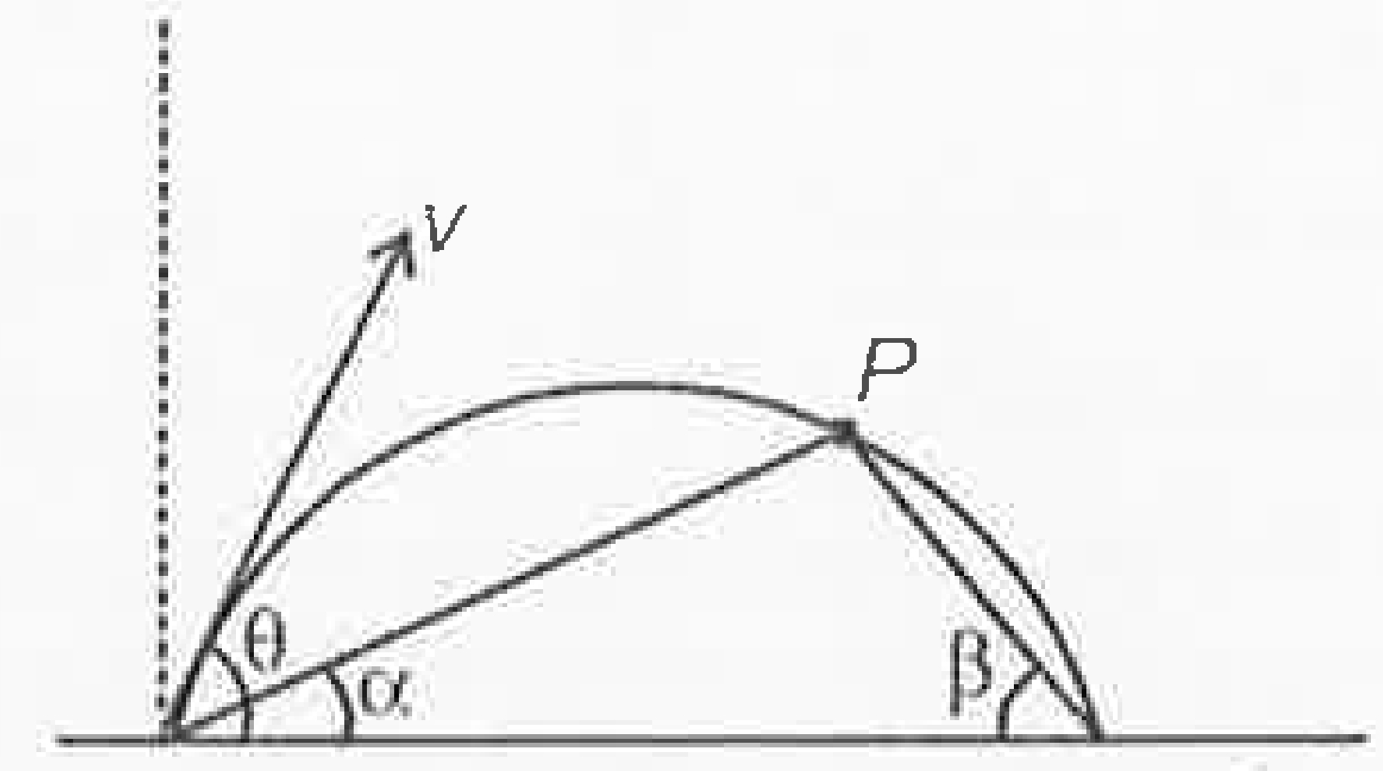
A point P on the trajectory of a projectile projected at angle with θ with horizontal subtrends angles α and β at the point of landing them
View SolutionThe maximum height attained by a projectile when thrown at an angle θ with the horizontal is found to be half the horizontal range. Then θ is equal to
View Solution
Knowledge Check
The angle which the velocity vector of a projectile thrown with a velocity v at an. angle θ to the horizontal Will make with the horizontal after time t of its being thrown up is
AθBtan−1(θ/t)Ctan−1(vcosθvsinθ−gt)Dtan−1(vsinθ−gtvcosθ)
A force acts on a body at a certain angle with the horizontal such that the vertical component is twice the horizontal component . What is the inclination of the force ?
View SolutionA projectile thrown with an initial speed u and the angle of projection 15∘ to the horizontal has a range R. If the same projectile is thrown at an angle of 45∘ to the horizontal with speed 2u, what will be its range ?
View SolutionIf two projectile of same mass are thrown at an angle of 50° and 40° with the horizontal with the same speed then, which of the following statement is true?
View SolutionA particle is thrown at time t = 0 with a velocity of 10ms−1 at an angle 60∘ with the horizontal from a point on an inclined plane, making an angle of 30∘ with the horizontal. The time when the velocity of the projectile becomes parallel to the incline is

View SolutionA particle is thrown at time t = 0 with a velocity of 10ms−1 at an angle 60∘ with the horizontal from a point on an inclined plane, making an angle of 30∘ with the horizontal. The time when the velocity of the projectile becomes parallel to the incline is

View SolutionA particle is thrown at time t = 0 with a velocity of 10ms−1 at an angle 60∘ with the horizontal from a point on an inclined plane, making an angle of 30∘ with the horizontal. The time when the velocity of the projectile becomes parallel to the incline is

View SolutionIf the escape velocity of the body which is thrown from earth surface is Ve, then the escape velocity of the body if it is thrown at an angle θ from horizontal earths surface is
View Solution
