A river is of width 120m which flows at a speed pf 8ms−1. If a man swims with a speed of 5ms−1 at an angle of 127∘ with the stream, his drift on reaching other bank is
50 m
150 m
200 m
300 m
The correct Answer is:B
To solve the problem, we need to determine the drift of the man as he swims across the river. Here’s a step-by-step breakdown of the solution:
Step 1: Understand the Problem
We have a river that is 120 meters wide and flows at a speed of 8 m/s. A man swims at a speed of 5 m/s at an angle of 127° with respect to the direction of the river flow. We need to find out how far downstream (drift) he will end up when he reaches the opposite bank.
Step 2: Resolve the Swimming Velocity
The swimming velocity of the man can be resolved into two components:
- Vertical Component (across the river): This is the component of his swimming speed that helps him cross the river.
- Horizontal Component (along the river): This is the component of his swimming speed that contributes to the drift downstream.
The angle with respect to the river flow is given as 127°. To find the angle with respect to the perpendicular direction (which is 90°), we calculate:
- Angle with respect to vertical = 127° - 90° = 37°.
Now, we can resolve the velocity:
- Vertical component Vy=Vman⋅cos(37°)
- Horizontal component Vx=Vman⋅sin(37°)
Where Vman=5m/s.
Using trigonometric values:
- cos(37°)≈45
- sin(37°)≈35
Calculating the components:
- Vy=5⋅cos(37°)=5⋅45=4m/s
- Vx=5⋅sin(37°)=5⋅35=3m/s
Step 3: Calculate the Time to Cross the River
The time taken to cross the river can be calculated using the width of the river and the vertical component of the man's swimming speed:
Time=Width of the riverVy=120m4m/s=30s
Step 4: Calculate the Drift
During the time the man is swimming across the river, he will also be carried downstream by the river's current. The effective horizontal speed (downstream) is the sum of the river's speed and the man's horizontal component:
Veffective=Vriver−Vx=8m/s−3m/s=5m/s
Now, we can calculate the drift:
Drift=Veffective⋅Time=5m/s⋅30s=150m
Final Answer
The drift on reaching the other bank is 150 meters.
---
We have a river that is 120 meters wide and flows at a speed of 8 m/s. A man swims at a speed of 5 m/s at an angle of 127° with respect to the direction of the river flow. We need to find out how far downstream (drift) he will end up when he reaches the opposite bank.
The swimming velocity of the man can be resolved into two components:
- Vertical Component (across the river): This is the component of his swimming speed that helps him cross the river.
- Horizontal Component (along the river): This is the component of his swimming speed that contributes to the drift downstream.
- Angle with respect to vertical = 127° - 90° = 37°.
- Vertical component Vy=Vman⋅cos(37°)
- Horizontal component Vx=Vman⋅sin(37°)
- cos(37°)≈45
- sin(37°)≈35
- Vy=5⋅cos(37°)=5⋅45=4m/s
- Vx=5⋅sin(37°)=5⋅35=3m/s
The time taken to cross the river can be calculated using the width of the river and the vertical component of the man's swimming speed:
Time=Width of the riverVy=120m4m/s=30s
During the time the man is swimming across the river, he will also be carried downstream by the river's current. The effective horizontal speed (downstream) is the sum of the river's speed and the man's horizontal component:
Veffective=Vriver−Vx=8m/s−3m/s=5m/s
Drift=Veffective⋅Time=5m/s⋅30s=150m
The drift on reaching the other bank is 150 meters.
Topper's Solved these Questions
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - EXERCISE-3 (Oblique projection )
25 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - EXERCISE-3 (Circular Motion)
7 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - EXERCISE-3 (Relative velocity)
5 Videos
- MAGNETISMBOOK - AAKASH SERIESCHAPTER - MAGNETISMEXERCISE - ADDITIONAL EXERCISE
22 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - QUESTION FOR DESCRIPTIVE ANSWER
7 Videos
Similar Questions
Person aiming to reach the exactly opposite point on the bank of a stream is swimming with a speed of 0.5ms−1 at an angle of 120∘ with the direction of flow of water.The speed of water in the stream is
View SolutionA swimmer crosses a river of width d flowing at velocity v. While swimming, he keeps himself always at an angle of 120∘ with the river flow and on reaching the other end. He finds a drift of d/2 in the direction of flow of river. The speed of the swimmer with respect to the river is :
View Solution
Knowledge Check
A river is flowing due east with a speed 3ms−1. A swimmer can swim in still water at a speed of 4ms−1. If swimmer starts swimming due north, then the resultant velocity of the swimmer is a) 3 m/s b) 5 m/s c) 7 m/s d) 2 m/s
A3 m s−1B5 m s−1C7 m s−1D2 m s−1
A person aiming to reach exactly opposite point on the bank of a stream is swimming with a speed of 0.6 m/s at an angle of 120∘ with the direction of flow of water . The speed of water in the stream is
View SolutionRain, pouring down at an angle α with the vertical has a speed of 10ms−1.A girl runs against the rain with a speed of 8ms/−1 and sees that the rain makes an angle β with the vertical, then relation between α and β is
View SolutionA river is flowing due east with a speed 3ms−1 A swimmer can swim in still water at a speed of 4ms−1 If swimmer starts swimming due north what will be his resultant velocity (magnitude and direction )?
View SolutionA river is flowing with a speed of 1kmh−1 A swimmer wants to go point C starting from A. He swims with a speed of 5kmh−1 at an angle θ w.r.t. the river flow. If AB=BC=400m, at what angle with the river bank should the swimmer swim ?
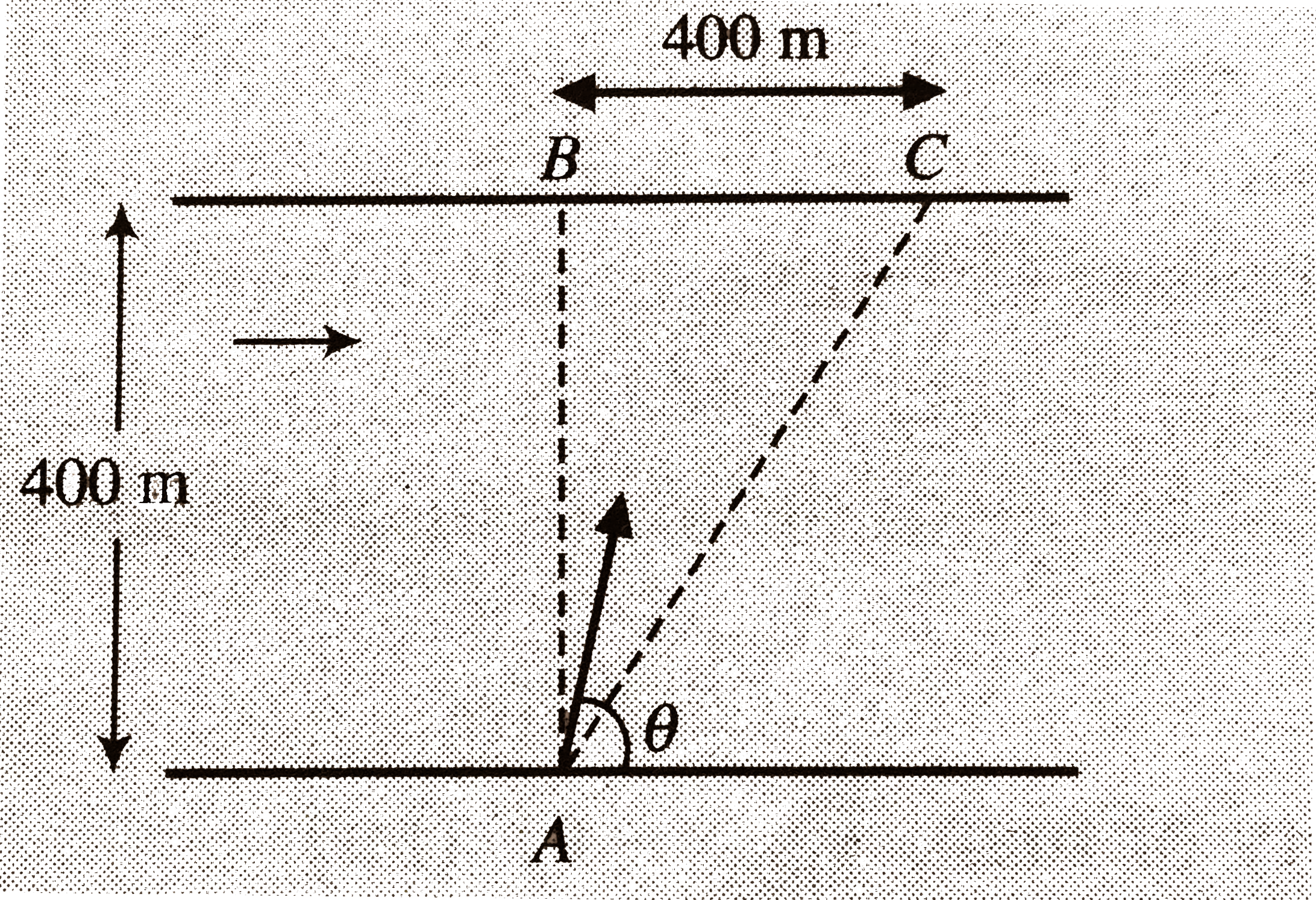 .
.
View SolutionA man walks for 1 minute at a speed of 1ms−1 and then runs for 1min at a speed of 3ms−1 along straight track, What is the average speed of the man?
View SolutionA swimmer swins in still water at a speed =5km/hr. He enters a 200m wide river, having river flow speed =4km/hr at point A and proceeds to swim at an angle of 127∘(sin37∘=0.6) with the river flow direction. Another point B is located directly across A on the other side. the swimmer lands on the other bank at a point C, form which he walks the distance CB with a speed =3km/hr. The total time in which he reaces from A to B is
View SolutionRain is falling vertically with a speed of 20ms−1., A person is running in the rain with a velocity of 5ms−1 and a wind is also blowing with a speed of 15ms−1 (both from the west) The angle with the vertical at which the person should hold his umbrella so that he may not get drenched is:
View Solution
