The time lag between two particles vibrating in a progressive wave seperated by a distance 20m is 0.02s. The wave velocity if the frequency of the wave is 500Hz, is
1000ms−1
500ms−1
2000ms−1
250ms−1
The correct Answer is:A
To solve the problem, we need to find the wave velocity given the distance between two particles and the time lag between their vibrations. Here’s the step-by-step solution:
Step 1: Identify the given values
- Distance (s) = 20 meters
- Time lag (t) = 0.02 seconds
- Frequency (f) = 500 Hz (though we won't need this value for calculating wave velocity directly)
Step 2: Use the formula for wave velocity
The wave velocity (v) can be calculated using the formula:
v=st
where:
- v is the wave velocity,
- s is the distance between the two particles,
- t is the time lag.
Step 3: Substitute the values into the formula
Now, we substitute the given values into the formula:
v=20m0.02s
Step 4: Perform the calculation
Calculating the above expression:
v=200.02=1000m/s
Step 5: State the final answer
Thus, the wave velocity is:
v=1000m/s
- Distance (s) = 20 meters
- Time lag (t) = 0.02 seconds
- Frequency (f) = 500 Hz (though we won't need this value for calculating wave velocity directly)
The wave velocity (v) can be calculated using the formula:
v=st
where:
- v is the wave velocity,
- s is the distance between the two particles,
- t is the time lag.
Now, we substitute the given values into the formula:
v=20m0.02s
Calculating the above expression:
v=200.02=1000m/s
Thus, the wave velocity is:
v=1000m/s
Topper's Solved these Questions
- WAVE MOTIONBOOK - AAKASH SERIESCHAPTER - WAVE MOTIONEXERCISE - PRACTICE SET (EXERCISE-I (LEVEL-II(ADVANCED)STRAIGHT OBJECTIVE TYPE QUESTIONS))
8 Videos
- WAVE MOTIONBOOK - AAKASH SERIESCHAPTER - WAVE MOTIONEXERCISE - PRACTICE SET (EXERCISE-I (LEVEL-II(ADVANCED) MORE THAN ONE CORRECT ANSWER TYPE QUESTIONS))
7 Videos
- WAVE MOTIONBOOK - AAKASH SERIESCHAPTER - WAVE MOTIONEXERCISE - LECTURE SHEET (EXERCISE-VI (LEVEL-II(ADVANCED) INTEGER TYPE QUESTIONS))
1 Videos
- UNITS AND MEASUREMENTSBOOK - AAKASH SERIESCHAPTER - UNITS AND MEASUREMENTSEXERCISE - EXERCISE -3
66 Videos
- WAVE MOTION AND SOUNDBOOK - AAKASH SERIESCHAPTER - WAVE MOTION AND SOUNDEXERCISE - PROBLEMS (LEVEL - II)
97 Videos
Similar Questions
Knowledge Check
The equation of a progressive wave can be given by Y = 15 sin (660πt−0.02πx) cm. The frequency of the wave is
A330 HzB342 HzC365 HzD660 Hz
The separation between two consecutive crests in a transverse wave is 100 m. If wave velocity is 20 m s−1 , find the frequency of wave.
View SolutionThe separation between two consecutive crests in a transverse wave is 100 m. If wave velocity is 20 m s−1, find the frequency of wave.
View SolutionThe phase difference between two points separated by 0.8 m in a wave of frequency 120 Hz is 0.5π . The wave velocity is
View SolutionGive two difference between progressive wave and stationary wave.
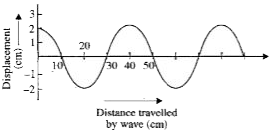
View SolutionA wave travelling along the length of a string is shown in Fig. 14.4.12. Using the scale on the given axis, find
(i) The amplitude of the wave
(ii) The wavelength of the wave
(iii) If the frequency of the wave is 10 Hz, What is the speed of the wave?
(iv) What is the initial phase of the wave ?
(v) What is the phase difference between two points separated by a distance 10 cm ?
(vi) If this wave is made to travel through water what is the wavelength of the wave, if the speed of, the waves in water is 10 m/s.
View SolutionThe angular frequency of a particle in a progressive wave in an elastic medium is 50π rad/s and it is moving with velocity of 150 m/s. The phase difference between two particles separated by a distance 30m at the same instant will be
View SolutionFrequency of a wave is 6×1015 Hz. The wave is
View Solution
