The velocities of sound in an ideal gas at temperature T1andT2 K are found to be V1andV2 respectively. If ther.m.s velocities of the molecules of the same gas at the same temperatures T1andT2 are v1andv2 respectively then
v2=v1(V1V2)
v2=v1(V2V1)
v2=v1√V2V1
v2=v1√V1V2
The correct Answer is:B
To solve the problem, we need to establish the relationship between the velocities of sound in an ideal gas and the root mean square (rms) velocities of the gas molecules at different temperatures.
1. Understanding the Velocities:
- The velocity of sound in an ideal gas is given by the formula:
V=√γRTM
where γ is the adiabatic index (ratio of specific heats), R is the universal gas constant, T is the absolute temperature, and M is the molar mass of the gas.
2. Applying the Formula for Two Temperatures:
- For temperature T1:
V1=√γRT1M
- For temperature T2:
V2=√γRT2M
3. Root Mean Square (rms) Velocity:
- The rms velocity of gas molecules is given by:
v=√3RTM
- For temperature T1:
v1=√3RT1M
- For temperature T2:
v2=√3RT2M
4. Establishing Proportional Relationships:
- From the equations for V1 and V2, we can see that:
V1∝√T1andV2∝√T2
- Similarly, for the rms velocities:
v1∝√T1andv2∝√T2
5. Setting Up the Ratio:
- We can set up the following ratios based on the proportional relationships:
V1v1=V2v2
6. Rearranging the Equation:
- From the ratio, we can express v2 in terms of V2 and V1:
v2=V2V1v1
Final Result:
Thus, the correct relationship between the velocities is:
v2=V2V1v1
- The velocity of sound in an ideal gas is given by the formula:
V=√γRTM
where γ is the adiabatic index (ratio of specific heats), R is the universal gas constant, T is the absolute temperature, and M is the molar mass of the gas.
- For temperature T1:
V1=√γRT1M
- For temperature T2:
V2=√γRT2M
- The rms velocity of gas molecules is given by:
v=√3RTM
- For temperature T1:
v1=√3RT1M
- For temperature T2:
v2=√3RT2M
- From the equations for V1 and V2, we can see that:
V1∝√T1andV2∝√T2
- Similarly, for the rms velocities:
v1∝√T1andv2∝√T2
- We can set up the following ratios based on the proportional relationships:
V1v1=V2v2
- From the ratio, we can express v2 in terms of V2 and V1:
v2=V2V1v1
Thus, the correct relationship between the velocities is:
v2=V2V1v1
Topper's Solved these Questions
- WAVE MOTIONBOOK - AAKASH SERIESCHAPTER - WAVE MOTIONEXERCISE - PRACTICE SET (EXERCISE-II (LEVEL-II(ADVANCED) MATRIX MATCHING TYPE QUESTIONS))
1 Videos
- WAVE MOTIONBOOK - AAKASH SERIESCHAPTER - WAVE MOTIONEXERCISE - PRACTICE SET (EXERCISE-V (LEVEL-I(MAIN) STRAIGHT OBJECTIVE TYPE QUESTIONS))
15 Videos
- WAVE MOTIONBOOK - AAKASH SERIESCHAPTER - WAVE MOTIONEXERCISE - PRACTICE SET (EXERCISE-III (LEVEL-II(ADVANCED) INTEGER TYPE QUESTIONS))
2 Videos
- UNITS AND MEASUREMENTSBOOK - AAKASH SERIESCHAPTER - UNITS AND MEASUREMENTSEXERCISE - EXERCISE -3
66 Videos
- WAVE MOTION AND SOUNDBOOK - AAKASH SERIESCHAPTER - WAVE MOTION AND SOUNDEXERCISE - PROBLEMS (LEVEL - II)
97 Videos
Similar Questions
Knowledge Check
Assertion : Current versus potential difference (i-V) graph for a conductor at two different temperatures T1 and T2 is shown in figure. Hence T1>T2.
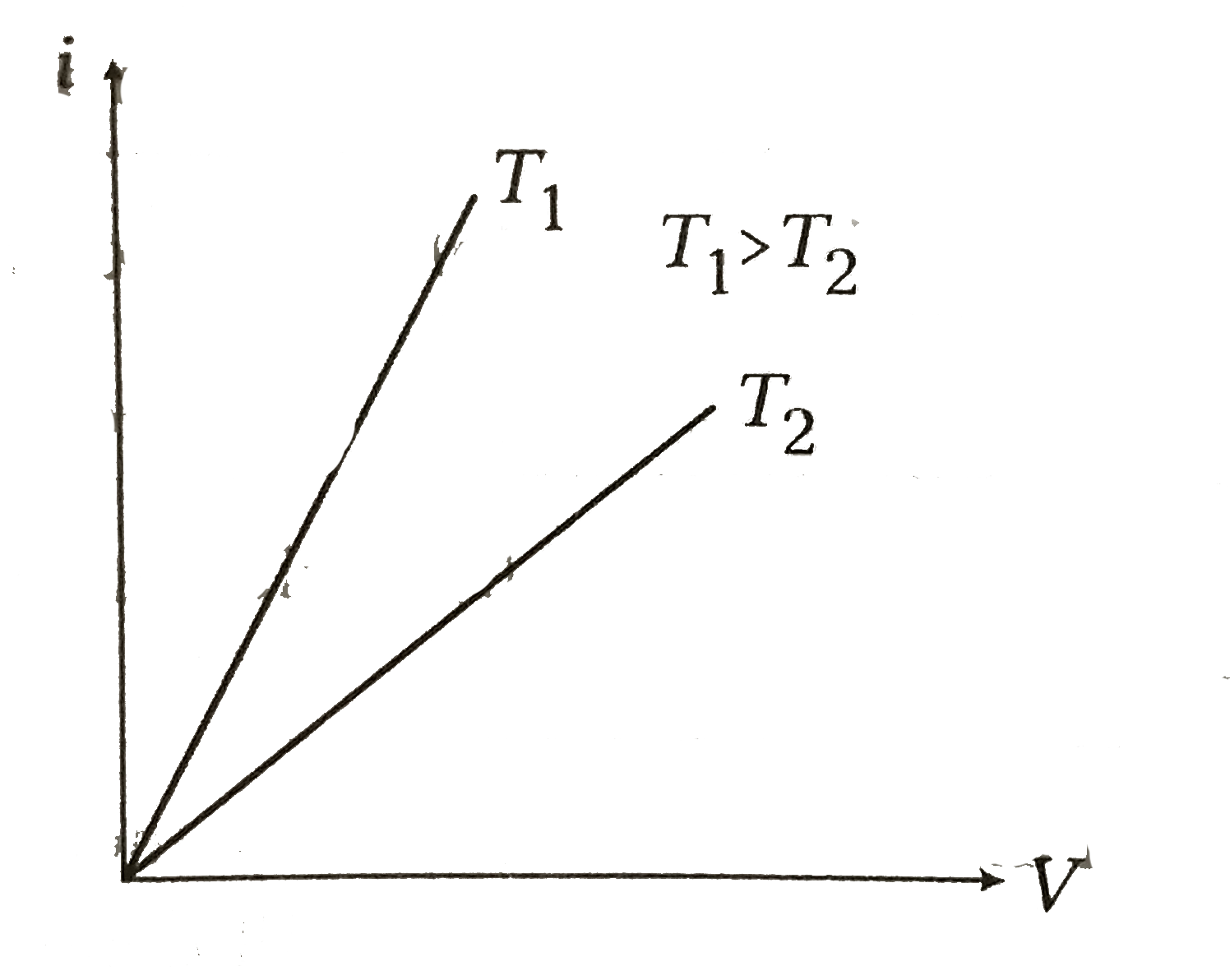
Reason : Resistance of a conductor increases with rise in temperature.Acos2θBsin2θCcot2θDtan2θ
Two bodies of masses (m1) and (m2) are droppded from heithts h1 and h2, respectively. They reach the ground after time t1 and t2 and strike the ground with v1 and v2, respectively Choose the correct relations from the following.
View SolutionThe area of hole (1) is A1 and of hole (2) is A2. The velocity of efflux and range of liquid is v1,v2andx1,x2 for holes (1) and (2) respectively. The time taken by liquid, jet to reach the ground from holes (1) and (2) are t1andt2 respectively. Cross section of tank is very very large compared to size of holes.
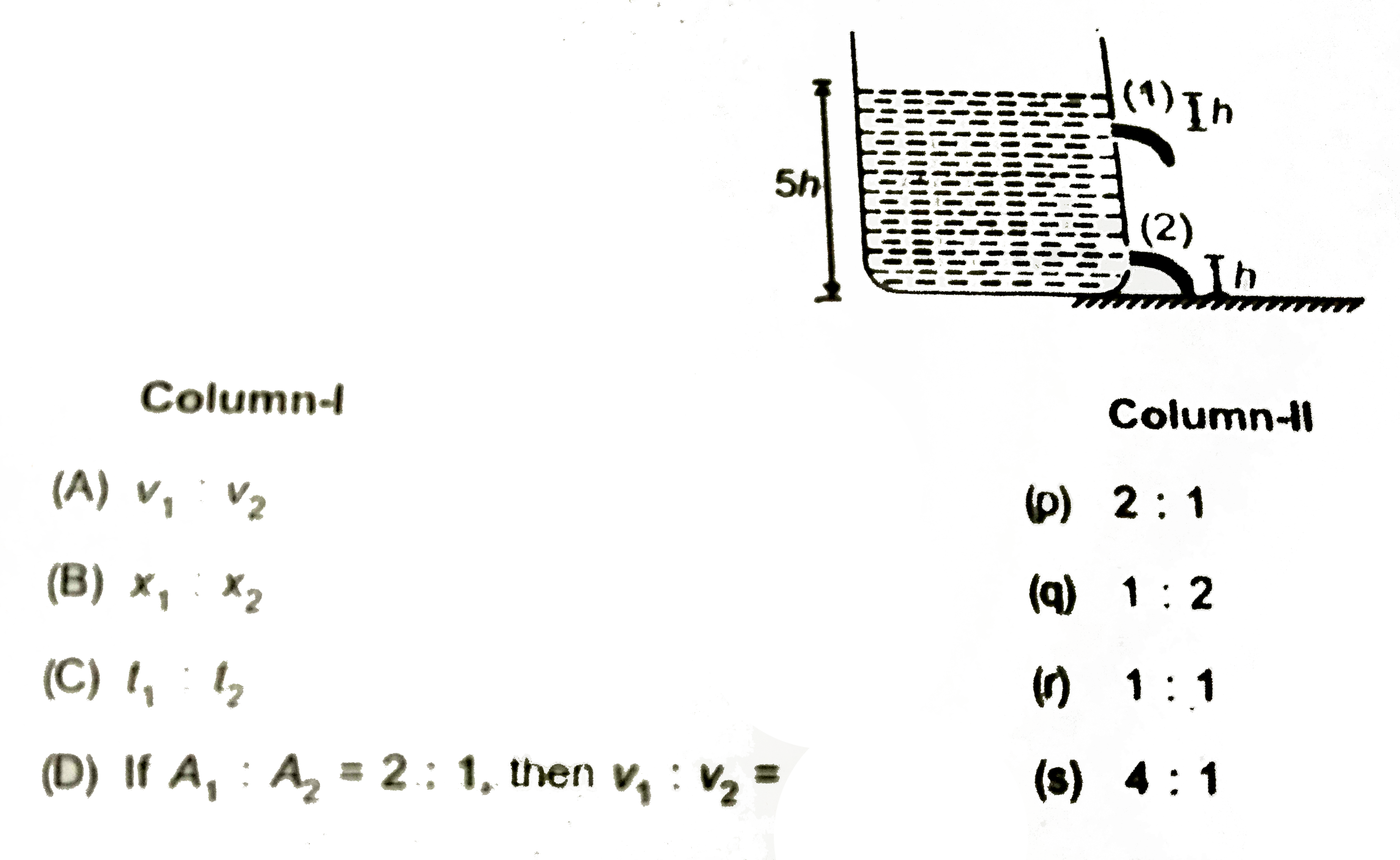
Column-IColumn-II(A)v1:v2(p)2:1(B)x1:x2(q)1:2(C)t1:t2(r)1:1(D)ifA1:A2=2:1,thenv1:v2=(s)4:1
View SolutionThree isothermal plots (P versus V) A, B and C are plotted at three temperature T1,T2andT3 respectively
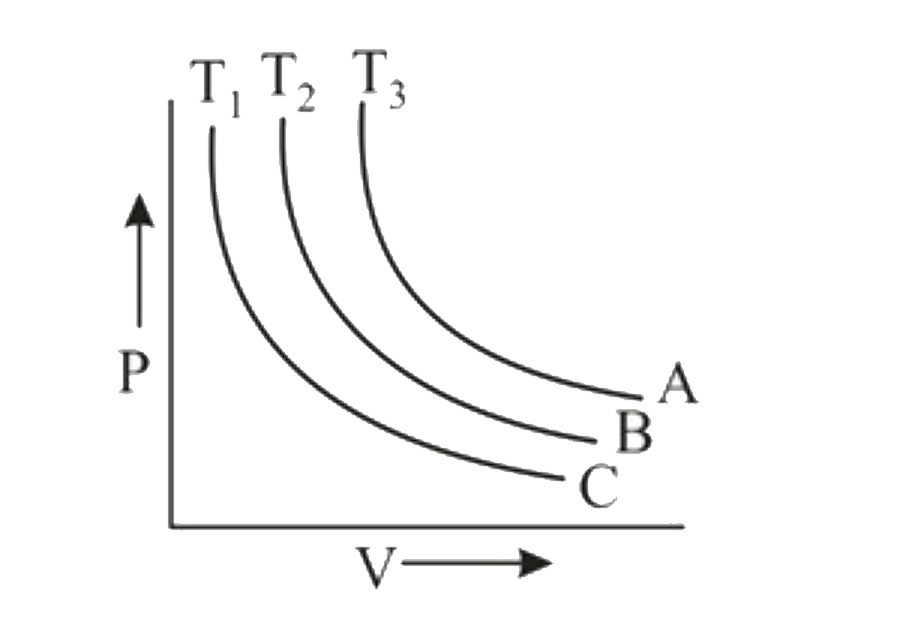
The correct order of the temperature will be
View SolutionTwo cars of same mass are moving with velocities v1andv2 respectively. If they are stopped by supplying same breaking power in time t1andt2 respectively then v1v2 is
View Solutionv1andv2 are the velocities of sound at the same temperature in two monoatomic gases of densities ρ1andρ2 respectively. If ρ1ρ2=14 then the ratio of velocities v1andv2 is
View SolutionThe velocities of sound at same temperature in two monoatomic gases densities ρ1 and ρ2 are v1 and v2 repectively , if ρ1ρ2=4, then the value of v1v2 will be
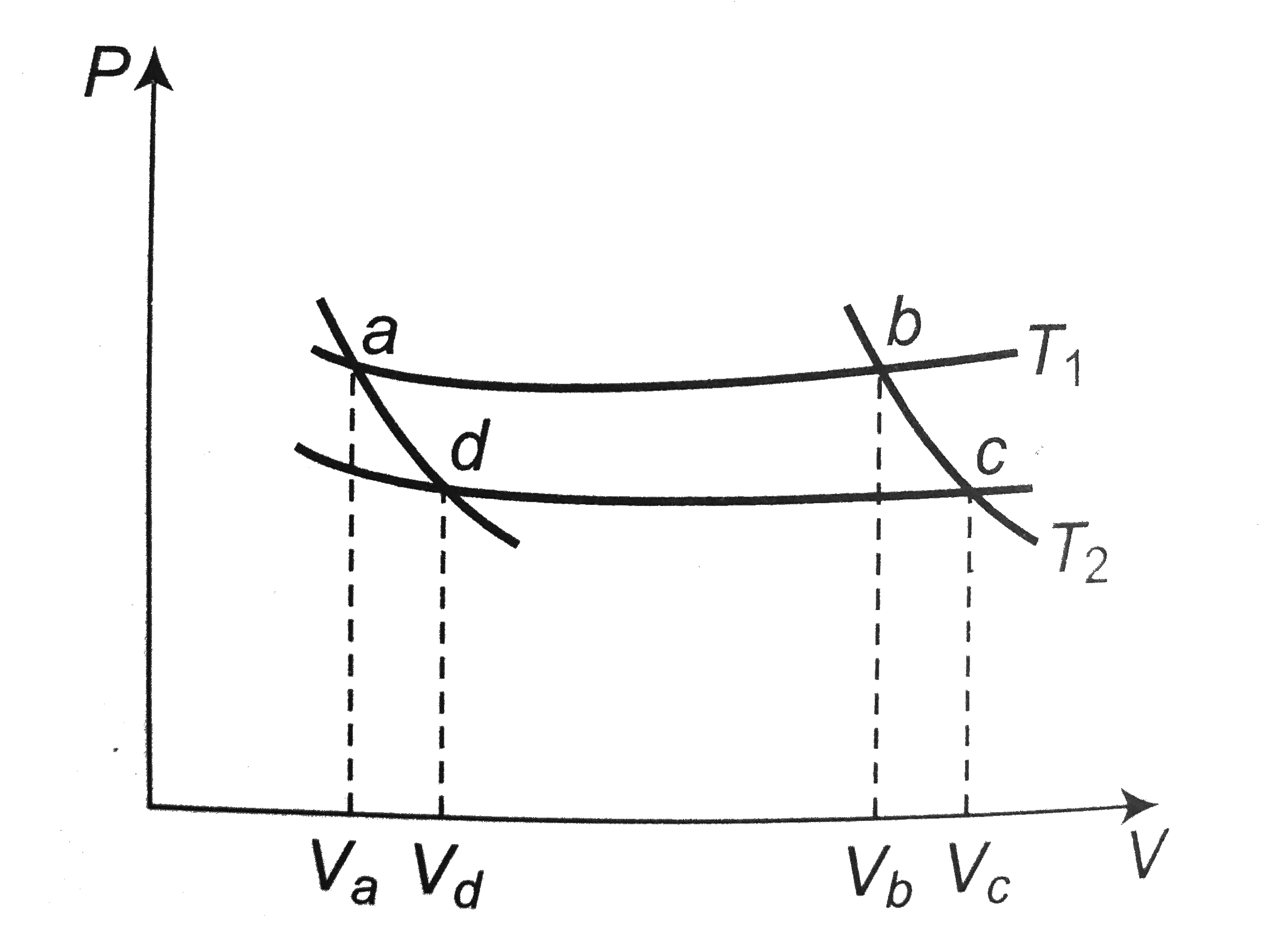
View SolutionIn the following P-V diagram two adiabatics cut two isothermals at temperature T1andT2 (fig). The value of VaVd will be
View Solution
