A unifrom rope of mass 0.1kg and length 2.45 m hangs from a rigid support. The time taken by the transverse wave formed in the rope to travel through the full length of the rope is (Assume g==9.8m/s2)
The correct Answer is:1
To solve the problem, we will follow these steps:
Step 1: Calculate the mass per unit length (μ) of the rope.
Given:
- Mass of the rope (m) = 0.1 kg
- Length of the rope (l) = 2.45 m
The mass per unit length (μ) is calculated as:
μ=ml=0.1kg2.45m≈0.04082kg/m
Step 2: Calculate the tension (T) in the rope.
The tension in the rope due to its weight is given by:
T=m⋅g
Where:
- g=9.8m/s2
Substituting the values:
T=0.1kg⋅9.8m/s2=0.98N
Step 3: Calculate the speed (v) of the wave in the rope.
The speed of the wave is given by the formula:
v=√Tμ
Substituting the values of T and μ:
v=√0.98N0.04082kg/m≈√24.06≈4.90m/s
Step 4: Calculate the time taken (t) for the wave to travel the length of the rope.
The time taken for the wave to travel the full length of the rope is given by:
t=lv
Substituting the values:
t=2.45m4.90m/s≈0.5s
Final Answer:
The time taken by the transverse wave to travel through the full length of the rope is approximately 0.5 seconds.
---
Given:
- Mass of the rope (m) = 0.1 kg
- Length of the rope (l) = 2.45 m
μ=ml=0.1kg2.45m≈0.04082kg/m
The tension in the rope due to its weight is given by:
T=m⋅g
Where:
- g=9.8m/s2
T=0.1kg⋅9.8m/s2=0.98N
The speed of the wave is given by the formula:
v=√Tμ
v=√0.98N0.04082kg/m≈√24.06≈4.90m/s
The time taken for the wave to travel the full length of the rope is given by:
t=lv
t=2.45m4.90m/s≈0.5s
The time taken by the transverse wave to travel through the full length of the rope is approximately 0.5 seconds.
Topper's Solved these Questions
- WAVE MOTIONBOOK - AAKASH SERIESCHAPTER - WAVE MOTIONEXERCISE - PRACTICE SHEET ADVANCED (MORE THAN ON CORRECT ANSWER TYPE QUESTIONS)
2 Videos
- WAVE MOTIONBOOK - AAKASH SERIESCHAPTER - WAVE MOTIONEXERCISE - PRACTICE SHEET ADVANCED (LINKED COMPREHENSION TYPE QUESTIONS)
9 Videos
- WAVE MOTIONBOOK - AAKASH SERIESCHAPTER - WAVE MOTIONEXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL-II(LACTURE SHEET ADVANCED) LINKED COMPREHENSION TYPE QUESTIONS))
9 Videos
- UNITS AND MEASUREMENTSBOOK - AAKASH SERIESCHAPTER - UNITS AND MEASUREMENTSEXERCISE - EXERCISE -3
66 Videos
- WAVE MOTION AND SOUNDBOOK - AAKASH SERIESCHAPTER - WAVE MOTION AND SOUNDEXERCISE - PROBLEMS (LEVEL - II)
97 Videos
Similar Questions
A uniform rope of mass 0.1kg and length 2.45m hangs from a ceiling.
(a) Find the speed of transverse wave in the rope at a point 0.5m distant from the lower end.
(b) Calculate the time taken by a transverse wave to travel the full length of the rope.

View SolutionA uniform rope of mass m and length L hangs from a celling. (a) Show that the speed of a transverse wave on the rope is a function of y, the distance from the lower end, and is given by t=2√L/g.
View Solution
Knowledge Check
A uniform rope of length 12 mm and mass 6 kg hangs vertically from a rigid support. A block of mass 2 kg is attached to the free end of the rope. A transverse pulse of wavelength 0.06 m is produced at the lower end of the rope. What is the wavelength of the pulse when it reaches the top of the rope?
A0.06 mB0.03 mC0.12 mD0.09 m
A uniform rope of mass M=0.1kg and length L=10m hangs from the celling. [g=10m/s2] :- (1)speed of transverse wave in the rope increases linearly from top to the bottom. (2)speed of the transverse wave in the rope decreases linearly from bottom to the top. (3)speed of the transverse wave in the rope remain constant along the length of the rope (4)time taken by the transverse wave to travel the full length of the rope is 2 sec.
View SolutionA thick uniform rope of length L is hanging from a rigid support. A transverse wave of wavelength λ0 is set up in the middle of the rope. The wavelength of the wave as it reaches the top most point is
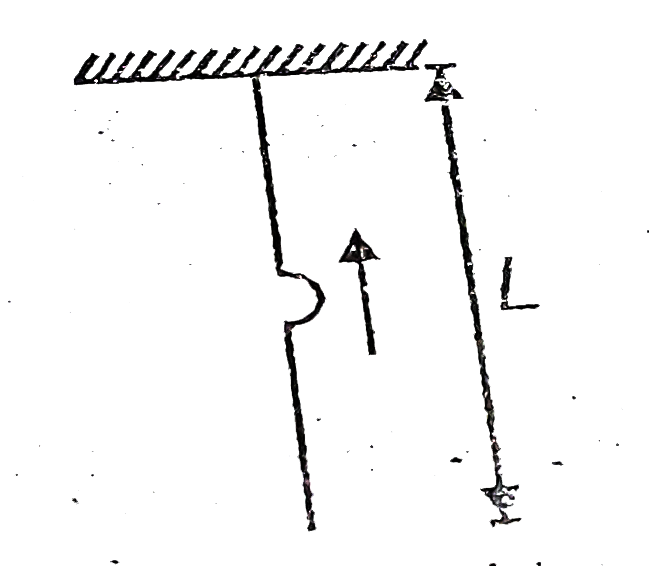
View SolutionA uniform rope of mass m per unit length, hangs vertically from a support so that the lower end just touches the table top shown in figure. If it is released, show that at the time a length y of the rope has fallen, the force on the table is equivalent to the weight of the length 3y of the rope.
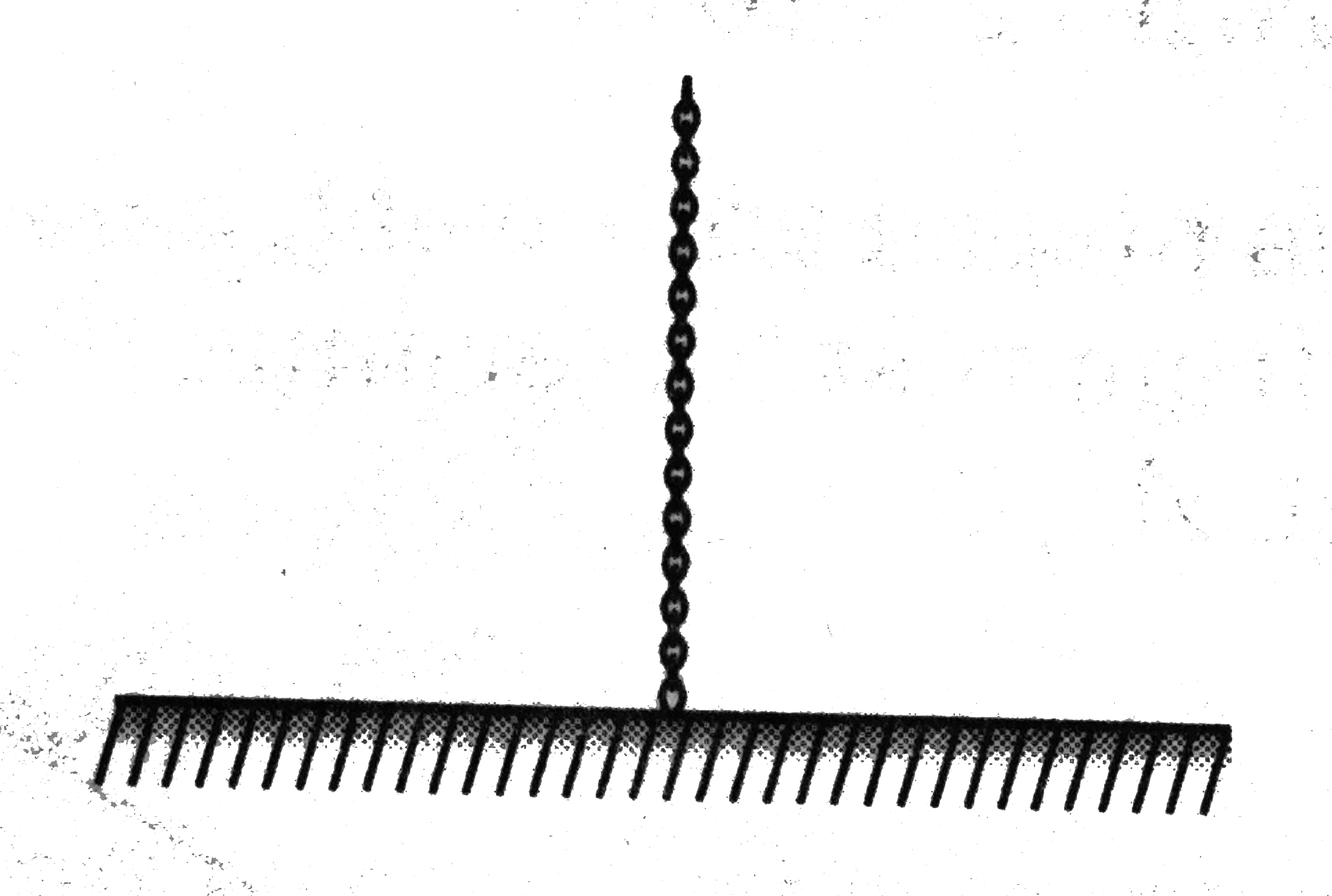
View SolutionA Uniform rope having mass m hags vertically from a rigid support. A transverse wave pulse is produced at the lower end. The speed v of wave pulse varies with height h from the lower end as
View SolutionA uniform rope of length 12 mm and mass 6 kg hangs vertically from a rigid support. A block of mass 2 kg is attached to the free end of the rope. A transverse pulse of wavelength 0.06 m is produced at the lower end of the rope. What is the wavelength of the pulse when it reaches the top of the rope?
View SolutionA uniform rope of length 12 mm and mass 6 kg hangs vertically from a rigid support. A block of mass 2 kg is attached to the free end of the rope. A transverse pulse of wavelength 0.06 m is produced at the lower end of the rope. What is the wavelength of the pulse when it reaches the top of the rope?
View SolutionA uniform rope of length 10 cm and mass 4 kg hangs vertically from a rigid support. A block of mass 2 kg is attached to the free end of the rope. A transverse pulse of wavelength 0.06 m is produced at the lower end of the rope, what is the wavelength of the pulse when it reaches the top of the rope?
View Solution
