A person looking through a telescope focuses lens at a point on the edge of the bottom of an empty cylindrical vessel. Next he fills the entire vessel with a liquid oif refractive index μ, without disturbing the telescope. Now, he observes the mid point of the vessel. Determine the radius to depth ratio of the vessel
12√1−μ2μ2+1
12√4−μ2μ2−1
12√4+μ2μ2+1
12√4+μμ+1
The correct Answer is:B
To solve the problem, we need to determine the radius to depth ratio of the cylindrical vessel after it has been filled with a liquid of refractive index μ. Here’s a step-by-step breakdown of the solution:
Step 1: Understand the Geometry
- Consider a cylindrical vessel with depth h and radius r.
- The person is looking through a telescope focused on the edge of the bottom of the vessel. When the vessel is filled with liquid, the light rays will bend due to refraction.
Step 2: Identify the Angles
- Let i be the angle of incidence when light travels from air to the liquid.
- Let r be the angle of refraction in the liquid.
- According to Snell's Law, we have:
n1sin(i)=n2sin(r)
where n1=1 (refractive index of air) and n2=μ (refractive index of the liquid).
Step 3: Set Up the Geometry
- The light ray that is incident at the edge of the vessel will create a right triangle with the following sides:
- The opposite side (perpendicular) is 2r (the diameter of the vessel).
- The adjacent side (base) is √(2r)2+h2.
Step 4: Apply Trigonometric Relationships
- From the geometry, we can express sin(i) and sin(r):
sin(i)=2r√(2r)2+h2
sin(r)=r√r2+h2
Step 5: Apply Snell's Law
- Substituting into Snell's Law:
1⋅2r√(2r)2+h2=μ⋅r√r2+h2
Step 6: Cross Multiply and Simplify
- Cross-multiplying gives:
2r√r2+h2=μr√(2r)2+h2
- Dividing both sides by r (assuming r≠0):
2√r2+h2=μ√4r2+h2
Step 7: Square Both Sides
- Squaring both sides results in:
4(r2+h2)=μ2(4r2+h2)
- Expanding gives:
4r2+4h2=4μ2r2+μ2h2
Step 8: Rearranging the Equation
- Rearranging terms leads to:
4h2−μ2h2=4μ2r2−4r2
- Factoring out common terms:
h2(4−μ2)=4r2(μ2−1)
Step 9: Solve for the Radius to Depth Ratio
- Dividing both sides by h2 gives:
r2h2=4−μ24(μ2−1)
- Taking the square root:
rh=12√4−μ2μ2−1
Final Result
- Therefore, the radius to depth ratio of the vessel is:
rh=12√4−μ2μ2−1
- Consider a cylindrical vessel with depth h and radius r.
- The person is looking through a telescope focused on the edge of the bottom of the vessel. When the vessel is filled with liquid, the light rays will bend due to refraction.
- Let i be the angle of incidence when light travels from air to the liquid.
- Let r be the angle of refraction in the liquid.
- According to Snell's Law, we have:
n1sin(i)=n2sin(r)
where n1=1 (refractive index of air) and n2=μ (refractive index of the liquid).
- The light ray that is incident at the edge of the vessel will create a right triangle with the following sides:
- The opposite side (perpendicular) is 2r (the diameter of the vessel).
- The adjacent side (base) is √(2r)2+h2.
- From the geometry, we can express sin(i) and sin(r):
sin(i)=2r√(2r)2+h2
sin(r)=r√r2+h2
- Substituting into Snell's Law:
1⋅2r√(2r)2+h2=μ⋅r√r2+h2
- Cross-multiplying gives:
2r√r2+h2=μr√(2r)2+h2
- Dividing both sides by r (assuming r≠0):
2√r2+h2=μ√4r2+h2
- Squaring both sides results in:
4(r2+h2)=μ2(4r2+h2)
- Expanding gives:
4r2+4h2=4μ2r2+μ2h2
- Rearranging terms leads to:
4h2−μ2h2=4μ2r2−4r2
- Factoring out common terms:
h2(4−μ2)=4r2(μ2−1)
- Dividing both sides by h2 gives:
r2h2=4−μ24(μ2−1)
- Taking the square root:
rh=12√4−μ2μ2−1
- Therefore, the radius to depth ratio of the vessel is:
rh=12√4−μ2μ2−1
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - LECTURE SHEET (EXERCISE II LEVEL -II (ADVANCED) MORE THAN ONE CORRECT TYPE QUESTIONS)7videos
GEOMETRICAL OPTICS
- BOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - LECTURE SHEET (EXERCISE II LEVEL -II (ADVANCED) LINKED COMPREHENSION TYPE QUESTIONS)9videos
GEOMETRICAL OPTICS
- BOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - LECTURE SHEET (EXERCISE II LEVEL -I (MAIN) STRAIGHT OBJECTIVE TYPE QUESTIONS)17videos
GEOMETRICAL OPTICS
- BOOK - AAKASH SERIESCHAPTER - ELEMENTS OF VECTORSEXERCISE - QUESTIONS FOR DESCRIPTIVE ANSWERS10videos
ELEMENTS OF VECTORS
- BOOK - AAKASH SERIESCHAPTER - LAWS OF MOTIONEXERCISE - PRACTICE EXERCISE106videos
LAWS OF MOTION
Similar Questions
A person just observes the point A on the circumference at the bottom of an empty cylindricla vessel of diameter 10 cm . When the vessel is completely filled with a liquid (μ=√2), he can just see the centre B of the bottom. Then the height of the vessel is
 View Solution
View SolutionA vessel of depth 2d cm is half filled with a liquid of refractive index μ1 and the upper half with a liquid of refractive index μ2. The apparent depth of the vessel seen perpendicularly is
View SolutionA small object is placed at the centre of the bottom of a cylindrical vessel of radius 3 cm and height 4 cm filled completely with water. Consider the ray leaving the vessel through a corner. Suppose this ray and the ray along the axis of the vessel are used to trace the image. Find the apparent depth of the image and the ratio of real depth to the apparent depth under the assumptions taken. Refractive index -of water = 1-33.
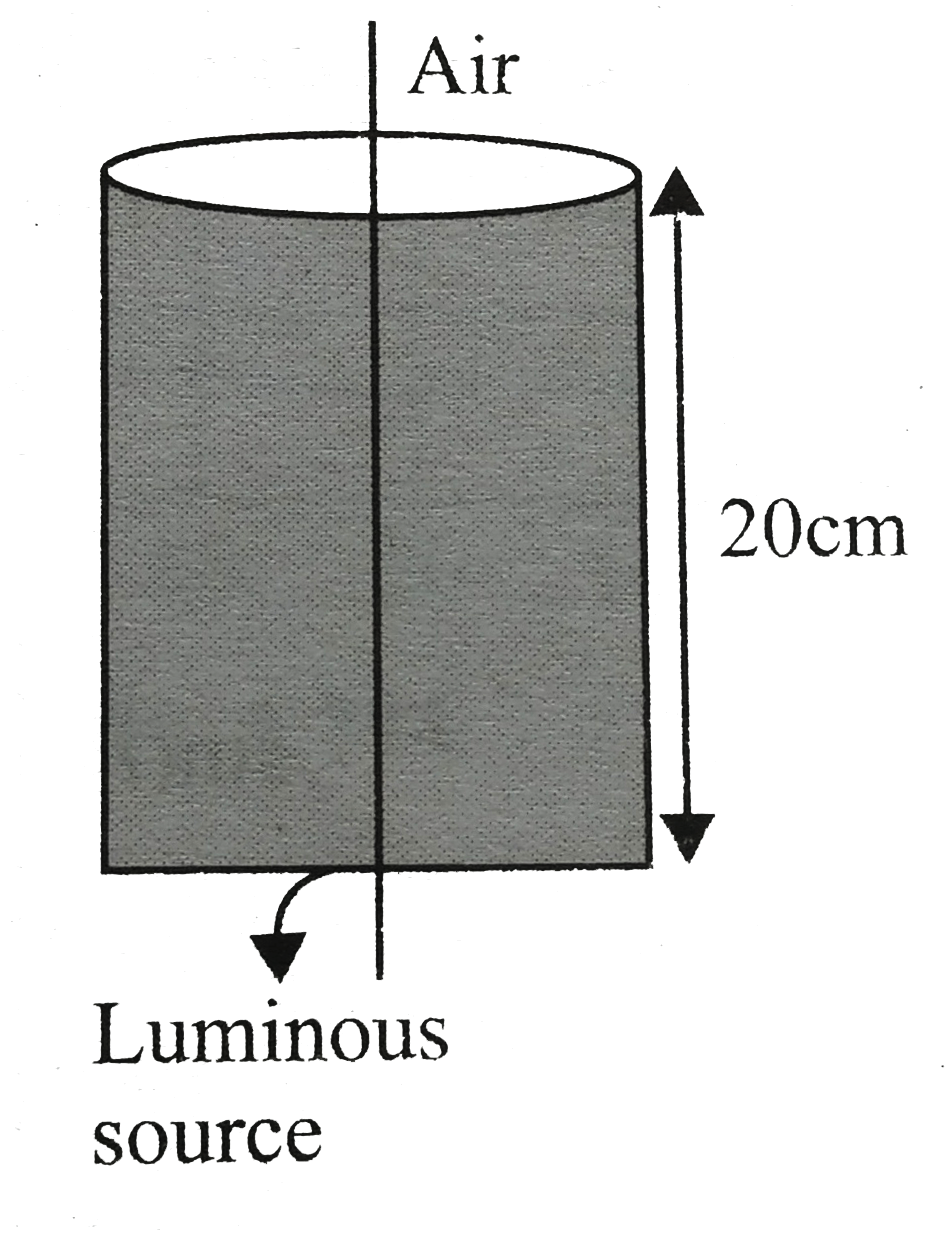
View SolutionA thin equiconvex glass lens (μg=1.5) is beign placed on the top of a vessel of height h=20cm as shown figure. A luminous point source is beign placed at the bottom of vessel on the principal axis of the lens. When the air is on both the side of the lens the image of luminous source is formed at a distance of 20cm from the lens out side the vessel. When the air inside the vessel is being replaced by a liquid of refractive index μl the image of the same source is being formed at a distance 30cm from the lens outside the vessel. Find the μl.
 View Solution
View SolutionA vessle of depth d is first filled to (23)rd of its depth with a liquid of refractive index 32 and the rest of the vessel is filled with a liquid of refractive index 43. What is the apparent depth of the inner surface of the bottom of the vessle.
View SolutionA vessle of depth d is first filled to (23)rd of its depth with a liquid of refractive index 32 and the rest of the vessel is filled with a liquid of refractive index 43. What is the apparent depth of the inner surface of the bottom of the vessle.
View SolutionA vessel is quarter filled with a liquid of refractive index μ. The remaining parts of the vessel is filled with an immiscible liquid of refractive index 3μ/2. The apparent depth of the vessel is 50% of the actual depth. The value of μ is
View SolutionIn the previous problem what will be refractive index of the liquid so that point E is visible (PE=R/3) through the telescope in air when the vessel is filled with liquid completely, without moving the telesope and vessel.
View SolutionA vessel of depth 2h is half filled with a liquid of refractive index 2√2 and the upper half with another liquid of refractive index √2. The liquids are immiscible. The apparent depth of the inner surface of the bottom of vessel will be:
View SolutionA point source of light S is placed at the bottom of a vessel containg a liquid of refractive index 5/3. A person is viewing the source from above the surface. There is an opaque disc of radius 1cm floating on the surface. The centre of the disc lies vertically above the source S. The liquid from the vessel is gradually drained out through a tap. What is the maximum height of the liquid for which the source cannot at all be seen from above?
View Solution

