The frequency distribution of the marks obtained by 100 students in a test carrying 50 marks is given below. Then the mean is
Marks 0−910−1920−2930−3940−49No. of students 8 15 20 45 12
28.3
28
27.3
26.4
The correct Answer is:A
To find the mean of the frequency distribution of marks obtained by 100 students, we will follow these steps:
Step 1: Create a Frequency Table
We will create a table with the midpoints (x_i), frequencies (f_i), and the product of midpoints and frequencies (x_i * f_i).
| Marks | No. of Students (f_i) | Midpoint (x_i) | f_i * x_i |
|------------|-----------------------|----------------|-----------|
| 0 - 9 | 8 | 4.5 | 36 |
| 10 - 19 | 15 | 14.5 | 217.5 |
| 20 - 29 | 20 | 24.5 | 490 |
| 30 - 39 | 45 | 34.5 | 1552.5 |
| 40 - 49 | 12 | 44.5 | 534 |
| Total | 100 | | 2830 |
Step 2: Calculate the Midpoints (x_i)
To find the midpoints for each class interval, we use the formula:
xi=Lower limit+Upper limit2
- For 0 - 9: x1=0+92=4.5
- For 10 - 19: x2=10+192=14.5
- For 20 - 29: x3=20+292=24.5
- For 30 - 39: x4=30+392=34.5
- For 40 - 49: x5=40+492=44.5
Step 3: Calculate the Product of Frequencies and Midpoints (f_i * x_i)
Now we multiply the frequency (f_i) by the corresponding midpoint (x_i):
- For 0 - 9: 8×4.5=36
- For 10 - 19: 15×14.5=217.5
- For 20 - 29: 20×24.5=490
- For 30 - 39: 45×34.5=1552.5
- For 40 - 49: 12×44.5=534
Step 4: Calculate the Summation of f_i and f_i * x_i
Next, we sum up the frequencies and the products:
- ∑fi=8+15+20+45+12=100
- ∑(fi⋅xi)=36+217.5+490+1552.5+534=2830
Step 5: Calculate the Mean
Finally, we use the formula for the mean:
Mean=∑(fi⋅xi)∑fi
Substituting the values we calculated:
Mean=2830100=28.3
Conclusion
The mean of the frequency distribution is 28.3.
---
We will create a table with the midpoints (x_i), frequencies (f_i), and the product of midpoints and frequencies (x_i * f_i).
|------------|-----------------------|----------------|-----------|
| 0 - 9 | 8 | 4.5 | 36 |
| 10 - 19 | 15 | 14.5 | 217.5 |
| 20 - 29 | 20 | 24.5 | 490 |
| 30 - 39 | 45 | 34.5 | 1552.5 |
| 40 - 49 | 12 | 44.5 | 534 |
| Total | 100 | | 2830 |
To find the midpoints for each class interval, we use the formula:
xi=Lower limit+Upper limit2
- For 10 - 19: x2=10+192=14.5
- For 20 - 29: x3=20+292=24.5
- For 30 - 39: x4=30+392=34.5
- For 40 - 49: x5=40+492=44.5
Now we multiply the frequency (f_i) by the corresponding midpoint (x_i):
- For 10 - 19: 15×14.5=217.5
- For 20 - 29: 20×24.5=490
- For 30 - 39: 45×34.5=1552.5
- For 40 - 49: 12×44.5=534
Next, we sum up the frequencies and the products:
- ∑(fi⋅xi)=36+217.5+490+1552.5+534=2830
Finally, we use the formula for the mean:
Mean=∑(fi⋅xi)∑fi
Mean=2830100=28.3
The mean of the frequency distribution is 28.3.
Similar Questions
If for somex∈R, the frequency distribution of the marks obtained by 20 students in a test is:
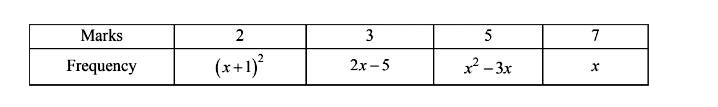
Then the mean of the marks is:
View SolutionMarks scored by 100 students in a 25 marks unit test of Mathematics is given below. Their median is
Marks 0−55−1010−1515−2020−25Students 10 18 42 23 7
View SolutionThe frequency distribution of marks obtained by 28 students in a test carrying 40 marks is given below:
Marks:0−1010−2020−3030−40Number of students:6xy6
If the mean of the above data is 20, then the difference between x and y is
View SolutionCompute the missing frequencies in the following distribution , given that is ∑fi = 100 and the median is 32
Marks0−1010−2020−3030−4040−5050−60Number of students 9 ? 26 30 ? 10
View SolutionDraw a cumulative frequency curve (ogive) for the following distribution
Mark 5−1010−1515−2020−2525−30 no. of students 7 9 12 8 6
View SolutionThe distribution given below, shows the marks obtained by 25 students in an aptitude test. Find the median and mode of the distribution.
View SolutionConstruct a frequency polygon for the following distribution, using a graph sheet :
Marks30−4040−5050−6060−7070−8080−90No. of students 6 15 28 34 18 8
View SolutionCalculate the mean devation from the mean the following data:
Marks0−1010−2020−3030−4040−50No. of stu.5815166
View SolutionThe marks obtained (out of 30) by five students in a test are 20, 15, 25, 18, and 22. Find the average marks obtained.
View SolutionFind the mean for the following data.
Marks obtained10−2020−3030−4040−5050−6060−70Number of students8612527
View Solution
