A metal rod of resistance R is fixed along a diameter of a conducting ring of radius r. There is a magnetic field of magnitude B perpendicular to the plane of the loop. The ring spins with an angular velocity ω about its axis. The centre of the ring is joined to its rim by an external wire W. The ring and W have no resistance. The current in W is
Zero
Br2ω2R
2Br2ωR
Br2ω2R
To solve the problem, we will follow these steps:
Step 1: Understand the Setup
We have a conducting ring of radius r with a metal rod of resistance R fixed along its diameter. The ring is spinning with an angular velocity ω in a magnetic field B that is perpendicular to the plane of the ring.
Step 2: Calculate the Induced EMF
When the ring spins in the magnetic field, an electromotive force (emf) is induced due to electromagnetic induction. The formula for the induced emf (E) in a rotating loop in a magnetic field is given by:
E=12Br2ω
where r is the radius of the ring, B is the magnetic field strength, and ω is the angular velocity.
Step 3: Determine the Resistance in the Circuit
The rod divides the ring into two halves, each half can be considered as a radius of the ring. Since the rod has a resistance R, and each half (radius) has a resistance of R2 (because the total resistance R is shared by two halves).
When considering the two halves in parallel, the equivalent resistance Req between the center and the rim of the ring is:
R_{eq} = \frac{R/2 \times R/2}{R/2 + R/2} = \frac{R/4}
Step 4: Calculate the Current
Using Ohm's Law, the current I flowing through the wire W can be calculated using the induced emf and the equivalent resistance:
I=EReq=12Br2ωR4=2Br2ωR
Final Answer
Thus, the current in the wire W is:
I=2Br2ωR
We have a conducting ring of radius r with a metal rod of resistance R fixed along its diameter. The ring is spinning with an angular velocity ω in a magnetic field B that is perpendicular to the plane of the ring.
When the ring spins in the magnetic field, an electromotive force (emf) is induced due to electromagnetic induction. The formula for the induced emf (E) in a rotating loop in a magnetic field is given by:
E=12Br2ω
where r is the radius of the ring, B is the magnetic field strength, and ω is the angular velocity.
The rod divides the ring into two halves, each half can be considered as a radius of the ring. Since the rod has a resistance R, and each half (radius) has a resistance of R2 (because the total resistance R is shared by two halves).
R_{eq} = \frac{R/2 \times R/2}{R/2 + R/2} = \frac{R/4}
Using Ohm's Law, the current I flowing through the wire W can be calculated using the induced emf and the equivalent resistance:
I=EReq=12Br2ωR4=2Br2ωR
Thus, the current in the wire W is:
I=2Br2ωR
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBook:AAKASH SERIESChapter:ELECTROMAGNETIC INDUCTIONExercise:EXERCISE - I (MAGNETIC FLUX, FARADAY.S LAW, LENZ.S LAW, AC GENERATOR)
Explore 26 Videos
- ELECTROMAGNETIC INDUCTIONBook:AAKASH SERIESChapter:ELECTROMAGNETIC INDUCTIONExercise:EXERCISE - I (SELF AND MUTUAL INDUCTION)
Explore 16 Videos
- ELECTROMAGNETIC INDUCTIONBook:AAKASH SERIESChapter:ELECTROMAGNETIC INDUCTIONExercise:EXERCISE-II
Explore 32 Videos
- ELECTRIC FIELD AND POTENTIALBook:AAKASH SERIESChapter:ELECTRIC FIELD AND POTENTIALExercise:PROBLEMS (LEVEL-II)
Explore 26 Videos
- ELECTROMAGNETIC WAVESBook:AAKASH SERIESChapter:ELECTROMAGNETIC WAVESExercise:EXERCISE -II
Explore 22 Videos
Similar Questions
A metal rod of resistance 20Ω is fixed along a diameter of a conducting ring of radius 0.1m and lies on x−y plane. There is a magnetic field →B=(50T)→k. The ring rotates with an angular velocity ω=20rads−1 about its axis. An external resistance of 10Ω is connected across the center of the ring and rim. The current external resistance is
View SolutionA vertical conducting ring ogradius R falls vertically in a horizontal magnetic field of magnitude B. The direction of B is perpendicular to the plane of the ring. When the speed of the ring is v,
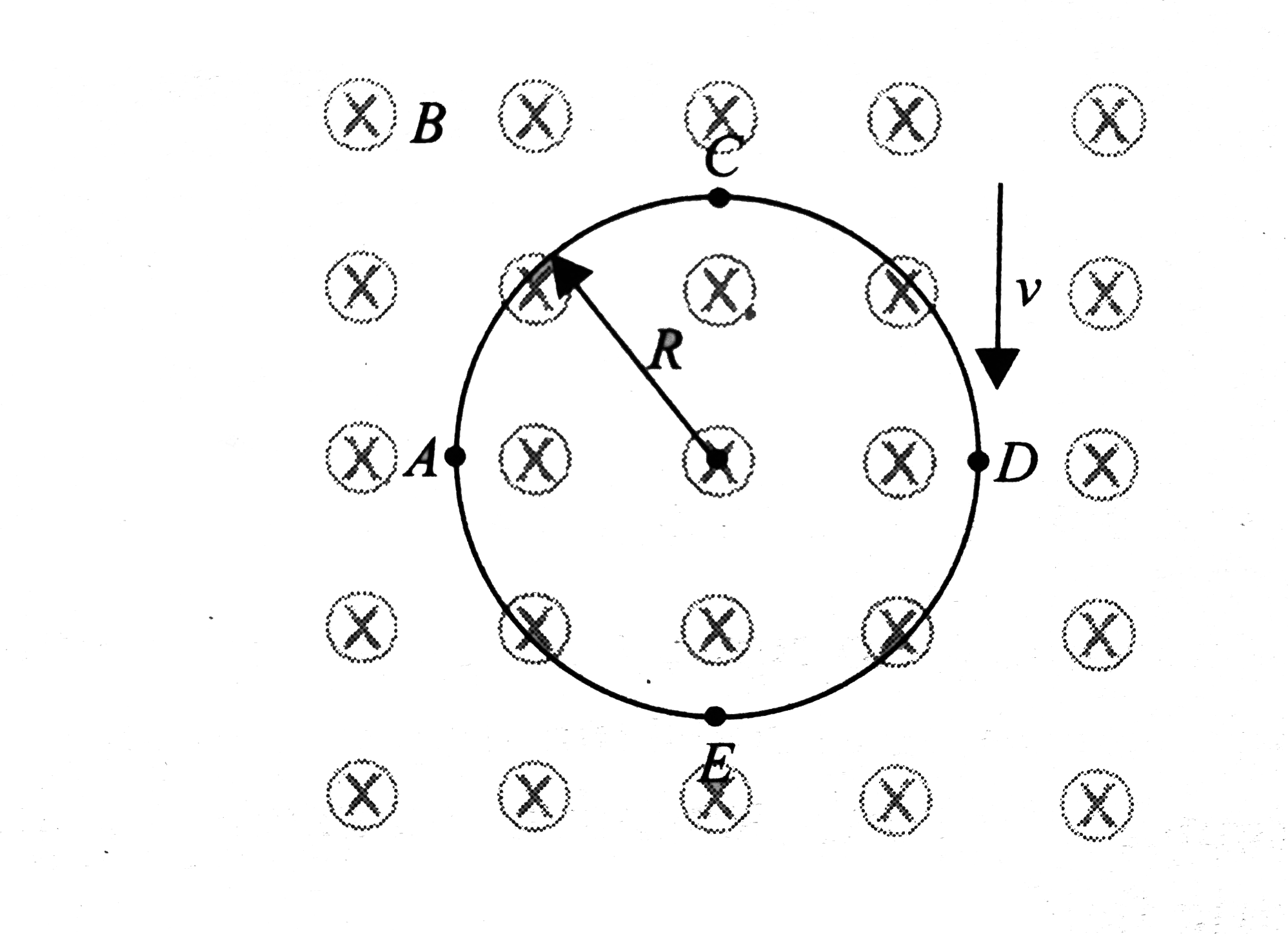
View Solution
Knowledge Check
A conducting ring is placed in a uniform magnetic field with its plane perpendicular to the field . An emf is induced in the ring if
Ait is rotated about its axis.Bit is rotated about its diameter.Cit is not moved.Dit is given translational motion in the field.
A ring of mass m and radius r is rotated in uniform magnetic field B which is perpendicular to the plane of the loop with constant angular velocity ω0.Find the net ampere force on the ring and the tension developed in the ring if there is a current i in the ring.Current and rotation both are clockwise.
View SolutionThe figure shows a conducting ring of radius R. A uniform steady magnetic field B lies perpendicular to the plane of the ring a circular region r(<R). If the resistance per unit length of the ring is λ, then the current induced in the ring when its radius gets doubled is

View SolutionA conducting ring of radius r having charge q is rotating with angular velocity ω about its axes. Find the magnetic field at the centre of the ring.

View SolutionA conducting ring of radius r having charge q is rotating with angular velocity ω about its axes. Find the magnetic field at the centre of the ring.

View SolutionA circular conducting ring is rotated about one of its diameter in a magnetic field
View SolutionA thin circular ring of mass m and radius R is rotating about its axis perpendicular to the plane of the ring with a constant angular velocity ω. Two point particleseach of mass M are attached gently to the opposite end of a diameter of the ring. The ring now rotates, with an angular velocityω2. Then the ratiomM is
View SolutionA ring of mass m and radius r rotates about an axis passing through its centre and perpendicular to its plane with angular velocity ω. Its kinetic energy is
View Solution
