A hollow spherical conductor of radius 1m has a charge of 250μC then electric intensity at a point distance of 0.5m from the centre of the spherical conductor is
zero
2.25×106N/C
4.5×104N/C
9×104N/C
The correct Answer is:A
To solve the problem of finding the electric intensity at a point 0.5 m from the center of a hollow spherical conductor with a charge of 250 µC, we can follow these steps:
1. Understand the Geometry and Charge Distribution:
- We have a hollow spherical conductor with a radius of 1 m and a total charge of 250 µC.
- The point of interest is located at a distance of 0.5 m from the center of the sphere.
2. Apply Gauss's Law:
- Gauss's Law states that the electric field (E) times the area (A) of a closed surface is equal to the charge (Q) enclosed by that surface divided by the permittivity of free space (ε₀):
ΦE=E⋅A=Qencϵ0
3. Determine the Enclosed Charge:
- Since the point at 0.5 m is inside the hollow conductor (the radius of the conductor is 1 m), the charge enclosed (Q_enc) is zero. This is because the charge resides on the surface of the conductor, and there is no charge within the hollow part.
4. Calculate the Electric Field:
- Since the enclosed charge (Q_enc) is zero, we can substitute this into Gauss's Law:
E⋅A=0ϵ0
- This implies that:
E⋅A=0⟹E=0
- Therefore, the electric field intensity at a point 0.5 m from the center of the hollow spherical conductor is 0.
5. Conclusion:
- The electric intensity at a distance of 0.5 m from the center of the hollow spherical conductor is 0 N/C.
Final Answer:
The electric intensity at a point 0.5 m from the center of the hollow spherical conductor is 0 N/C.
- We have a hollow spherical conductor with a radius of 1 m and a total charge of 250 µC.
- The point of interest is located at a distance of 0.5 m from the center of the sphere.
- Gauss's Law states that the electric field (E) times the area (A) of a closed surface is equal to the charge (Q) enclosed by that surface divided by the permittivity of free space (ε₀):
ΦE=E⋅A=Qencϵ0
- Since the point at 0.5 m is inside the hollow conductor (the radius of the conductor is 1 m), the charge enclosed (Q_enc) is zero. This is because the charge resides on the surface of the conductor, and there is no charge within the hollow part.
- Since the enclosed charge (Q_enc) is zero, we can substitute this into Gauss's Law:
E⋅A=0ϵ0
- This implies that:
E⋅A=0⟹E=0
- Therefore, the electric field intensity at a point 0.5 m from the center of the hollow spherical conductor is 0.
- The electric intensity at a distance of 0.5 m from the center of the hollow spherical conductor is 0 N/C.
The electric intensity at a point 0.5 m from the center of the hollow spherical conductor is 0 N/C.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Exercise-II56videos
ELECTRIC CHARGES AND FIELDS
- BOOK - AAKASH SERIESCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - PRACTICE EXERCISEX42videos
DUAL NATURE OF RADIATION AND MATTER
- BOOK - AAKASH SERIESCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - PROBLEMS (LEVEL-II)26videos
ELECTRIC FIELD AND POTENTIAL
Similar Questions
A spherical shell of radius 10 cm is carrying a charge q. If the electric potential at distances 5 cm, 10 cm and 15 cm from the centre of the spherical shell is V1,V2 and V3 respectively, then
View SolutionA hollow sphere of radius 0.1 m has a charge of 5×10−8C. The potential at a distance of 5 cm from the centre of the sphere is
(14πε0=9×109Nm2C−2)View SolutionA spherical conductor of radius 10 cm has a charge of 3.2 x 10^-7 C distributed uniformly. What is magnitude of electric field at point 15 cm from centre of sphere?
View SolutionA charged disc of radius 3 m has charge density σ. Electric field at a distance of 4 m from its centre on axis is _______
View SolutionA thin spherical shell of metal has a radius of 0.25 m and carries charge of 0.2 micro coulomb. Calculate the electric intensity at 3.0 m from the centre of the shell.
View SolutionIf a charged spherical conductor of radius 10cm has potential V at a point distant 5cm from its centre, then the potential at a point distant 15cm from the centre will be
View SolutionIf a charged spherical conductor of radius 5 cm has potential V at a point distant 5 cm its centre, then the potential at a point distant 30 cm from the centre will be
View SolutionA thin spherical shell of metal has a radius of 0.25 m and carries a chaarge of 0.2 mC. The electric indtensity at a point for outside the shell will be
View SolutionThe electric field intensity at a distance 20cm from the centre of a uniformly charged non conducting solid sphere of radius 10cm is E.Then what is the electric field intensity at a distance 5cm from the centre it will be.....
View SolutionA few spherical equipotential surfaces are shown in the figure. The electric field at any point, at a distance x from the centre, is
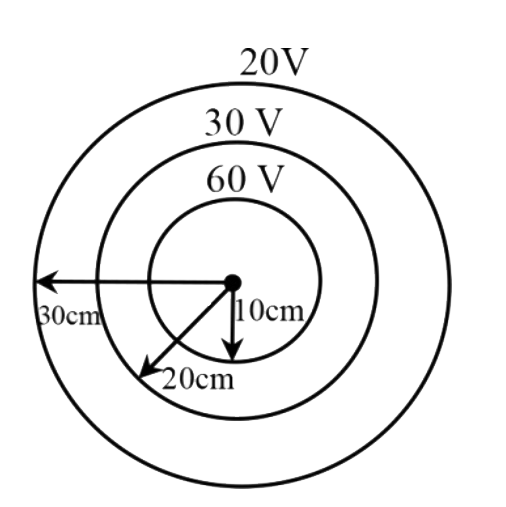 View Solution
View Solution

