Four wires made of same material have different lengths and radii, the wire having more resistance in the following case is
l=100cm,r=1mm
l=50cm,r=2mm
l=100cm,r=12mm
l=50cm,r=12mm
The correct Answer is:C
To determine which wire has the highest resistance among the four given cases, we can use the formula for resistance:
R=ρLA
where:
- R is the resistance,
- ρ is the resistivity of the material (constant for all wires since they are made of the same material),
- L is the length of the wire,
- A is the cross-sectional area of the wire.
The cross-sectional area A for a wire with radius r is given by:
A=πr2
Thus, we can rewrite the resistance formula as:
R=ρLπr2
From this, we can see that resistance R is directly proportional to the length L and inversely proportional to the square of the radius r. Therefore, we can express this relationship as:
R∝Lr2
Now, let's analyze each case provided:
Case A:
- Length LA=100 cm
- Radius rA=110 cm
Calculating RA:
RA∝100(110)2=1001100=10000
Case B:
- Length LB=50 cm
- Radius rB=210 cm
Calculating RB:
RB∝50(210)2=50(210)2=504100=1250
Case C:
- Length LC=100 cm
- Radius rC=120 cm
Calculating RC:
RC∝100(120)2=1001400=40000
Case D:
- Length LD=50 cm
- Radius rD=120 cm
Calculating RD:
RD∝50(120)2=501400=20000
Summary of Results:
- RA∝10000
- RB∝1250
- RC∝40000
- RD∝20000
From the calculations, we can see that:
RC>RD>RA>RB
Conclusion:
The wire with the highest resistance is Case C.
---
- R is the resistance,
- ρ is the resistivity of the material (constant for all wires since they are made of the same material),
- L is the length of the wire,
- A is the cross-sectional area of the wire.
- Length LA=100 cm
- Radius rA=110 cm
- Length LB=50 cm
- Radius rB=210 cm
- Length LC=100 cm
- Radius rC=120 cm
- Length LD=50 cm
- Radius rD=120 cm
- RA∝10000
- RB∝1250
- RC∝40000
- RD∝20000
The wire with the highest resistance is Case C.
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - PRACTICE SHEET (EXERCISE - II LEVEL-II (ADVANCED) (More than One correct answer Type Questions))
3 Videos
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - PRACTICE SHEET (EXERCISE - II LEVEL-II (ADVANCED) (Matrix matching Type Questions))
1 Videos
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - PRACTICE SHEET (EXERCISE - I LEVEL-II (ADVANCED) (Integer Type Questions))
3 Videos
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - PROBLEMS (LEVEL-II)
27 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - AAKASH SERIESCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - PRACTICE EXERCISEX
42 Videos
Similar Questions
Two heater wires, made of the same material and having the same length and the same radius, are first connected in series and then in parallel to a constant potential difference. If the rate of heat produced in the two cases are Hs and Hp respectively then HsHp will be
View SolutionTwo conductors made of the same material have lengths L and 2L but have equal resistance. The two are connected in series in a circuit which current is flowing. Which of the following is/are correct?
View Solution_E01_007_Q01.png)
The load versus elongation graph for four wires of the same material and same length is shown in the figure. The hinnest wire is represented by the line
View SolutionTwo wires A and B of same material have radii in the ratio 2:1 and lengths in the ratio 4:1. The ratio of the normal forces required to produce the same change in the lengths of these two wires is
View SolutionTwo wires 'A' and 'B' of the same material have their lengths in the ratio 1:2 and radii in the ratio 2:1 The two wires are connected in parallel across a battery. The ratio of the heat produced in 'A' to the heat produced in 'B' for the same time is
View SolutionIn Fig. the circular and the straight parts of the wire are made of same material but have different diameters. The magnetic field at the centre is zero.
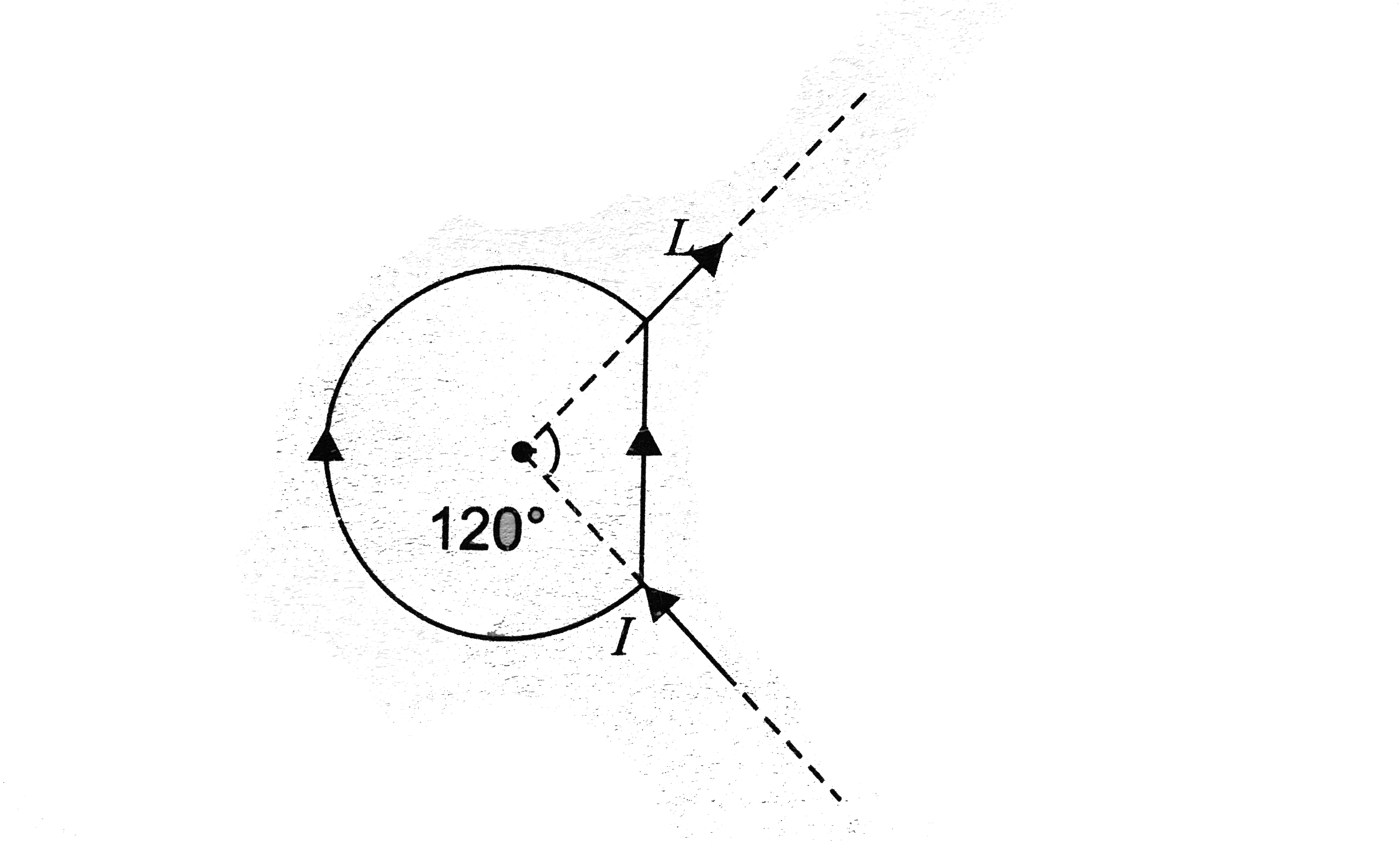
The ratio of the diameters of wires of circular and straight parts is
View SolutionIn Fig. the circular and the straight parts of the wire are made of same material but have different diameters. The magnetic field at the centre is zero.

The ratio of the diameters of wires of circular and straight parts is
View SolutionAn electric current is passed through a circuit containing two wires of the same material connected in parallel. If the lengths and radii of the wire are in the ratio 43 and 23, then the ratio of the currents passing through the wires will be
View SolutionTwo heating coils, one of fine wire and the other of thick wire of the same material and of the same length are connected in series and in parallel. Which of the following statement is correct ?
View SolutionTwo wires A and B are made of same material. The wire A has a length l and diameter r while the wire B has a length 2l and diameter r/2. If the two wires are stretched by the same force the elongation in A divided by the elongation in B is
View Solution
