An electric current is passed through a circuit containing two wires of the same material connected in parallel. If the lengths and radii of the wire are in the ratio and , then the ratio of the currents passing through the wires will be
3
To solve the problem of finding the ratio of currents passing through two wires connected in parallel, we will follow these steps:
Step 1: Understand the relationship between resistance and dimensions
The resistance R of a wire is given by the formula:
R=ρLA
where ρ is the resistivity (which is constant since both wires are made of the same material), L is the length of the wire, and A is the cross-sectional area. The area A can be expressed in terms of the radius r as:
A=πr2
Step 2: Set up the ratios for lengths and radii
Given that the lengths of the wires are in the ratio:
L1L2=43
and the radii are in the ratio:
r1r2=23
Step 3: Calculate the areas
Using the radius to find the areas:
A1=πr21andA2=πr22
Thus, the ratio of the areas is:
A1A2=πr21πr22=r21r22=(23)2=49
Step 4: Set up the resistance ratio
Now we can find the ratio of the resistances R1 and R2:
R1R2=L1L2⋅A2A1=4/34/9=43⋅94=93=3
Thus, we have:
R1R2=3orR1=3R2
Step 5: Use Ohm's law for parallel circuits
In a parallel circuit, the voltage across each wire is the same. According to Ohm's law, the current through each wire can be expressed as:
I=VR
Thus, the ratio of the currents I1 and I2 is given by:
I1I2=R2R1
Substituting R1=3R2:
I1I2=R23R2=13
Final Answer
The ratio of the currents passing through the wires is:
I1:I2=1:3
The resistance R of a wire is given by the formula:
R=ρLA
where ρ is the resistivity (which is constant since both wires are made of the same material), L is the length of the wire, and A is the cross-sectional area. The area A can be expressed in terms of the radius r as:
A=πr2
Given that the lengths of the wires are in the ratio:
L1L2=43
and the radii are in the ratio:
r1r2=23
Using the radius to find the areas:
A1=πr21andA2=πr22
Thus, the ratio of the areas is:
A1A2=πr21πr22=r21r22=(23)2=49
Now we can find the ratio of the resistances R1 and R2:
R1R2=L1L2⋅A2A1=4/34/9=43⋅94=93=3
Thus, we have:
R1R2=3orR1=3R2
In a parallel circuit, the voltage across each wire is the same. According to Ohm's law, the current through each wire can be expressed as:
I=VR
Thus, the ratio of the currents I1 and I2 is given by:
I1I2=R2R1
Substituting R1=3R2:
I1I2=R23R2=13
The ratio of the currents passing through the wires is:
I1:I2=1:3
Topper's Solved these Questions
- DC CIRCUITBOOK - VMC MODULES ENGLISHCHAPTER - DC CIRCUITEXERCISE - LEVEL-1 DAILY TUTORIAL SHEET-2
15 Videos
- DC CIRCUITBOOK - VMC MODULES ENGLISHCHAPTER - DC CIRCUITEXERCISE - LEVEL-1 DAILY TUTORIAL SHEET-3
15 Videos
- DC CIRCUITBOOK - VMC MODULES ENGLISHCHAPTER - DC CIRCUITEXERCISE - LEVEL-0 LONG ANSWER TYPE
3 Videos
- CURRENT ELECTRICITYBOOK - VMC MODULES ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - IN-CHAPTER EXERCISE-F
10 Videos
- DYNAMICS OF A PARTICLEBOOK - VMC MODULES ENGLISHCHAPTER - DYNAMICS OF A PARTICLEEXERCISE - JEE Advance (Archive) Level - II (SINGLE OPTION CORRECT TYPE )
31 Videos
Similar Questions
Knowledge Check
An electric of 5A is passing through a circuit contaning three arrengement in parallel if the length and radius of the wires are in the ratio 2:3:4and3:4:5 then the ratio of current passing through wires should be
A3:6:10B4:9:16C9:16:25D54:64:75
Will current flow more easily through a thick wire or a thin wire of the same material when connected to the same source ? Why ?
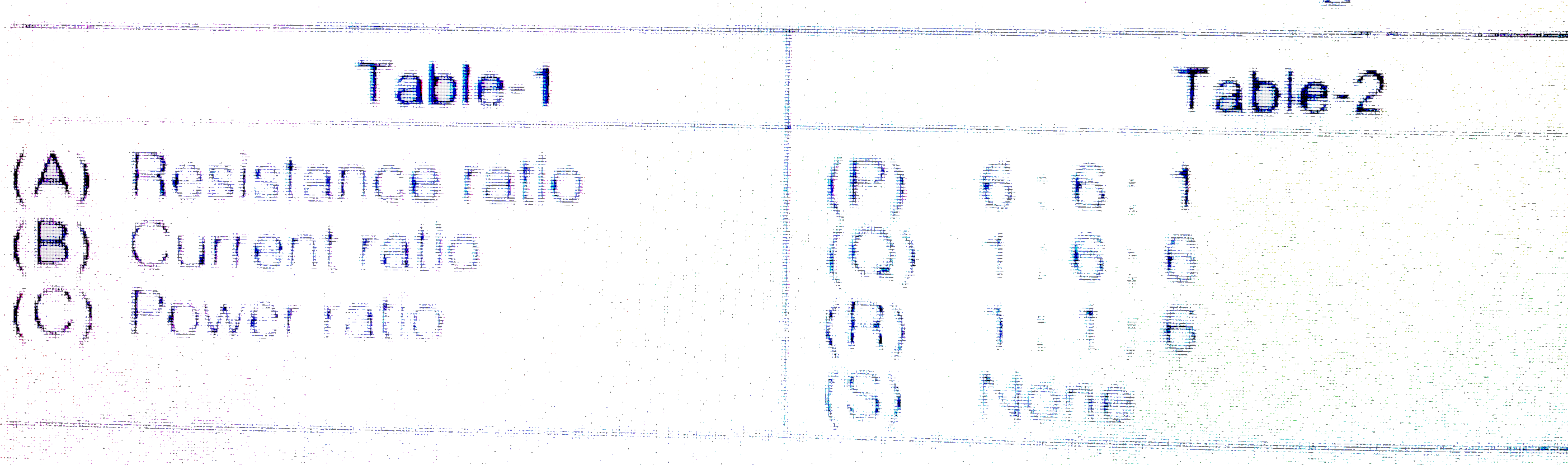
View SolutionThree wires of same material are connected in parallel to a source of emf. The length ratio of the wires is 1:2:3 and the ratio of their area of cross section is 2:4:1 then match the following
View SolutionTwo wires of the same material and same mass are stretched by the same force. Their lengths are in the ratio 2:3. Their elongation are in the ratio
View SolutionTwo wires of the same material and length are stretched by the same force. Their masses are in the ratio 3:2. Their elongations are in the ratio
View SolutionAn electric current of 2.0 A passes through a wire of resistance 25Ω. How much heat will be developed in 1 minute?
View SolutionAn electric current of 2.0 A passes through a wire of resistance 25Ω. How much heat will be developed in 1 minute?
View SolutionTwo wires of the same material have lengths in the ratio 1:2 and their radii are in the ratio 1:√2 If they are stretched by applying equal forces, the increase in their lengths will be in the ratio
View Solution
