What will be input of A and B for the Boolean expression ¯¯¯¯¯¯¯¯¯¯¯¯¯¯(A+B).¯¯¯¯¯¯¯¯¯¯¯(A.B)=1?
0,0
0,1
1,0
1,1
The correct Answer is:D
Step by step video solution for What will be input of A and B for the Boolean expression bar((A+B)).bar((A.B))=1? by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SEMICONDUCTOR DEVICESBOOK - AAKASH SERIESCHAPTER - SEMICONDUCTOR DEVICESEXERCISE - PRACTICE EXERCISE (ENERGY BANDS AND CLASSIFICATION OF SOLIDS )
31 Videos
- SEMICONDUCTOR DEVICESBOOK - AAKASH SERIESCHAPTER - SEMICONDUCTOR DEVICESEXERCISE - PRACTICE EXERCISE (TRANSISTORS )
8 Videos
- SEMICONDUCTOR DEVICESBOOK - AAKASH SERIESCHAPTER - SEMICONDUCTOR DEVICESEXERCISE - EXERCISE -II (TRANSISTORS )
9 Videos
- RAY OPTICSBOOK - AAKASH SERIESCHAPTER - RAY OPTICSEXERCISE - PROBLEMS ( LEVEL-II)
60 Videos
- UNITS AND MEASUREMENTBOOK - AAKASH SERIESCHAPTER - UNITS AND MEASUREMENTEXERCISE - PRACTICE EXERCISE
45 Videos
Similar Questions
Knowledge Check
What will be the input of A and B for the Boolean expression ¯¯¯¯¯¯¯¯¯¯¯¯¯¯(A+B).¯¯¯¯¯¯¯¯¯¯¯(A.B)=1?
A(0,0)B(0,1)C(1,0)D(1,1)Point A, B, and C all lie on a line. Point D is midpoint of ¯¯¯¯¯¯AB and E is the midpoint of BC,¯¯¯¯¯¯AB=4and¯¯¯¯¯¯BC=10. Which of the following could be the length of ¯¯¯¯¯¯AE?
A1B2C3D4
An AND gate is following by a NOT gate in series. With two inputs A & B, the Boolean expression for the out put Y will be :
View SolutionIf A = 1 and B = 0, then in terms of Boolean algebra , A+¯¯¯B= .
View SolutionThe diagram shows a coil wound around a U shape soft iron bar AB.
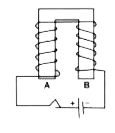
What will be the polarities at A and B if the direction of current is reversed in the circuit ?
View SolutionName the gate , which represents the Boolean expression y = A. B for inputs A, B
View SolutionIf A, B, C, be the centres of three co-axial circles and t1,t2,t3 be the lengths of the tangents of them any piont, prove that
¯¯¯¯¯¯BC.t21+¯¯¯¯¯¯CA.t22+¯¯¯¯¯¯AB.t23=0
View SolutionIf A and B are two sets, then A∩(¯¯¯¯¯¯¯¯¯¯A∪B) is equal to :
View Solution
