A solenoid of infinite length consists of a single layer 1000 turns per unit length of a wire carrying a current of 2mA. Calculate the magnetic field on the axis at the middle of the solenoid.
To solve the problem of calculating the magnetic field at the axis of an infinite solenoid, we can follow these steps:
1. Identify the Given Values:
- Number of turns per unit length (n) = 1000 turns/m
- Current (I) = 2 mA = 2 × 10^(-3) A
- Permeability of free space (μ₀) = 4π × 10^(-7) T·m/A
2. Use the Formula for the Magnetic Field in a Solenoid:
The magnetic field (B) inside a long solenoid is given by the formula:
B=μ0⋅n⋅I
3. Substitute the Values into the Formula:
Substitute the known values into the formula:
B=(4π×10−7T⋅m/A)⋅(1000turns/m)⋅(2×10−3A)
4. Calculate the Magnetic Field:
- First, calculate the product of the constants:
B=4π×10−7⋅1000⋅2×10−3
- Simplifying this:
B=4π×10−7⋅2×10−3⋅1000
- The 1000 can be expressed as 103:
B=4π×10−7⋅2×103⋅10−3
- This simplifies to:
B=4π×2×10−7T
- Which equals:
B=8π×10−7T
5. Calculate the Numerical Value:
- Using the approximate value of π≈3.14:
B≈8×3.14×10−7T≈25.12×10−7T
6. Final Answer:
B≈2.512×10−6Tor25.12μT
- Number of turns per unit length (n) = 1000 turns/m
- Current (I) = 2 mA = 2 × 10^(-3) A
- Permeability of free space (μ₀) = 4π × 10^(-7) T·m/A
The magnetic field (B) inside a long solenoid is given by the formula:
B=μ0⋅n⋅I
Substitute the known values into the formula:
B=(4π×10−7T⋅m/A)⋅(1000turns/m)⋅(2×10−3A)
- First, calculate the product of the constants:
B=4π×10−7⋅1000⋅2×10−3
- Simplifying this:
B=4π×10−7⋅2×10−3⋅1000
- The 1000 can be expressed as 103:
B=4π×10−7⋅2×103⋅10−3
- This simplifies to:
B=4π×2×10−7T
- Which equals:
B=8π×10−7T
- Using the approximate value of π≈3.14:
B≈8×3.14×10−7T≈25.12×10−7T
B≈2.512×10−6Tor25.12μT
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETICSEXERCISE - PROBLEMS (LEVEL -II)40videos
ELECTROMAGNETICS
- BOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETICSEXERCISE - ADDITIONAL EXERCISE13videos
ELECTROMAGNETICS
- BOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETICSEXERCISE - EXERCISE (VERY SHORT ANSWER QUESTIONS )19videos
ELECTROMAGNETICS
- BOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - EXERCISE -II22videos
ELECTROMAGNETIC WAVES
- BOOK - AAKASH SERIESCHAPTER - ELECTROSTATICSEXERCISE - ADDITIONAL PRACTICE EXERCISE (PRACTICE SHEET (ADVANCED) INTEGER TYPE QUESTIONS)5videos
ELECTROSTATICS
Similar Questions
A solenoid of length 0.4m and diameter 0.6m consists of a single layer of 1000 turns of fine wire carrying a current of 5.0×10−3 ampere.The magnetic field strength on the axis at the middle is πN×10−5T, then find value of N.
View SolutionA thin solenoid of length 0.4m and having 500 turns of wire carries a current 1A,then find the magnetic field on the axis inside the solenoid.
View Solution
Knowledge Check
- Question 1 - Select One
A solenoid of length 0.6 m has a radius of 2 cm and is made up of 600 turns If it carries a current of 4 A, then the magnitude of the magnetic field inside the solenoid is
A6.024×10−3TB8.024×10−3TC5.024×10−3TD7.024×10−3T
A solenoid of 2m long & 3cm diameter has 5 layers of winding of 500 turns per metre length in each layer & carries a current of 5A. Find intensity of magnetic field at the centre of the solenoid.
View SolutionA long solenoid has a radius a and number of turns per unit length is n. If it carries a current i, then the magnetic field on its axis is directly proportional to
View SolutionA wire of infinite length bent as shown in figure carries current l. What is the magnetic field at the point P ?
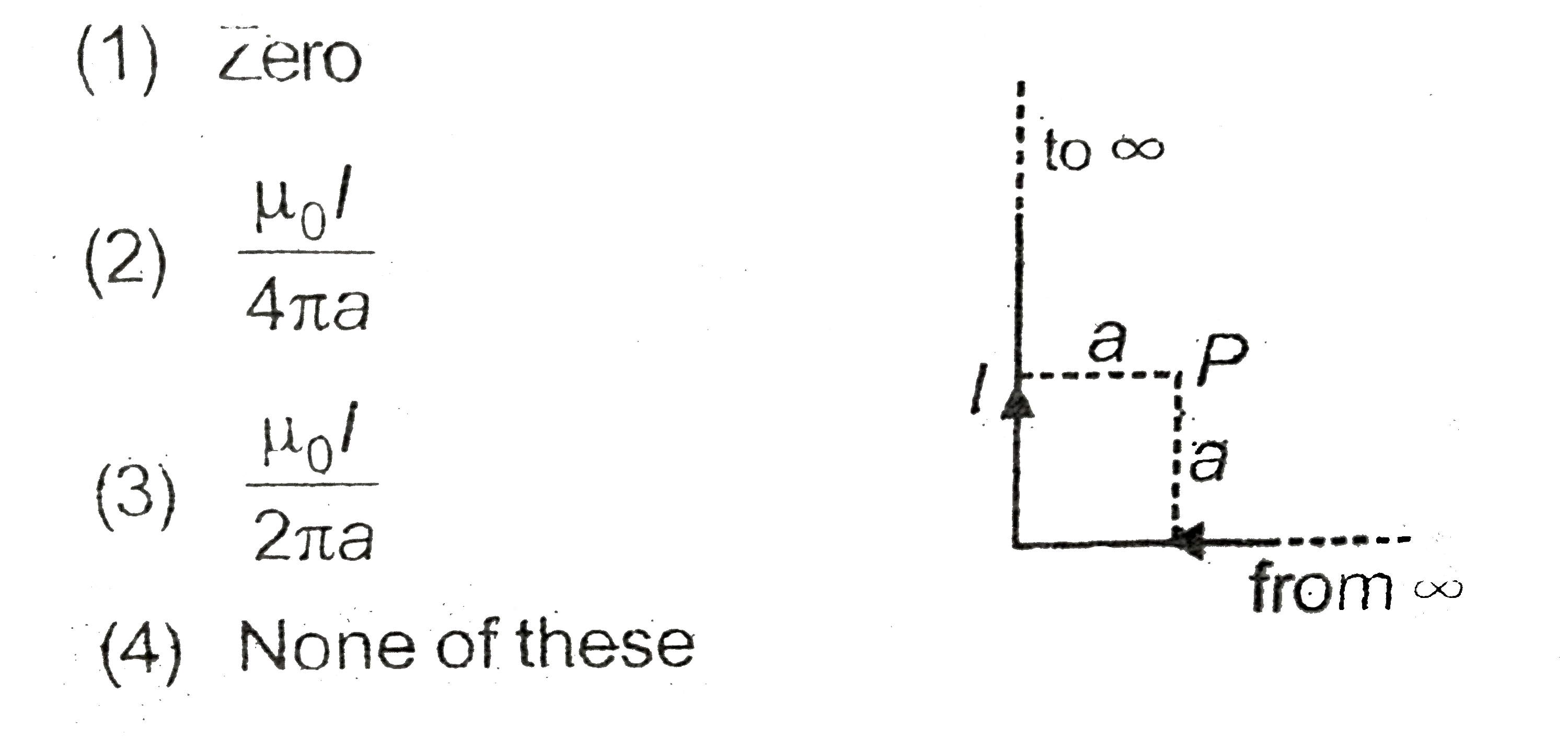 View Solution
View SolutionA long solenoid of 50 cm length having 100 turns carries a current of 2.5A. The magnetic field at centre of solenoid is:
View SolutionA long solenoid has 800 turns per metre length of solenoid A currentof 1.6 A flow through it. The magnetic induction at the end of the solenoid on its axis is
View SolutionA solenoid of length 8cm has 500 turns /m in it. If radius of coil is 3cm and if it is carrying a current of 2A, find the magnetic induction at a point 4cm from the end on the axis of the solenoid
View SolutionA solenoid of length 0⋅5m has a radius of 1cm and is made up of 500 turns. It carries a current of 5A. What is the magnitude of the magnetic field inside the solenoid?
View Solution

