Show that tiem of ascent of a vertically projected body is equal to time of descent.
To show that the time of ascent of a vertically projected body is equal to the time of descent, we can follow these steps:
1. Define the Problem:
We are projecting a body vertically upwards with an initial velocity u. We need to find the time taken to reach the maximum height (time of ascent, ta) and the time taken to fall back to the ground from that height (time of descent, td).
2. Analyze the Ascent:
- When the body reaches its maximum height, its final velocity v becomes 0.
- We can use the first equation of motion:
v=u+at
Here, a=−g (acceleration due to gravity acting downwards), v=0, and t=ta.
- Substituting these values gives:
0=u−gta
- Rearranging this equation, we find:
gta=u⟹ta=ug
3. Analyze the Descent:
- When the body falls back to the ground, it starts from rest at the maximum height, so its initial velocity u=0 at the maximum height and it will reach the ground with a final velocity v=u (the same as the initial velocity when projected).
- Again, we use the first equation of motion:
v=u+at
Here, u=0, a=g, and t=td.
- Substituting these values gives:
u=0+gtd
- Rearranging this equation, we find:
gtd=u⟹td=ug
4. Equate the Times:
- From the equations derived, we have:
ta=ugandtd=ug
- Therefore, we can conclude that:
ta=td
- This shows that the time of ascent is equal to the time of descent.
Conclusion:
Thus, we have proved that the time of ascent of a vertically projected body is equal to the time of descent.
We are projecting a body vertically upwards with an initial velocity u. We need to find the time taken to reach the maximum height (time of ascent, ta) and the time taken to fall back to the ground from that height (time of descent, td).
- When the body reaches its maximum height, its final velocity v becomes 0.
- We can use the first equation of motion:
v=u+at
Here, a=−g (acceleration due to gravity acting downwards), v=0, and t=ta.
- Substituting these values gives:
0=u−gta
- Rearranging this equation, we find:
gta=u⟹ta=ug
- When the body falls back to the ground, it starts from rest at the maximum height, so its initial velocity u=0 at the maximum height and it will reach the ground with a final velocity v=u (the same as the initial velocity when projected).
- Again, we use the first equation of motion:
v=u+at
Here, u=0, a=g, and t=td.
- Substituting these values gives:
u=0+gtd
- Rearranging this equation, we find:
gtd=u⟹td=ug
- From the equations derived, we have:
ta=ugandtd=ug
- Therefore, we can conclude that:
ta=td
- This shows that the time of ascent is equal to the time of descent.
Thus, we have proved that the time of ascent of a vertically projected body is equal to the time of descent.
Similar Questions
A : In the presence of air resistance, if the ball is thrown vertically upwards then time of ascent is less than the time of descent.
R : Force due to air friction always acts opposite to the motion of the body.View SolutionA body is pushed up on a rough inclined plane making an angle 30∘ to the horizontal. If its time of ascent on the plane is half the time of its descent, find coefficient of friction between the body and the incined plane.
View SolutionShow that the work done to rotate a body is equal to the change in rotational kinetic energy,
View SolutionRead the following statements and choose the correct answer.
(a) For a freely falling body the average velocity is proportional to square root of height of fall.
(b) For a freely falling body the displacements in successive equal time intervals are in the ratio 1:4:9:....
(c) For a vertically projected body the displacement during last second of time of flight changes with velocity of projection.
(d) For a body projected from the top of the tower the displacement of the body is negative when the body crosses the point of projectionView Solution(A) : The time of ascent for a body projected to move up a rough inclined plane is less than the time of descent.
(R ) : The retardation for upward motion is more than the acceleration for down motion on rough inclined plane.View SolutionIn case of an object vertically projected, show that the speed of the object upon reaching the point of projection is equal to speed of projection .
View SolutionShow that if a body be projected vertically upward from the surface of the earth so as to reach a height nR above the surface
(i) the increase in its potential energy is (nn+1)mgR,
(ii) the velocity with it must be projected is √2ngRn+1, where r is the radius of the earth and m the mass of body.View SolutionA body of mass 5×10−3 kg is launched upon a rough inclined plane making an angle of 30∘ with the horizontal. Obtain the coefficient of friction between the body and the plane if the time of ascent is half of the time of descent.
View SolutionA particle is thrown upwards from ground. It experiences a constant resistance force which can produce a retardation of 2ms−2. The ratio of time of ascent to time of descent is (g=10ms−2)
View SolutionPQ is a smooth inclined plane whose angle θ can be varied in such a way that point Q remains fixed and P can move on a vertical line PR. A particle slides from rest from point P at different value of θ time for descent from P and Q is noted. The following statement (s) is / are correct about the time of descent :
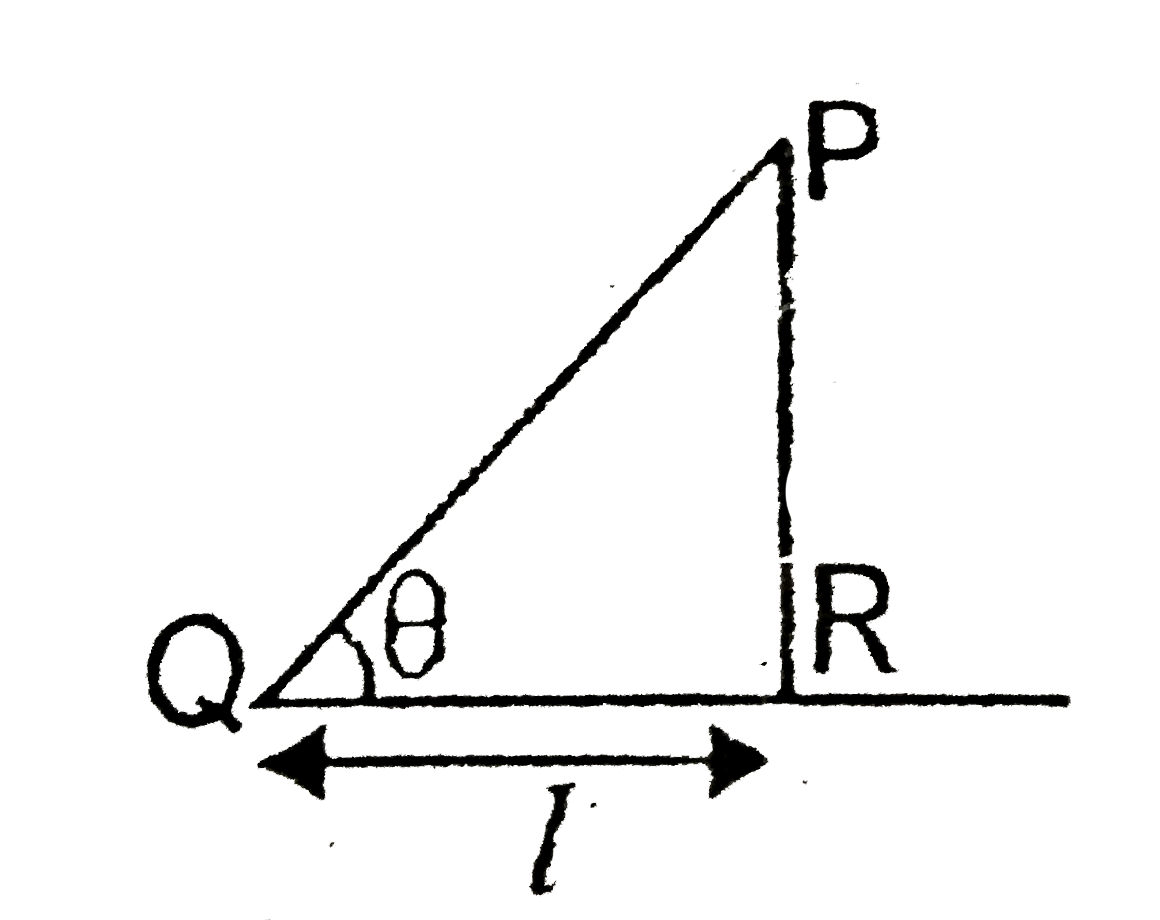
A. the minimum time of descent is 2√l/g
B. the time descent is minimum continuously as θ is increased
C. the time of descent decreases continuously as θ is increased
D. the time of descent first decreases then increases.View Solution

