For the reactionA→B, for which graph between half life (t1/2)and∈itialconcentration(a)oftherectantisgivenbelow<br><imga≤ocr_imageload∈g=lazysrc=https://d10lpgp6xz60nq.cloudfront.net/physics_images/NRA_PHY_CHM_JMA_C07_E01_022_Q01.pngwh=80%mx-autosty≤=background:white;><br>Hencegraphbetween-(d[A])/(dt) and time will be: Solution in Telugu
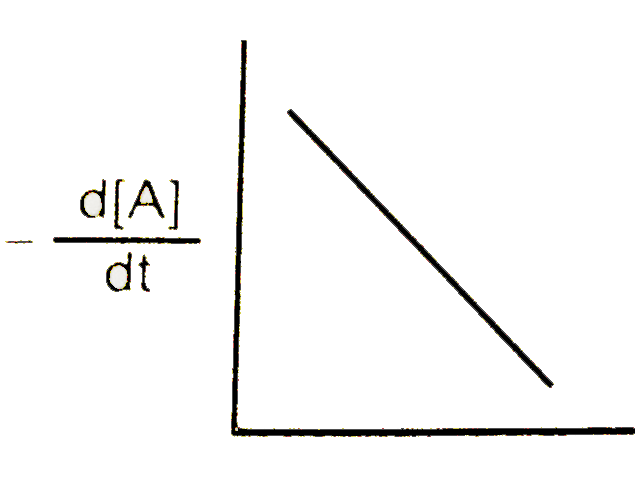
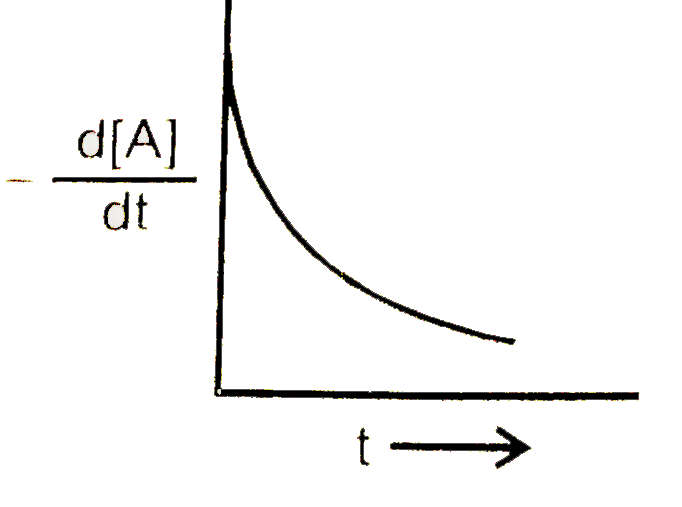
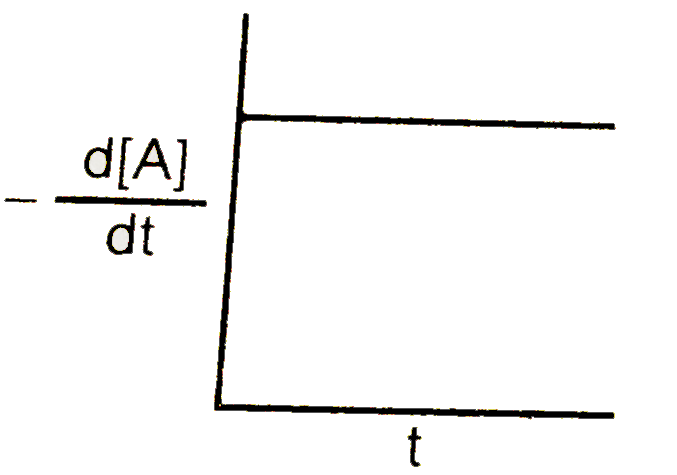
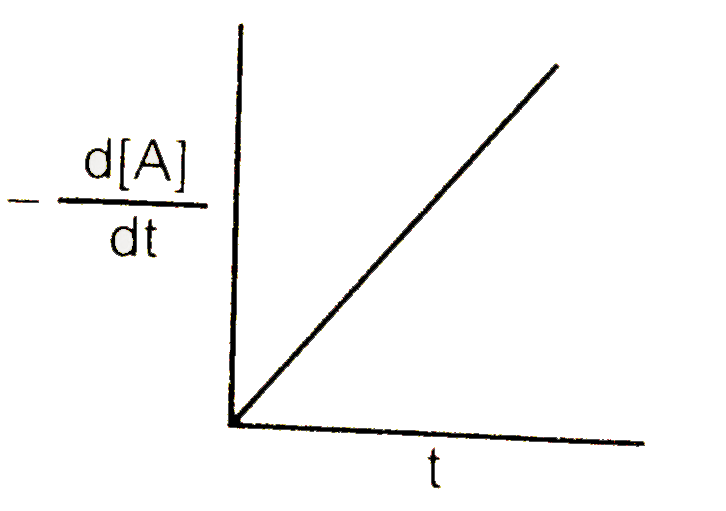
The correct Answer is:C
Step by step video & image solution for For the reactionAtoB, for which graph between half life (t_(1//2)) and initial concentration (a) of the rectant is given below Hence graph between -(d[A])/(dt) and time will be: by Chemistry experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CHEMICAL KINETIC & NUCLEAR CHEMISTRYBOOK - NARENDRA AWASTHICHAPTER - CHEMICAL KINETIC & NUCLEAR CHEMISTRYEXERCISE - Level 1 (Q.3 To Q.32)
2 Videos
- CHEMICAL KINETIC & NUCLEAR CHEMISTRYBOOK - NARENDRA AWASTHICHAPTER - CHEMICAL KINETIC & NUCLEAR CHEMISTRYEXERCISE - Level 1 (Q.33 To Q.62)
3 Videos
- SURFACE CHEMISTRYBOOK - NARENDRA AWASTHICHAPTER - SURFACE CHEMISTRYEXERCISE - Level 3 - Assertion - Reason Type Questions
1 Videos
Similar Questions
The reaction of A2 and B2 follows the equation
A2(g)+B2(g)→2AB(g)
The following data were observed
[A2]0[B2]0Initial rate of appearance of AB(g)(inMs−10.100.102.5×10−40.200.105×10−40.200.2010×10−4
The value of rate constatnt for the above reaction is :
View SolutionHalf-life (t1/2) and completion time (T) of the reaction (A→2B) are if initial concentration A is 1M and k = 0.001M/ min :
View Solutioneach question constain STATEMENT-1(Assertion ) and STATEMENT - 2 (reason). examine the statement carefully and work the correct answer accoridng to the instructions given below :
STATEMENT-1: Dispersed phase particles of colloidal solution cannot pass through ultra -filter paper.
STATEMENT-2: The size of colloidal particles are larger than the size of true solution particles.
View SolutionThe graph between concentration (X) of the Product and time of the reaction A→B is of the type 1. Hence, graph between −d[A]dt and time will be of the type:
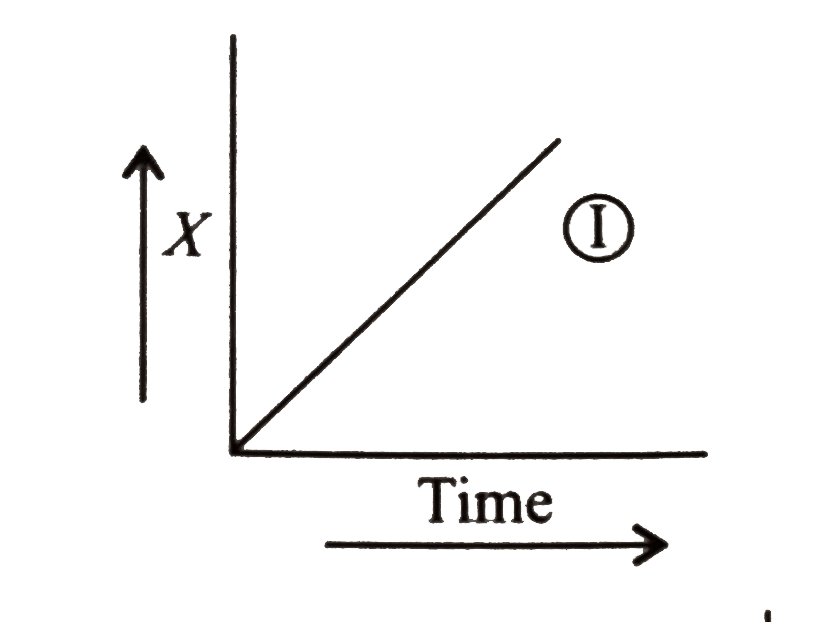
View SolutionFollowing is the graph between log T_(50) and log a ( a = initial concentration) for a given reaction at 27^(@)C . Hence order is
View SolutionFollowing is the graph between logt1/2 and loga (a initial concentration) for a given reaction at 27∘C.

Find the order of reaction.
View SolutionFor which order reaction a straight line is obtained along with x -axis by plotting a graph between half-life (t_(1//2)) and initial concentration 'a'
View SolutionA nucleus A (parent) decays into B (Daughter) with half life T1 and B decays into C with half life T2. Graph is drawn between number of atoms/activity versus time. Select the correct graph (s)
View SolutionFor the reaction AtoB , for which graph between half life (t_(1//2)) and initial concentration (a) of the rectant is given below Hence graph between -(d[A])/(dt) and time will be:
View SolutionGraph between concentration of the product and time of the reaction A rarr B is of the type Hence, graph -d[A]//dt and time will be of the type:
View SolutionFollowing is the graph between log T_(50) and log a (a = initial concentration) for a given reaction at 27^(@)C . Hence, order is
View SolutionFor which order reaction a straight line is obtained along with x-axis by plotting a graph between half-life (t1/2) and initial concentration 'a'
View SolutionWhich of the following graphs formed plotted between t_(1//2) and initial concentration (a) represents a zero order reaction ?
View SolutionIn a first order reaction the graph between t_(1//2) and concentration is.
View SolutionGive relation between half reaction time (t1/2) and initial concentration of reactant for (n−1) order reaction
View SolutionFor the reactionA→B, for which graph between half life (t1/2)and∈itialconcentration(a)oftherectantisgivenbelow<br><imga≤ocr_imageload∈g=lazysrc=https://d10lpgp6xz60nq.cloudfront.net/physics_images/NRA_PHY_CHM_JMA_C07_E01_022_Q01.pngwh=80%mx-autosty≤=background:white;><br>Hencegraphbetween-(d[A])/(dt) and time will be:
View Solution
